Published online by Cambridge University Press: 03 November 2016
The problem of finding rational integral numbers which could be made the sides of right triangles, is said by Proclus to have been solved by Pythagoras, by a method which is equivalent to the use of the formula,
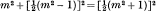
where m is any odd integer.
Heath (1) quotes Proclus’ statement as follows:
“The method starts from odd numbers. For it makes the odd number the smaller of the sides about the right angle; then it takes the square of it, subtracts unity, and makes half the difference the greater of the sides about the right angle; lastly it adds unity to this and so forms the remaining side the hypotenuse.”