Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-28T07:23:50.435Z Has data issue: false hasContentIssue false
  • English
  • Français

A lower bound for the prime counting function

Published online by Cambridge University Press:  23 January 2015

Daniel Shiu  and
Peter Shiu
Daniel Shiu
Affiliation:
16 St Luke's Place, Cheltenham, GL53 7HP
Peter Shiu
Affiliation:
353 Fulwood Road, Sheffield, S10 3BQ e-mail:, [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

Let π (x) count the primes p ≤ x, where x is a large real number. Euclid proved that there are infinitely many primes, so that π (x) → ∞ as x → ∞; in fact his famous argument ([1: Section 2.2]) can be used to show that

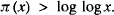
There was no further progress on the problem of the distribution of primes until Euler developed various tools for the purpose; in particular he proved in 1737 [1: Theorem 427] that

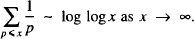
Type
Articles
Information
The Mathematical Gazette , Volume 95 , Issue 534 , November 2011 , pp. 433 - 436
Copyright
Copyright © The Mathematical Association 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Hardy, G. H. and Wright, E. M., An introduction to the theory of numbers (4th edn.), Oxford University Press (1960).Google Scholar