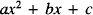Everyone knows that the condition for the quadratic to have two equal roots, i.e. to have a repeated root, is that its discriminant should be zero. We should remark, at the outset, that we are concerned only with ordinary polynomials whose coefficients are complex numbers. Indeed, little is lost if a reader assumes that all our polynomials are real, i.e. have real numbers for all their coefficients, though their complex roots must be considered as well as their real ones.