Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-20T01:07:45.658Z Has data issue: false hasContentIssue false
  • English
  • Français

92.55 Reconstructing heronian triangles

Published online by Cambridge University Press:  01 August 2016

Paul Stephenson
Paul Stephenson*
Affiliation:
The Magic Mathworks Travelling Circus, Old Coach House, Penypwllau, Holywell CH8 8HB, e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

An abstract is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
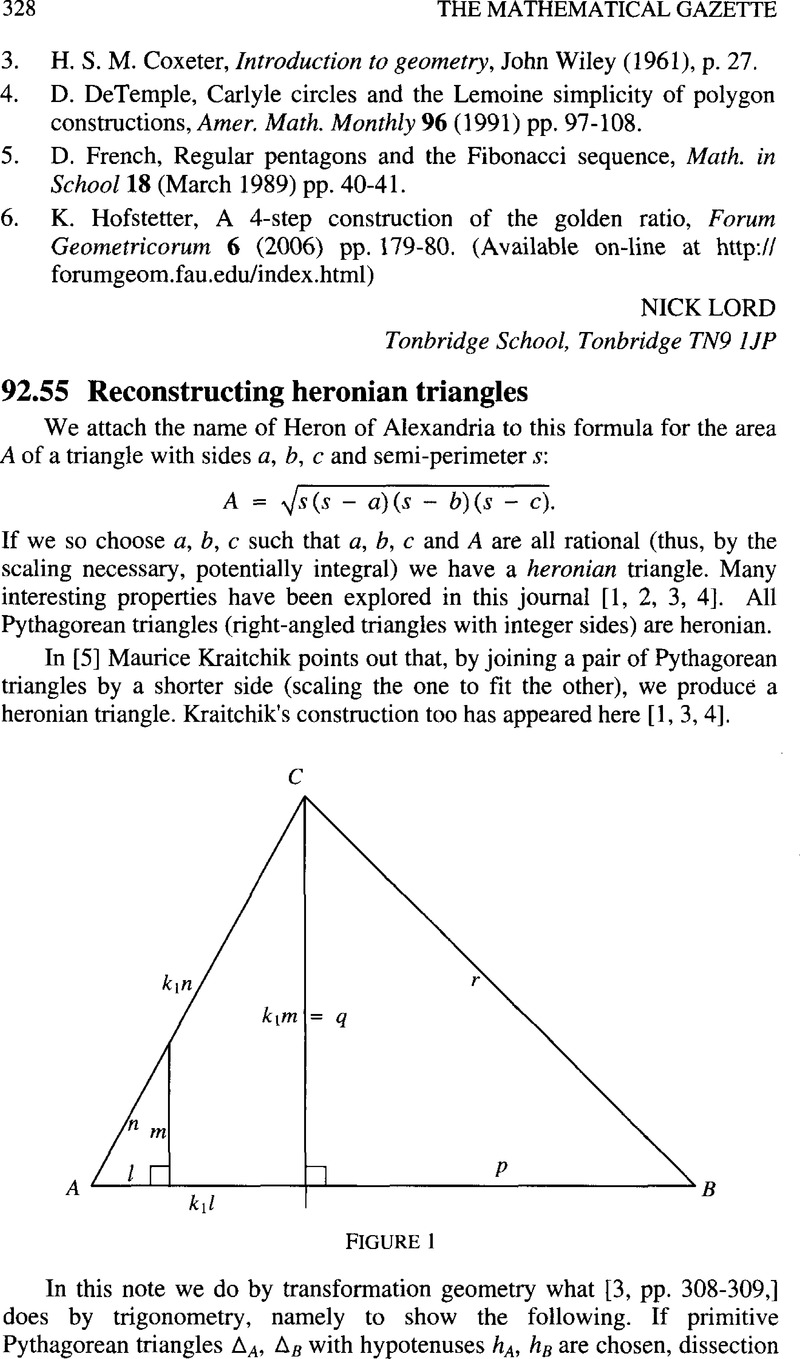
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Notes
Information
The Mathematical Gazette , Volume 92 , Issue 524 , July 2008 , pp. 328 - 331
Copyright
Copyright © The Mathematical Association 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Wain, G. & Wynne-Willson, W., 13, 14, 15: an investigation, Math. Gaz. 71 (March 1987) pp. 32–37.Google Scholar
2. McLean, K. Robin, Heronian triangles are almost everywhere, Math. Gaz. 72 (March 1988) pp. 49–51.Google Scholar
3. Ellis, L. E., Hero triangles, Math. Gaz. 86 (July 2002) pp. 307–310.Google Scholar
4. Bradley, C. J., Heron triangles and touching circles, Math. Gaz. 87 (March 2003) pp. 36–41.Google Scholar
5. Kraitchik, M., Mathematical Recreations, W. W. Norton (1942) pp. 104–108.Google Scholar
6. Jackson, M., Complex numbers and Pythagorean triples, Math. Gaz. 71 (March 1987) p. 127.Google Scholar
7. Dantzig, T., Number (4th edn.), The Macmillan Company, New York (1954) pp. 288–289.Google Scholar