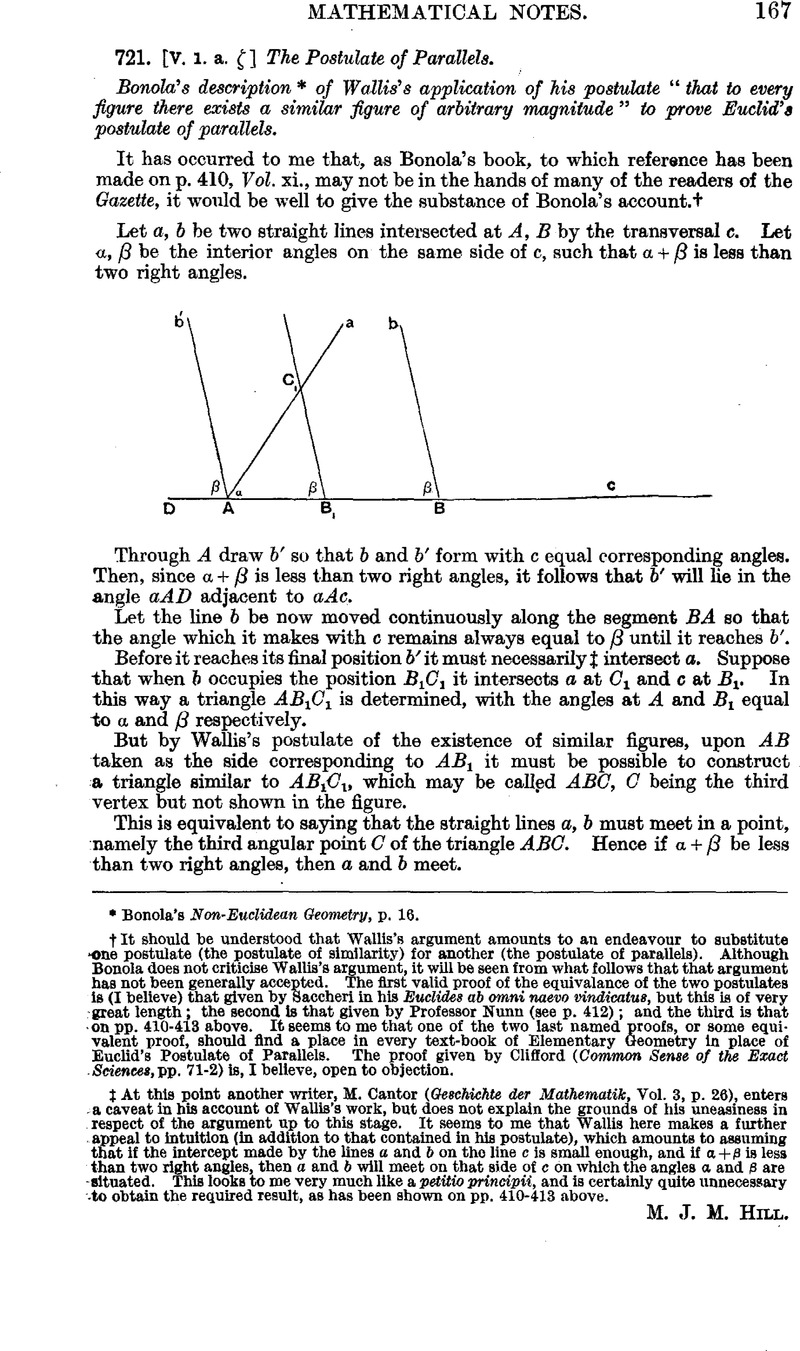
Published online by Cambridge University Press: 03 November 2016
page 167 note * Bonola’s Non-Euclidean Geometry, p. 16.
page 167 note † It should be understood that Wallis’s argument amounts to an endeavour to substitute one postulate (the postulate oí similarity) for another (the postulate of parallels). Although Bonola does not criticise Wallis’s argument, it will be seen from what follows that that argument has not been generally accepted. The first valid proof of the equivalance of the two postulates is (I believe) that given by Saccheri in his Euclides ab omni naevo vindicatue, but this is of very great length ; the second is that given by Professor Nunn (see p. 412) ; and the third is that on pp. 410-413 above. It seems to me that one of the two last named proofs, or some equivalent proof, should find a place in every text-book of Elementary Geometry in place of Euclid’s Postulate of Parallels. The proof given by Clifford (Common Sense of the Exact Sciences, pp. 71-2) is, I believe, open to objection.
page 167 note ‡ At this point another writer, M. Cantor (Geschichte der Mathematih, Vol. 3, p. 26), enters a caveat in his account of Wallis’s work, but does not explain the grounds of his uneasiness in respect of the argument up to this stage. It seems to me that Wallis here makes a further appeal to intuition (in addition to that contained in his postulate), which amounts to assuming that if the intercept made by the lines a and b on tho line c is small enough, and if a+3 is less than two right angles, then a and 6 will meet on that side of c on which the angles a and S are situated. This looks to me very much like a petitio principii, and is certainly quite unnecessary to obtain the required result, as has been shown on pp. 410-413 above.