Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-25T17:03:29.829Z Has data issue: false hasContentIssue false
  • English
  • Français

Single-class genera of positive integral lattices

Part of: Forms and linear algebraic groups

Published online by Cambridge University Press:  01 August 2013

David Lorch  and
Markus Kirschmer
David Lorch
Affiliation:
Lehrstuhl D für Mathematik,RWTH Aachen University,Templergraben 64,D-52062 Aachen,Germany email [email protected]@math.rwth-aachen.de
Markus Kirschmer
Affiliation:
Lehrstuhl D für Mathematik,RWTH Aachen University,Templergraben 64,D-52062 Aachen,Germany email [email protected]@math.rwth-aachen.de

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

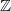We give an enumeration of all positive definite primitive $ \mathbb{Z} $-lattices in dimension 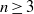$n\geq 3$ whose genus consists of a single isometry class. This is achieved by using bounds obtained from the Smith–Minkowski–Siegel mass formula to computationally construct the square-free determinant lattices with this property, and then repeatedly calculating pre-images under a mapping first introduced by G. L. Watson.

We hereby complete the classification of single-class genera in dimensions 4 and 5 and correct some mistakes in Watson’s classifications in other dimensions. A list of all single-class primitive $ \mathbb{Z} $-lattices has been compiled and incorporated into the Catalogue of Lattices.

MSC classification

Secondary: 11E12: Quadratic forms over global rings and fields 11E41: Class numbers of quadratic and Hermitian forms
Type
Research Article
Information
LMS Journal of Computation and Mathematics , Volume 16 , October 2013 , pp. 172 - 186
Copyright
© The Author(s) 2013 

References

Bosma, W., Cannon, J. and Playoust, C., ‘The Magma algebra system. I. The user language’, J. Symbolic Comput. 24 (1997) no. 3–4, 235–265; Computational algebra and number theory (London, 1993).CrossRefGoogle Scholar
Conway, J. H., ‘On the classification of integral quadratic forms’, Sphere packings, lattices, and groups, 3rd edn (Springer, Berlin, 1999) 352–384.CrossRefGoogle Scholar
Conway, J. H. and Sloane, N., ‘Low-dimensional lattices. iv. The mass formula’, Proc. R. Soc. Lond. 419 (1988) 259–286.Google Scholar
Hanke, J., ‘Enumerating maximal definite quadratic forms of bounded class number over Z in $n\gt = 3$ variables’, Preprint, 2011, arXiv:1110.1876 [math.NT].Google Scholar
Jagy, W. C., Kaplansky, I. and Schiemann, A., ‘There are 913 regular ternary forms’, Mathematika 44 (1997) no. 2, 332–341.CrossRefGoogle Scholar
Nebe, G. and Sloane, N., ‘Catalogue of lattices’, http://www.math.rwth-aachen.de/~Gabriele.Nebe/LATTICES.Google Scholar
Timothy O’Meara, O., Introduction to quadratic forms (Springer, Berlin, 1973).CrossRefGoogle Scholar
Voight, J., ‘Quadratic forms that represent almost the same primes’, Math. Comput. 76 (2007) 1589–1617.CrossRefGoogle Scholar
Watson, G. L., ‘Transformations of a quadratic form which do not increase the class-number’, Proc. Lond. Math. Soc. (3) 12 (1962) 577–587.CrossRefGoogle Scholar
Watson, G. L., ‘The class-number of a positive quadratic form’, Proc. London Math. Soc. (3) 13 (1963) 549–576.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive ternary quadratic forms’, Mathematika 19 (1972) 96–104.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive quadratic forms in at least five variables’, Acta Arith. 26 (1974/75) no. 3, 309–327.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive quaternary quadratic forms’, Acta Arith. 24 (1975) no. 1, 461–475.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive ternary quadratic forms. II’, Mathematika 22 (1975) no. 1, 1–11.CrossRefGoogle Scholar
Watson, G. L., ‘Transformations of a quadratic form which do not increase the class-number (II)’, Acta Arith. 27 (1975) 171–189.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive quadratic forms in nine and ten variables’, Mathematika 25 (1978) no. 1, 57–67.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive quadratic forms in eight variables’, J. London Math. Soc. (2) 26 (1982) no. 2, 227–244.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive quadratic forms in seven variables’, Proc. London Math. Soc. (3) 48 (1984) no. 1, 175–192.CrossRefGoogle Scholar
Watson, G. L., ‘One-class genera of positive quadratic forms in six variables’, unfinished draft, 1988.Google Scholar