Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-23T03:10:50.444Z Has data issue: false hasContentIssue false
  • English
  • Français

Seven new champion linear codes

Part of: Polytopes and polyhedra Special varieties Arithmetic problems. Diophantine geometry

Published online by Cambridge University Press:  01 January 2013

Gavin Brown  and
Alexander M. Kasprzyk
Gavin Brown
Affiliation:
Department of Mathematical Sciences,Loughborough University,Loughborough, LE11 3TU,United Kingdom email [email protected]
Alexander M. Kasprzyk
Affiliation:
Department of Mathematics,Imperial College London,London, SW7 2AZ,United Kingdom email [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

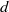We exhibit seven linear codes exceeding the current best known minimum distance $d$ for their dimension $k$ and block length $n$. Each code is defined over 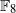${ \mathbb{F} }_{8} $, and their invariants 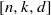$[n, k, d] $ are given by $[49, 13, 27] $, 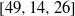$[49, 14, 26] $, $[49, 16, 24] $, $[49, 17, 23] $, 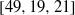$[49, 19, 21] $, 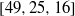$[49, 25, 16] $ and $[49, 26, 15] $. Our method includes an exhaustive search of all monomial evaluation codes generated by points in the 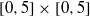$[0, 5] \times [0, 5] $ lattice square.

MSC classification

Secondary: 14G50: Applications to coding theory and cryptography 52B20: Lattice polytopes (including relations with commutative algebra and algebraic geometry) 14M25: Toric varieties, Newton polyhedra
Type
Research Article
Information
LMS Journal of Computation and Mathematics , Volume 16 , October 2013 , pp. 109 - 117
Copyright
© The Author(s) 2013 

References

Amaya, J. E., Harry, A. J. and Vega, B. M., ‘A systematic census of generalized toric codes over ${ \mathbb{F} }_{4} $ , ${ \mathbb{F} }_{5} $ and ${ \mathbb{F} }_{16} $ ’, Technical report, MSRI-UP, July 2009, http://www.msri.org/web/msri/scientific/workshops/show/-/event/Wm491.Google Scholar
Bosma, W., Cannon, J. and Playoust, C., ‘The Magma algebra system. I. The user language’, J. Symbolic Comput. 24 (1997) no. 3–4, 235–265.Google Scholar
Brown, G., Buczyński, J. and Kasprzyk, A. M., ‘Convex polytopes and polyhedra’, Handbook of Magma Functions, Edition 2.17, 2011, http://magma.maths.usyd.edu.au/.Google Scholar
Brown, G. and Kasprzyk, A. M., 2005–2013, ‘The graded ring database’, http://grdb.lboro.ac.uk/.Google Scholar
Brown, G. and Kasprzyk, A. M., ‘Magma routines for computing sets of lattice points up to affine equivalence’, 2012, http://grdb.lboro.ac.uk/files/toriccodes/distinctpoints.tar.gz.Google Scholar
Brown, G. and Kasprzyk, A. M., ‘Small polygons and toric codes’, J. Symbolic Comput. 51 (2013) 55–62.Google Scholar
Carbonara, A., Murillo, J. P. and Ortiz, A., ‘A census of two dimensional toric codes over Galois fields of sizes 7, 8 and 9’, Tech. report, MSRI-UP, July 2009, http://www.msri.org/web/msri/scientific/workshops/show/-/event/Wm491.Google Scholar
Guerrini, E., Bellini, E. and Sala, M., ‘Some bounds on the size of codes’, Preprint, 2012, arXiv:1206.6006v2 [cs.IT].Google Scholar
Grassl, M., ‘Bounds on the minimum distance of linear codes and quantum codes’, 2012, http://www.codetables.de/.Google Scholar
Hansen, J. P., ‘Toric surfaces and error-correcting codes’, Coding theory, cryptography and related areas Guanajuato, 1998 (Springer, Berlin, 2000) 132–142.CrossRefGoogle Scholar
Little, J. B., ‘Remarks on generalized toric codes’, Preprint, 2011, arXiv:1107.4530v2 [cs.IT].Google Scholar
Little, J. and Schwarz, R., ‘On toric codes and multivariate Vandermonde matrices’, Appl. Algebra Engrg. Comm. Comput. 18 (2007) no. 4, 349–367.CrossRefGoogle Scholar
Ruano, D., ‘On the structure of generalized toric codes’, J. Symbolic Comput. 44 (2009) no. 5, 499–506.CrossRefGoogle Scholar