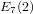Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Burness, Timothy C.
2018.
On base sizes for almost simple primitive groups.
Journal of Algebra,
Vol. 516,
Issue. ,
p.
38.
Burness, Timothy C.
and
Thomas, Adam R.
2018.
On the involution fixity of exceptional groups of Lie type.
International Journal of Algebra and Computation,
Vol. 28,
Issue. 03,
p.
411.
Kelsey, Veronica
and
Rowley, Peter
2020.
A note on involution centralizers in black box groups.
Journal of Group Theory,
Vol. 23,
Issue. 2,
p.
287.
Burness, Timothy C.
and
Thomas, Adam R.
2021.
A note on extremely primitive affine groups.
Archiv der Mathematik,
Vol. 116,
Issue. 2,
p.
141.
Burness, Timothy C
and
Thomas, Adam R
2022.
The Classification of Extremely Primitive Groups.
International Mathematics Research Notices,
Vol. 2022,
Issue. 13,
p.
10148.
Gill, Nick
Liebeck, Martin W.
and
Spiga, Pablo
2022.
Cherlin’s Conjecture for Finite Primitive Binary Permutation Groups.
Vol. 2302,
Issue. ,
p.
71.
Pierro, Emilio
2022.
Hurwitz generation in groups of types 𝐹4, 𝐸6, 2E6, 𝐸7 and 𝐸8
.
Journal of Group Theory,
Vol. 0,
Issue. 0,
Burness, Timothy C.
and
Lee, Melissa
2023.
On the classification of extremely primitive affine groups.
Israel Journal of Mathematics,
Vol. 255,
Issue. 1,
p.
265.
Galt, A. A.
and
Tyutyanov, V. N.
2023.
On the Existence of Hereditarily $ G $-Permutable Subgroups in Exceptional Groups $ G $ of Lie Type.
Siberian Mathematical Journal,
Vol. 64,
Issue. 5,
p.
1110.
Yin, Fu-Gang
Feng, Yan-Quan
and
Xia, Binzhou
2023.
The smallest vertex-primitive 2-arc-transitive digraph.
Journal of Algebra,
Vol. 626,
Issue. ,
p.
1.
2024.
Groups St Andrews 2022 in Newcastle.
p.
191.