Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-24T12:45:43.301Z Has data issue: false hasContentIssue false
  • English
  • Français

Effective Hasse principle for the intersection of two quadrics

Part of: Diophantine equations Computational number theory

Published online by Cambridge University Press:  26 August 2016

Tony Quertier
Tony Quertier*
Affiliation:
UMR 6139 - Laboratoire de Mathématiques Nicolas Oresme (LMNO), Université de Caen Normandie UFR des Sciences, Campus 2, Côte de nacre Bd Maréchal Juin, 14032 Caen cedex 5, France email [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider a smooth system of two homogeneous quadratic equations over $\mathbb{Q}$ in 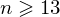$n\geqslant 13$ variables. In this case, the Hasse principle is known to hold, thanks to the work of Mordell in 1959. The only local obstruction is over $\mathbb{R}$. In this paper, we give an explicit algorithm to decide whether a nonzero rational solution exists and, if so, compute one.

MSC classification

Primary: 11D09: Quadratic and bilinear equations 11Y50: Computer solution of Diophantine equations
Type
Research Article
Information
LMS Journal of Computation and Mathematics , Volume 19 , Special Issue A: Algorithmic Number Theory Symposium XII , 2016 , pp. 73 - 82
Copyright
© The Author 2016 

References

Birch, B. J., Lewis, D. J. and Murphy, T. G., ‘Simultaneous quadratic forms’, Amer. J. Math. 84 (1962) 110–115.Google Scholar
Castel, P., ‘Solving quadratic equations in dimension 5 or more without factoring’, ANTS X — Proceedings of the Tenth Algorithmic Number Theory Symposium (Mathematical Sciences Publishers, Berkeley, CA, 2013).Google Scholar
Colliot-Thélène, J.-L., Sansuc, J.-J. and Swinnerton-Dyer, H. P. F., ‘Intersections de deux quadriques et surfaces de Châtelet’, C. R. Acad. Sci. Paris Sér. I Math. 298 (1984) 377–380.Google Scholar
Demyanov, V. B., ‘Pairs of quadratic forms over a complete field with discrete norm with a finite field of residue classes’, Izv. Akad. Nauk SSSR Ser. Mat. 20 (1956) 307–324.Google Scholar
Harris, J., Algebraic geometry (Springer, New York, 1995).Google Scholar
Mordell, L. J., ‘Integer solutions of simultaneous quadratic equations’, Abh. Math. Semin. Univ. Hambg. 23 (1959) 126–143.CrossRefGoogle Scholar
Serre, J.-P., A course in arithmetic (Springer, 1996).Google Scholar
Simon, D., ‘Solving quadratic equations using reduced unimodular quadratic forms’, Math. Comput. 74 (2005) no. 251, 1531–1543.Google Scholar
Swinnerton-Dyer, H. P. F., ‘Rational zeros of two quadratic forms’, Acta Arith. 9 (1964) 261–270.CrossRefGoogle Scholar
Wittenberg, O., ‘Principe de Hasse pour les intersections de deux quadriques’, C. R. Acad. Sci. Paris, Ser. I 342 (2006) no. 4, 223–227.CrossRefGoogle Scholar