Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-24T13:57:14.707Z Has data issue: false hasContentIssue false
  • English
  • Français

Constructing genus-3 hyperelliptic Jacobians with CM

Part of: Curves Computational aspects in algebraic geometry Arithmetic algebraic geometry

Published online by Cambridge University Press:  26 August 2016

Jennifer S. Balakrishnan ,
Sorina Ionica ,
Kristin Lauter  and
Christelle Vincent
Jennifer S. Balakrishnan
Affiliation:
Mathematical Institute, University of Oxford, Woodstock Road, Oxford OX2 6GG, United Kingdom email [email protected]
Sorina Ionica
Affiliation:
Laboratoire MIS, Université de Picardie Jules Verne, 33 Rue Saint Leu, 80000 Amiens, France email [email protected]
Kristin Lauter
Affiliation:
Microsoft Research, 1 Microsoft Way, Redmond, WA 98062, USA email [email protected]
Christelle Vincent
Affiliation:
Department of Mathematics and Statistics, The University of Vermont, 16 Colchester Avenue, Burlington, VT 05401, USA email [email protected]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

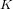Given a sextic CM field $K$, we give an explicit method for finding all genus-$3$ hyperelliptic curves defined over 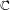$\mathbb{C}$ whose Jacobians are simple and have complex multiplication by the maximal order of this field, via an approximation of their Rosenhain invariants. Building on the work of Weng [J. Ramanujan Math. Soc. 16 (2001) no. 4, 339–372], we give an algorithm which works in complete generality, for any CM sextic field $K$, and computes minimal polynomials of the Rosenhain invariants for any period matrix of the Jacobian. This algorithm can be used to generate genus-3 hyperelliptic curves over a finite field 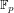$\mathbb{F}_{p}$ with a given zeta function by finding roots of the Rosenhain minimal polynomials modulo $p$.

MSC classification

Primary: 11G15: Complex multiplication and moduli of abelian varieties
Secondary: 11G30: Curves of arbitrary genus or genus $ne 1$ over global fields 14H42: Theta functions; Schottky problem 14Q05: Curves
Type
Research Article
Information
LMS Journal of Computation and Mathematics , Volume 19 , Special Issue A: Algorithmic Number Theory Symposium XII , 2016 , pp. 283 - 300
Copyright
© The Author(s) 2016 

References

Balakrishnan, J. S., Ionica, S., Lauter, K. and Vincent, C., ‘Genus 3’, 2016, https://github.com/christellevincent/genus3.Google Scholar
Birkenhake, C. and Lange, H., Complex abelian varieties , 2nd edn, Grundlehren der Mathematischen Wissenschaften 302 (Springer, Berlin, 2004).CrossRefGoogle Scholar
Cohen, H., A course in computational algebraic number theory , Graduate Texts in Mathematics 138 (Springer, Berlin, 1993).Google Scholar
Cosset, R., ‘Applications des fonctions thêta à la cryptographie sur les courbes hyperelliptiques’, PhD Thesis, Université Henri Poincaré – Nancy I, 2011.Google Scholar
Costello, C., Deines-Schartz, A., Lauter, K. and Yang, T., ‘Constructing abelian surfaces for cryptography via Rosenhain invariants’, LMS J. Comput. Math. 17 (2014) no. A, 157–180.Google Scholar
Diem, C., ‘An index calculus algorithm for plane curves of small degree’, Algorithmic number theory: 7th international symposium, ANTS VII , Lecture Notes in Computational Science 4076 (eds Hess, F., Pauli, S. and Pohst, M. E.; Springer, Berlin, 2006).Google Scholar
Dupont, R., ‘Moyenne arithmético-géométrique, suites de Borchardt et applications’, PhD Thesis, École Polytechnique, 2006.Google Scholar
Gaudry, P., Thomé, E., Thériault, N. and Diem, C., ‘A double large prime variation for small genus hyperelliptic index calculus’, Math. Comp. 76 (2007) 475–492.CrossRefGoogle Scholar
Gottschling, E., ‘Explizite Bestimmung der Randflächen des Fundamentalbereiches der Modulgruppe zweiten Grades’, Math. Ann. 138 (1959) 103–124.CrossRefGoogle Scholar
Gross, B. and Harris, J., On some geometric constructions related to theta characteristics (Johns Hopkins University Press, Baltimore, MD, 2004) 279–311.Google Scholar
Igusa, J., ‘Modular forms and projective invariants’, Amer. J. Math. 89 (1967) 817–855.Google Scholar
Igusa, J., Theta functions , Grundlehren der mathematischen Wissenschaften 194 (Springer, New York, 1972).Google Scholar
Koike, K. and Weng, A., ‘Construction of CM Picard curves’, Math. Comp. 74 (2005) no. 249, 499–518.Google Scholar
Laine, K. and Lauter, K., ‘Time-memory trade-offs for index calculus in genus 3’, J. Math. Cryptol. 9 (2015) no. 2, 95–114.Google Scholar
Lang, S., Complex multiplication , Grundlehren der Mathematischen Wissenschaften 255 (Springer, New York, 1983).Google Scholar
Mumford, D., Tata lectures on theta. I , Modern Birkhäuser classics (Birkhäuser, Boston, 2007), with the collaboration of C. Musili, M. Nori, E. Previato and M. Stillman, Reprint of the 1983 edition.Google Scholar
Mumford, D., Tata lectures on theta. II , Modern Birkhäuser classics (Birkhäuser, Boston, 2007) with the collaboration of C. Musili, M. Nori, E. Previato, M. Stillman and H. Umemura, Reprint of the 1984 original.Google Scholar
Poor, C., ‘The hyperelliptic locus’, Duke Math. J. 76 (1994) no. 3, 809–884.Google Scholar
Shimura, G. and Taniyama, Y., Complex multiplication of abelian varieties and its applications to number theory , Publications of the Mathematical Society of Japan 6 (Mathematical Society of Japan, Tokyo, 1961).Google Scholar
Smith, B., ‘Isogenies and the discrete logarithm problem in Jacobians of genus 3 hyperelliptic curves’, J. Cryptology 22 (2009) no. 4, 505–529.Google Scholar
Spallek, A.-M., ‘Kurven von Geschlecht 2 und ihre Anwendung in Public Key Kryptosystemen’, PhD Thesis, Institut für Experimentelle Mathematik, Universität GH Essen, 1994.Google Scholar
Stein, W. A. et al. , ‘Sage Mathematics Software (Version 6.10)’, The Sage Development Team, 2015, http://www.sagemath.org.Google Scholar
Streng, M., ‘Complex multiplication of abelian surfaces’, PhD Thesis, Universiteit Leiden, 2010.Google Scholar
Takase, K., ‘A generalization of Rosenhain’s normal form for hyperelliptic curves with an application’, Proc. Japan Acad. Ser. A Math. Sci. 72 (1996) no. 7, 162–165.CrossRefGoogle Scholar
van Wamelen, P., ‘Examples of genus two CM curves defined over the rationals’, Math. Comp. 68 (1999) no. 225, 307–320.CrossRefGoogle Scholar
Weber, H.-J., ‘Hyperelliptic simple factors of J 0(N) with dimension at least 3’, Experiment. Math. 6 (1997) no. 4, 273–287.Google Scholar
Weng, A., ‘A class of hyperelliptic CM-curves of genus three’, J. Ramanujan Math. Soc. 16 (2001) no. 4, 339–372.Google Scholar