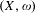Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Chu, Jianchun
Tosatti, Valentino
and
Weinkove, Ben
2018.
C1,1 regularity for degenerate complex Monge–Ampère equations and geodesic rays.
Communications in Partial Differential Equations,
Vol. 43,
Issue. 2,
p.
292.
Darvas, Tamás
Di Nezza, Eleonora
and
Lu, Chinh H.
2018.
Monotonicity of nonpluripolar products and complex Monge–Ampère equations with prescribed singularity.
Analysis & PDE,
Vol. 11,
Issue. 8,
p.
2049.
Darvas, Tamás
Di Nezza, Eleonora
and
Lu, Chinh H.
2018.
On the singularity type of full mass currents in big cohomology classes.
Compositio Mathematica,
Vol. 154,
Issue. 2,
p.
380.
Di Nezza, Eleonora
and
Guedj, Vincent
2018.
Geometry and topology of the space of Kähler metrics on singular varieties.
Compositio Mathematica,
Vol. 154,
Issue. 8,
p.
1593.
Abja, Soufian
2019.
Geometry and Topology of the Space of Plurisubharmonic Functions.
The Journal of Geometric Analysis,
Vol. 29,
Issue. 1,
p.
510.
Dyrefelt, Zakarias Sjöström
2019.
Moduli of K-stable Varieties.
Vol. 31,
Issue. ,
p.
103.
McCleerey, Nicholas
2020.
Envelopes with Prescribed Singularities.
The Journal of Geometric Analysis,
Vol. 30,
Issue. 4,
p.
3716.
Sjöström Dyrefelt, Zakarias
2020.
On K-Polystability of cscK Manifolds with Transcendental Cohomology Class.
International Mathematics Research Notices,
Vol. 2020,
Issue. 9,
p.
2769.
Darvas, Tamás
and
Lu, Chinh H
2020.
Geodesic stability, the space of rays and uniform convexity in Mabuchi geometry.
Geometry & Topology,
Vol. 24,
Issue. 4,
p.
1907.
Sjöström Dyrefelt, Zakarias
2020.
Optimal lower bounds for Donaldson's J-functional.
Advances in Mathematics,
Vol. 374,
Issue. ,
p.
107271.
Xia, Mingchen
2021.
On sharp lower bounds for Calabi-type functionals and destabilizing properties of gradient flows.
Analysis & PDE,
Vol. 14,
Issue. 6,
p.
1951.
Chu, Jianchun
and
McCleerey, Nicholas
2021.
C1,1 regularity of geodesics of singular Kähler metrics.
Journal of the London Mathematical Society,
Vol. 104,
Issue. 1,
p.
66.
Darvas, Tamás
Di Nezza, Eleonora
and
Lu, Hoang-Chinh
2021.
The metric geometry of singularity types.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2021,
Issue. 771,
p.
137.
Berman, Robert
Boucksom, Sébastien
and
Jonsson, Mattias
2021.
A variational approach to the Yau–Tian–Donaldson conjecture.
Journal of the American Mathematical Society,
Vol. 34,
Issue. 3,
p.
605.
Darvas, Tamás
and
Xia, Mingchen
2022.
The closures of test configurations and algebraic singularity types.
Advances in Mathematics,
Vol. 397,
Issue. ,
p.
108198.
Berndtsson, B.
2022.
Long Geodesics in the Space of Kähler Metrics.
Analysis Mathematica,
Vol. 48,
Issue. 2,
p.
377.
Darvas, Tamás
George, Erin
and
Smith, Kevin
2022.
Optimal asymptotic of the J functional with respect to the $$d_1$$ metric.
Selecta Mathematica,
Vol. 28,
Issue. 2,
Bachhuber, S.
Benda, A.
Christophel, B.
and
Darvas, T.
2022.
Extremizers of the J Functional with Respect to the d1 Metric.
Analysis Mathematica,
Vol. 48,
Issue. 2,
p.
307.
Hisamoto, Tomoyuki
2023.
Geometric Flow, Multiplier Ideal Sheaves and Optimal Destabilizer for a Fano Manifold.
The Journal of Geometric Analysis,
Vol. 33,
Issue. 8,
Xia, Mingchen
2023.
Pluripotential-Theoretic Stability Thresholds.
International Mathematics Research Notices,
Vol. 2023,
Issue. 14,
p.
12324.