Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T01:42:01.622Z Has data issue: false hasContentIssue false
  • English
  • Français

RECIPROCITY SHEAVES AND THEIR RAMIFICATION FILTRATIONS

Part of: $K$-theory in number theory Cycles and subschemes (Co)homology theory

Published online by Cambridge University Press:  19 March 2021

Kay Rülling Open the ORCID record for Kay Rülling [Opens in a new window]  and
Shuji Saito
Kay Rülling
Affiliation:
Bergische Universität Wuppertal, Gaußstr 20, 42119 Wuppertal, Germany and Technische Universität München, Boltzmannstr. 3, 85748 Garching, Germany ([email protected])
Shuji Saito
Affiliation:
Graduate School of Mathematical Sciences, University of Tokyo, 3-8-1 Komaba, Tokyo, 153-8914, Japan ([email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We define a motivic conductor for any presheaf with transfers F using the categorical framework developed for the theory of motives with modulus by Kahn, Miyazaki, Saito and Yamazaki. If F is a reciprocity sheaf, this conductor yields an increasing and exhaustive filtration on $F(L)$ , where L is any henselian discrete valuation field of geometric type over the perfect ground field. We show that if F is a smooth group scheme, then the motivic conductor extends the Rosenlicht–Serre conductor; if F assigns to X the group of finite characters on the abelianised étale fundamental group of X, then the motivic conductor agrees with the Artin conductor defined by Kato and Matsuda; and if F assigns to X the group of integrable rank 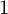$1$ connections (in characteristic 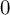$0$ ), then it agrees with the irregularity. We also show that this machinery gives rise to a conductor for torsors under finite flat group schemes over the base field, which we believe to be new. We introduce a general notion of conductors on presheaves with transfers and show that on a reciprocity sheaf, the motivic conductor is minimal and any conductor which is defined only for henselian discrete valuation fields of geometric type with perfect residue field can be uniquely extended to all such fields without any restriction on the residue field. For example, the Kato–Matsuda Artin conductor is characterised as the canonical extension of the classical Artin conductor defined in the case of a perfect residue field.

Keywords

Reciprocity sheafconductorramification

MSC classification

Primary: 14C25: Algebraic cycles
Secondary: 14F42: Motivic cohomology; motivic homotopy theory 19F15: Symbols and arithmetic
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 22 , Issue 1 , January 2023 , pp. 71 - 144
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (https://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution and reproduction, provided the original work is properly cited.
Copyright
© The Author(s), 2021. Published by Cambridge University Press

References

Artin, M., Grothendieck, A. and Verdier, J. L., Séminaire de géométrie algébrique du Bois-Marie 1963–1964. Théorie des topos et cohomologie étale des schémas. (SGA 4). Un séminaire dirigé par M. Artin, A. Grothendieck, J. L. Verdier. Avec la collaboration de Bourbaki, N., Deligne, P., Saint-Donat, B.. Tome 1: Théorie des topos. Exposés I à IV, 2nd ed., 269 (Springer, Cham, Switzerland, 1972).Google Scholar
Artin, M., Grothendieck, A. and Verdier, J. L., Séminaire de géométrie algébrique du Bois-Marie 1963–1964. Théorie des topos et cohomologie étale des schémas (SGA 4). Un séminaire dirigé par Artin, M., Grothendieck, A., Verdier, J. L.. Avec la collaboration de P. Deligne, B. Saint-Donat. Tome 3. Exposés IX à XIX, 305 (Springer, Cham, Switzerland, 1973).Google Scholar
Berthelot, P., Breen, L. and Messing, W., Théorie de Dieudonné cristalline. II, Lecture Notes in Mathematics, 930 (Springer-Verlag, Berlin, 1982).CrossRefGoogle Scholar
Bourbaki, N., Éléments de mathématique: Algèbre commutative. Chapitres 8 et 9 (Springer, Berlin, 2006). Reprint of the 1983 original.Google Scholar
Brylinski, J.-L., Théorie du corps de classes de Kato et revêtements abéliens de surfaces, Ann. Inst. Fourier (Grenoble) 33(3) (1983), 23–38.CrossRefGoogle Scholar
Chatzistamatiou, A. and Rülling, K., Higher direct images of the structure sheaf in positive characteristic, Algebra Number Theory 5(6) (2011), 693–775.CrossRefGoogle Scholar
Chatzistamatiou, A. and Rülling, K., Hodge-Witt cohomology and Witt-rational singularities, Doc . Math. 17 (2012), 663–781.Google Scholar
Colliot-Thélène, J.-L., Hoobler, R. T. and Kahn, B., The Bloch-Ogus-Gabber theorem, in Algebraic $K$ -theory (Toronto, ON, 1996), Fields Institute Communications, 16, pp. 31–94 (American Mathematical Society, Providence, RI, 1997).CrossRefGoogle Scholar
Costeanu, V., On the 2-typical de Rham-Witt complex, Doc. Math. 13 (2008), 413–452.CrossRefGoogle Scholar
Deligne, P., Équations différentielles à points singuliers réguliers, Lecture Notes in Mathematics, 163 (Springer-Verlag, Berlin, 1970).CrossRefGoogle Scholar
Deligne, P., La conjecture de Weil. II, Publ. Math. Inst. Hautes Études Sci. 52 (1980), 137–252.CrossRefGoogle Scholar
Demazure, M. and Gabriel, P., Groupes algébriques. Tome I: Géométrie algébrique, généralités, groupes commutatifs (Masson & Cie, Paris, 1970). With an appendix by M. Hazewinkel).Google Scholar
Demazure, M. and Grothendieck, A. (eds.), Schémas en groupes. I: Propriétés générales des schémas en groupes. Exposés I à VIIb. Séminaire de Géométrie Algébrique 1962/64, dirigé par Michel Demazure et Alexander Grothendieck, revised reprint, 151 (Springer, Cham, Switzerland, 1970).Google Scholar
Geisser, T. and Hesselholt, L., On the $K$ -theory of complete regular local ${F}_p$ -algebras, Topology 45(3) (2006), 475–493.Google Scholar
Gros, M., Classes de Chern et classes de cycles en cohomologie de Hodge-Witt logarithmique, Mém. Soc. Math. Fr. (N.S.) 21 (1985), 1–87.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. I, Publ. Math. Inst. Hautes Études Sci. 20 (1964), 5–259.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. II, Publ. Math. Inst. Hautes Études Sci. 24 (1965), 5–231.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. III, Publ. Math. Inst. Hautes Études Sci . 28 (1966), 5–255.CrossRefGoogle Scholar
Grothendieck, A., Le groupe de Brauer. III. Exemples et compléments, in Dix exposés sur la cohomologie des schémas, Advanced Studies in Pure Mathematics, 3, pp. 88–188 (North-Holland, Amsterdam, 1968).Google Scholar
Hartshorne, R., On the De Rham cohomology of algebraic varieties, Publ. Math. Inst. Hautes Études Sci. 45 (1975), 5–99.CrossRefGoogle Scholar
Hesselholt, L. and Madsen, I, On the De Rham-Witt complex in mixed characteristic, Ann. Sci. Éc. Norm. Supér. (4) 37(1) (2004), 1–43.CrossRefGoogle Scholar
Illusie, L., Complexe de de Rham-Witt et cohomologie cristalline, Ann. Sci. Éc. Norm. Supér. (4) 12(4) (1979), 501–661.Google Scholar
Ivorra, F. and Rülling, K., K-groups of reciprocity functors, J. Algebraic Geom. 26(2) (2017), 199–278.CrossRefGoogle Scholar
Kahn, B., Miyazaki, H., Saito, S. and Yamazaki, T., Motives with modulus, I: Modulus sheaves with transfers for non-proper modulus pairs, Épijournal Géom. Algébrique 5 (2021), https://epiga.episciences.org/7114.Google Scholar
Kahn, B., Miyazaki, H., Saito, S. and Yamazaki, T., Motives with modulus, II: Modulus sheaves with transfers for proper modulus pairs, Épijournal Géom. Algébrique 5 (2021) https://epiga.episciences.org/7115.Google Scholar
Kahn, B., Saito, S. and Yamazaki, T., Reciprocity sheaves, Compos. Math. 152(9) (2016), 1851–1898. With two appendices by K. Rülling.CrossRefGoogle Scholar
Kahn, B., Saito, S. and Yamazaki, T., Reciprocity sheaves, II, to appear in Homology, Homotopy and Applications https://arxiv.org/abs/1707.07398.Google Scholar
Kahn, B. and Yamazaki, T., Voevodsky’s motives and Weil reciprocity, Duke Math. J. 162(14) (2013), 2751–2796.CrossRefGoogle Scholar
Kato, K., A generalization of local class field theory by using $K$ -groups. II, J. Fac. Sci. Univ. Tokyo Sect. IA Math. 27(3) (1980), 603–683.Google Scholar
Kato, K., Swan conductors for characters of degree one in the imperfect residue field case, in Algebraic $K$ -theory and algebraic number theory (Honolulu, HI, 1987), Contemporary Mathematics, 83, pp. 101–131 (American Mathematical Society, Providence, RI, 1989).CrossRefGoogle Scholar
Kato, K., Class field theory, $\mathbf{\mathcal{D}}$ -modules, and ramification on higher-dimensional schemes. I, Amer. J. Math. 116(4) (1994), 757–784.CrossRefGoogle Scholar
Kato, K. and Russell, H., Modulus of a rational map into a commutative algebraic group, Kyoto J. Math. 50(3) (2010), 607–622.CrossRefGoogle Scholar
Kato, K. and Russell, H., Albanese varieties with modulus and Hodge theory, Ann. Inst. Fourier (Grenoble) 62(2) (2012), 783–806.CrossRefGoogle Scholar
Kerz, M. and Schmidt, A., On different notions of tameness in arithmetic geometry, Math. Ann. 346(3) (2010), 641–668.CrossRefGoogle Scholar
Kerz, M. and Saito, S., Chow group of 0-cycles with modulus and higher-dimensional class field theory, Duke Math. J. 165(15) (2016), 2811–2897.CrossRefGoogle Scholar
Langer, A. and Zink, T., De Rham-Witt cohomology for a proper and smooth morphism, J. Inst. Math. Jussieu 3(2) (2004), 231–314.CrossRefGoogle Scholar
Matsuda, S., On the Swan conductor in positive characteristic, Amer. J. Math. 119(4) (1997), 705–739.CrossRefGoogle Scholar
Matsumura, H., Commutative Ring Theory, Cambridge Studies in Advanced Mathematics, 8, 2nd ed. (Cambridge University Press, Cambridge, UK, 1989).Google Scholar
Mazza, C., Voevodsky, V. and Weibel, C., Lecture Notes on Motivic Cohomology, Clay Mathematics Monographs, 2 (American Mathematical Society, Providence, RI, 2006).Google Scholar
Milne, J. S., Étale Cohomology, Princeton Mathematical Series, 33 (Princeton University Press, Princeton, 1980).Google Scholar
Popescu, D., General Néron desingularization and approximation, Nagoya Math. J. 104 (1986), 85–115.CrossRefGoogle Scholar
Raynaud, M., Anneaux locaux henséliens, Lecture Notes in Mathematics, 169 (Springer-Verlag, Berlin, 1970).CrossRefGoogle Scholar
Rülling, K., The generalized de Rham-Witt complex over a field is a complex of zero-cycles, J. Algebraic Geom. 16(1) (2007), 109–169.CrossRefGoogle Scholar
Rülling, K., Erratum to: “The generalized de Rham-Witt complex over a field is a complex of zero-cycles” [J. Algebraic Geom. 16 (2007), no. 1, 109–169; mr2257322], J. Algebraic Geom. 16(4) (2007), 793–795.Google Scholar
Saito, S., Purity of reciprocity sheaves, Adv. Math. 366 (2020), 107067.CrossRefGoogle Scholar
Serre, J.-P., Algèbre locale. Multiplicités, Cours au Collège de France, 1957–1958, rédigé par Pierre Gabriel, Lecture Notes in Mathematics, 11, 2nd ed. (Springer-Verlag, Berlin, 1965).Google Scholar
Serre, J.-P., Groupes algébriques et corps de classes, Publications de l’Institut Mathématique de l’Université de Nancago, 7, 2nd ed. (Hermann, Paris, 1984).Google Scholar
Voevodsky, V., Cohomological theory of presheaves with transfers, in Cycles, transfers, and motivic homology theories, Annals of Mathematics Studies, 143, pp. 87–137 (Princeton University Press, Princeton, 2000).Google Scholar
Voevodsky, V., Triangulated categories of motives over a field, in Cycles, transfers, and motivic homology theories, Annals of Mathematics Studies, 143, pp. 188–238 (Princeton University Press, Princeton, 2000).Google Scholar