Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-23T01:43:00.303Z Has data issue: false hasContentIssue false
  • English
  • Français

THE p-COHOMOLOGY OF ALGEBRAIC VARIETIES AND SPECIAL VALUES OF ZETA FUNCTIONS

Part of: Arithmetic algebraic geometry Arithmetic problems. Diophantine geometry

Published online by Cambridge University Press:  02 June 2014

James S. Milne  and
Niranjan Ramachandran
James S. Milne
Affiliation:
Mathematics Department, University of Michigan, Ann Arbor, MI 48109, USA ([email protected]) URL: www.jmilne.org/math/
Niranjan Ramachandran
Affiliation:
Mathematics Department, University of Maryland, College Park, MD 20742, USA ([email protected]) URL: www2.math.umd.edu/∼atma/
Get access Rights & Permissions [Opens in a new window]

Abstract

The 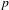$p$-cohomology of an algebraic variety in characteristic 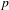$p$ lies naturally in the category 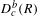$D_{c}^{b}(R)$ of coherent complexes of graded modules over the Raynaud ring (Ekedahl, Illusie, Raynaud). We study homological algebra in this category. When the base field is finite, our results provide relations between the absolute cohomology groups of algebraic varieties, log varieties, algebraic stacks, etc., and the special values of their zeta functions. These results provide compelling evidence that 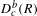$D_{c}^{b}(R)$ is the correct target for 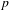$p$-cohomology in characteristic 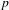$p$.

Keywords

zeta functionscrystalline cohomology

MSC classification

Primary: 14G10: Zeta-functions and related questions (Birch-Swinnerton-Dyer conjecture) 11G20: Curves over finite and local fields
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 14 , Issue 4 , October 2015 , pp. 801 - 835
Copyright
© Cambridge University Press 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Berthelot, P., Le théorème de dualité plate pour les surfaces (d’après J. S. Milne), in Algebraic surfaces (Orsay, 1976–78), Lecture Notes in Math, Volume 868, pp. 203–237(Springer, Berlin, 1981).Google Scholar
Berthelot, P., Géométrie rigide et cohomologie des variétés algébriques de caractéristique p, in Introductions aux cohomologies p-adiques (Luminy, 1984), Mém. Soc. Math. Fr. (N.S.), (1986) 3, 7–32.Google Scholar
Berthelot, P., Dualité de Poincaré et formule de Künneth en cohomologie rigide, C. R. Acad. Sci. Paris Sér. I Math. 325 (1997a), 493–498.Google Scholar
Berthelot, P., Finitude et pureté cohomologique en cohomologie rigide, Invent. Math. 128 (1997b), 329–377.Google Scholar
Bloch, S., Algebraic K-theory and crystalline cohomology, Inst. Hautes Études Sci. Publ. Math.(1977), 187–268.Google Scholar
Chiarellotto, B.and Le Stum, B., Sur la pureté de la cohomologie cristalline, C. R. Acad. Sci. Paris Sér. I Math. 326 (1998), 961–963.Google Scholar
Cisinski, D.-C.and Déglise, F., Mixed Weil cohomologies, Adv. Math. 230 (2012a), 55–130.Google Scholar
Cisinski, D.-C.and Déglise, F., (2012b), Triangulated categories of mixed motives. arXiv:0912.2110v3.Google Scholar
Deligne, P., A quoi servent les motifs?in Motives (Seattle, WA, 1991), Proc. Sympos. Pure Math, Volume 55, pp. 143–161 (Amer Math Soc, Providence, RI, 1994).Google Scholar
Demazure, M., Lectures on p-divisible groups, Lecture Notes in Mathematics, Volume 302(Springer, Berlin, 1972).Google Scholar
Dwork, B., On the rationality of the zeta function of an algebraic variety, Amer. J. Math. 82 (1960), 631–648.Google Scholar
Ekedahl, T., On the multiplicative properties of the de Rham–Witt complex I, Ark. Mat. 22 (1984), 185–239.Google Scholar
Ekedahl, T., On the multiplicative properties of the de Rham–Witt complex II, Ark. Mat. 23 (1985), 53–102.CrossRefGoogle Scholar
Ekedahl, T., Diagonal complexes and F-gauge structures(Travaux en Cours Hermann, Paris, 1986).Google Scholar
Ekedahl, T., On the adic formalism, in The Grothendieck Festschrift, Vol II, Progr Math, Volume 87, pp. 197–218(Birkhäuser Boston, Boston, MA, 1990).Google Scholar
Étesse, J.-Y., Complexe de de Rham–Witt à coefficients dans un cristal, Compositio Math. 66 (1988a), 57–120.Google Scholar
Étesse, J.-Y., Rationalité et valeurs de fonctions Len cohomologie cristalline, Ann. Inst. Fourier (Grenoble) 38 (1988b), 33–92.Google Scholar
Étesse, J.-Y.and Le Stum, B., Fonctions L associées aux F-isocristaux surconvergents I Interprétation cohomologique, Math. Ann. 296 (1993), 557–576.Google Scholar
Geisser, T., Arithmetic cohomology over finite fields and special values of 𝜁-functions, Duke Math. J. 133 (2006), 27–57.CrossRefGoogle Scholar
Grosse-Klönne, E., Finiteness of de Rham cohomology in rigid analysis, Duke Math. J. 113 (2002), 57–91.Google Scholar
Illusie, L., Finiteness, duality, and Künneth theorems in the cohomology of the de Rham–Witt complex, in Algebraic geometry (Tokyo/Kyoto, 1982), Lecture Notes in Math, Volume 1016, pp. 20–72(Springer, Berlin, 1983).Google Scholar
Illusie, L.and Raynaud, M., Les suites spectrales associées au complexe de de Rham–Witt, Inst. Hautes Études Sci. Publ. Math. (1983), 73–212.Google Scholar
Kato, K., Logarithmic structures of Fontaine-Illusie, in Algebraic analysis, geometry, and number theory (Baltimore, MD, 1988), pp. 191–224(Johns Hopkins Univ. Press, Baltimore, MD, 1989).Google Scholar
Katz, N. M.and Messing, W., Some consequences of the Riemann hypothesis for varieties over finite fields, Invent. Math. 23 (1974), 73–77.Google Scholar
Le Stum, B., Rigid cohomology, in Cambridge Tracts in Mathematics, Volume 172(Cambridge University Press, Cambridge, 2007).Google Scholar
Lorenzon, P., Logarithmic Hodge–Witt forms and Hyodo–Kato cohomology, J. Algebra 249 (2002), 247–265.CrossRefGoogle Scholar
Milne, J. S., Duality in the flat cohomology of a surface, Ann. Sci. Éc. Norm. Super. (4) 9 (1976), 171–201.Google Scholar
Milne, J. S., Values of zeta functions of varieties over finite fields, Amer. J. Math. 108 (1986), 297–360; Addendum, Amer. J. Math., to appear.CrossRefGoogle Scholar
Milne, J. S.and Ramachandran, N., The de Rham–Witt and ℤp -cohomologies of an algebraic variety, Adv. Math 198 (2005), 36–42.CrossRefGoogle Scholar
Milne, J. S.and Ramachandran, N., (2013), Motivic complexes and special values of zeta functions. arXiv:1311.3166.Google Scholar
Nakkajima, Y., p-adic weight spectral sequences of log varieties, J. Math. Sci. Univ. Tokyo 12 (2005), 513–661.Google Scholar
Nakkajima, Y., Weight filtration and slope filtration on the rigid cohomology of a variety in characteristic p> 0, Mém. Soc. Math. Fr. (N.S.) (2012), 130–131:vi+250.Google Scholar
Nakkajima, Y.and Shiho, A., Weight filtrations on log crystalline cohomologies of families of open smooth varieties, Lecture Notes in Mathematics, Volume 1959 (Springer, Berlin, 2008).Google Scholar
Olsson, M. C., Crystalline cohomology of algebraic stacks and Hyodo-Kato cohomology, Astérisque 316 (2007), 412.Google Scholar
Petrequin, D., Classes de Chern et classes de cycles en cohomologie rigide, Bull. Soc. Math. France 131 (2003), 59–121.Google Scholar
Rapoport, M.and Zink, T., Über die lokale Zetafunktion von Shimuravarietäten Monodromiefiltration und verschwindende Zyklen in ungleicher Charakteristik, Invent. Math. 68 (1982), 21–101.Google Scholar
Suh, J., Symmetry and parity in Frobenius action on cohomology, Compos. Math. 148 (2012), 295–303.Google Scholar
Sun, S., Decomposition theorem for perverse sheaves on Artin stacks over finite fields, Duke Math. J. 161 (2012), 2297–2310.Google Scholar
Tate, J. T., On the conjectures of Birch and Swinnerton-Dyer and a geometric analog, Séminaire Bourbaki 1965/66 (1966), Expose 306.Google Scholar
Tsuzuki, N., Cohomological descent of rigid cohomology for proper coverings, Invent. Math. 151 (2003), 101–133.Google Scholar
Voevodsky, V., Suslin, A.and Friedlander, E. M., Cycles, transfers, and motivic homology theories, Ann. Math. Stud., Volume 143 (Princeton University Press, Princeton, NJ, 2000).Google Scholar