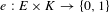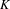Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ravsky, O.
and
Banakh, T.
2021.
SEMITOPOLOGICAL MODULES.
Bukovinian Mathematical Journal,
Vol. 9,
Issue. 1,
p.
9.
Mykhaylyuk, V.
and
Karlova, O.
2021.
A GENERALIZATION OF SIERPINSKI THEOREM ON UNIQUE DETERMINING OF A SEPARATELY CONTINUOUS FUNCTION.
Bukovinian Mathematical Journal,
Vol. 9,
Issue. 1,
p.
250.
Ferrer, M.
Hernández, S.
Sepúlveda, I.
and
Trigos-Arrieta, F.
2024.
The Baire property and precompact duality.
Proceedings of the American Mathematical Society, Series B,
Vol. 11,
Issue. 53,
p.
612.