Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-23T02:01:18.107Z Has data issue: false hasContentIssue false
  • English
  • Français

HECKE OPERATORS AND THE COHERENT COHOMOLOGY OF SHIMURA VARIETIES

Part of: Arithmetic algebraic geometry Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  08 April 2021

Najmuddin Fakhruddin  and
Vincent Pilloni Open the ORCID record for Vincent Pilloni [Opens in a new window]
Najmuddin Fakhruddin
Affiliation:
School of Mathematics, Tata Institute of Fundamental Research, Homi Bhabha Road, Mumbai 400005, INDIA ([email protected])
Vincent Pilloni
Affiliation:
CNRS, Ecole Normale Supérieure de Lyon, Lyon, FRANCE ([email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We consider the problem of defining an action of Hecke operators on the coherent cohomology of certain integral models of Shimura varieties. We formulate a general conjecture describing which Hecke operators should act integrally and solve the conjecture in certain cases. As a consequence, we obtain p-adic estimates of Satake parameters of certain nonregular self-dual automorphic representations of 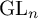$\mathrm {GL}_n$ .

Keywords

automorphic formsShimura varietiesHecke operators

MSC classification

Primary: 11F46: Siegel modular groups; Siegel and Hilbert-Siegel modular and automorphic forms
Secondary: 11G18: Arithmetic aspects of modular and Shimura varieties
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 22 , Issue 1 , January 2023 , pp. 1 - 69
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

References

Arthur, J. and Clozel, L., Simple Algebras, Base Change, and the Advanced Theory of the Trace Formula, Annals of Mathematics Studies, Vol. 120 (Princeton University Press, Princeton, NJ, 1989).Google Scholar
Ash, A., Mumford, D., Rapoport, M. and Tai, Y.-S., Smooth Compactifications of Locally Symmetric Varieties, 2nd ed., Cambridge Mathematical Library (Cambridge University Press, Cambridge, 2010). With the collaboration of Peter Scholze.Google Scholar
Barnet-Lamb, T., Gee, T., Geraghty, D., and Taylor, R., Potential automorphy and change of weight, Ann. Math. (2) 179(2) (2014), 501–609.CrossRefGoogle Scholar
Borel, A., Automorphic $L$ -functions, automorphic forms, representations and $L$ -functions, in Proc. Sympos. Pure Math., XXXIII (American Mathematical Society, Providence, RI, 1979), 27–61.Google Scholar
Boxer, G., Calegari, F., Gee, T. and Pilloni, V., Abelian surfaces over totally real fields are potentially modular, Preprint, 2018, arXiv:1812.09269.Google Scholar
Bruhat, F. and Tits, J., Groupes réductifs sur un corps local, Inst. Hautes Études Sci. Publ. Math. (41) (1972), 5–251.CrossRefGoogle Scholar
Bruns, W. and Herzog, H. J., Cohen-Macaulay Rings, Cambridge Studies in Advanced Mathematics (Cambridge University Press, Cambridge, 1998).CrossRefGoogle Scholar
Buzzard, K. and Gee, T., The conjectural connections between automorphic representations and Galois representations , in Automorphic forms and Galois representations, Vol. 1, London Math. Soc. Lecture Note Ser., Vol. 414 (Cambridge University Press, Cambridge, 2014), 135–187.CrossRefGoogle Scholar
Cartier, P., Representations of $p$ -adic groups: a survey, in Automorphic Forms, Representations and $L$ -Functions, Part 1, Proc. Sympos. Pure Math., XXXIII (American Mathematical Society, Providence, RI, 1979), 111–155.Google Scholar
Chai, C.-L. and Norman, P., Singularities of the ${\varGamma}_0(p)$ -level structure, J. Algebraic Geom. 1(2) (1992), 251–278.Google Scholar
Chenevier, G., On restrictions and extensions of cusp forms, Preprint, 2018.Google Scholar
Chenevier, G. and Harris, M., Construction of automorphic Galois representations, II , Camb. J. Math. 1(1) (2013), 53–73.CrossRefGoogle Scholar
Clozel, L., Motifs et formes automorphes: applications du principe de fonctorialité, in Automorphic Forms, Shimura Varieties, and $L$ -Functions, Vol. I, Perspect. Math., Vol. 10 (Academic Press, Boston, 1990), 77–159.Google Scholar
Conrad, B., Grothendieck duality and base change, Lecture Notes in Mathematics, Vol. 1750 Springer, Berlin, 2000).Google Scholar
Conrad, B., Arithmetic moduli of generalized elliptic curves, J. Inst. Math. Jussieu 6(2) (2007), 209–278.CrossRefGoogle Scholar
de Jong, A. J., The moduli spaces of principally polarized abelian varieties with ${\varGamma}_0(p)$ -level structure, J. Algebraic Geom. 2(4) (1993), 667–688.Google Scholar
Deligne, P., Variétés de Shimura: interprétation modulaire, et techniques de construction de modèles canoniques, in Automorphic Forms, Representations and $L$ -Functions, Part 2, Proc. Sympos. Pure Math., XXXIII (American Mathematical Society, Providence, RI, 1979), 247–289.Google Scholar
Deligne, Pierre and Pappas, Georgios, Singularités des espaces de modules de Hilbert, en les caractéristiques divisant le discriminant, Compos. Math. 90(1) (1994), 59–79.Google Scholar
Emerton, M., Reduzzi, D. and Xiao, L., Unramifiedness of Galois representations arising from Hilbert modular surfaces, Forum Math. Sigma 5 (2017), e29, 70.CrossRefGoogle Scholar
Faltings, G. and Chai, C.-L., Degeneration of abelian varieties, in Ergebnisse der Mathematik und ihrer Grenzgebiete (3) [Results in Mathematics and Related Areas (3)], Vol. 22 (Springer, Berlin, 1990). With an appendix by David Mumford.Google Scholar
Goldring, W., Galois representations associated to holomorphic limits of discrete series, Compos. Math. 150(2) (2014), 191–228.CrossRefGoogle Scholar
Goldring, W. and Koskivirta, J.-S., Strata Hasse invariants, Hecke algebras and Galois representations, Invent. Math. 217(3) (2019), 887–984.CrossRefGoogle Scholar
Görtz, U., On the flatness of models of certain Shimura varieties of PEL-type, Math. Ann. 321(3) (2001), 689–727.CrossRefGoogle Scholar
Görtz, U., On the flatness of local models for the symplectic group, Adv. Math. 176(1) (2003), 89–115.CrossRefGoogle Scholar
Gross, B. H., On the Satake isomorphism, in Galois Representations in Arithmetic Algebraic Geometry , London Math. Soc. Lecture Note Ser., Vol. 254 (Cambridge University Press, Cambridge, 1998), 223–237.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. II, Inst. Hautes Études Sci. Publ. Math. (24) (1965), 231.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas, troisième partie, Inst. Hautes Études Sci. Publ. Math. (28) (1966), 255.Google Scholar
Harris, M., Functorial properties of toroidal compactifications of locally symmetric varieties, Proc. London Math. Soc. (3) 59(1) (1989), 1–22.CrossRefGoogle Scholar
Harris, M., Automorphic forms and the cohomology of vector bundles on Shimura varieties, Automorphic Forms, Shimura Varieties, and $L$ -Functions, Vol. II, Perspect. Math., Vol. 11 (Academic Press, Boston, 1990), 41–91.Google Scholar
Harris, M., Automorphic forms of $\overline{\partial}$ -cohomology type as coherent cohomology classes, J. Differ. Geom. 32(1) (1990), 1–63.Google Scholar
Harris, M. and Taylor, R., The Geometry and Cohomology of Some Simple Shimura Varieties , Annals of Mathematics Studies, Vol. 151 (Princeton University Press, Princeton, NJ, 2001). With an appendix by Vladimir G. Berkovich.Google Scholar
Hartshorne, R., Residues and Duality , Lecture Notes in Mathematics, No. 20 (Springer, Berlin, 1966). Lecture notes of a seminar on the work of A. Grothendieck, given at Harvard 1963/64. With an appendix by P. Deligne.Google Scholar
He, X., Normality and Cohen-Macaulayness of local models of Shimura varieties, Duke Math. J. 162(13) (2013), 2509–2523.CrossRefGoogle Scholar
Henniart, G., Une preuve simple des conjectures de Langlands pour $GL(n)$ sur un corps $p$ -adique, Invent. Math. 139(2) (2000), 439–455.CrossRefGoogle Scholar
Hiraga, K. and Saito, H., On $L$ -packets for inner forms of $S{L}_n$ , Mem. Amer. Math. Soc. 215(1013) (2012).Google Scholar
Illusie, L., Complexe cotangent et déformations. I , Lecture Notes in Mathematics, Vol. 239 (Springer, Berlin, 1971).Google Scholar
Jun, S., Coherent cohomology of Shimura varieties and automorphic forms, Preprint, 2018, arXiv:1810.12056.Google Scholar
Katz, N. M., $p$ -Adic properties of modular schemes and modular forms, in Modular Functions of One Variable, III, Lecture Notes in Mathematics, Vol. 350 (Springer, Berlin, 1973), 69–190.Google Scholar
Kim, W. and Pera, K. M., 2-Adic integral canonical models, Forum Math. Sigma 4 (2016), e28, 34.CrossRefGoogle Scholar
Kisin, M., Integral models for Shimura varieties of abelian type, J. Amer. Math. Soc. 23(4) (2010), 967–1012.CrossRefGoogle Scholar
Knudsen, F. F. and Mumford, D., The projectivity of the moduli space of stable curves. I. Preliminaries on ‘det’ and ‘Div’, Math. Scand. 39(1) (1976), 19–55 . CrossRefGoogle Scholar
Kottwitz, R. E., Points on some Shimura varieties over finite fields, J. Amer. Math. Soc. 5(2) (1992), 373–444.CrossRefGoogle Scholar
Kottwitz, R. and Rapoport, M., Minuscule alcoves for ${\mathrm{GL}}_{\mathrm{n}}$ and $\mathrm{G}{\mathrm{Sp}}_{2\mathrm{n}}$ , Manuscripta Math. 102 (2000), no. 4, 403–428.CrossRefGoogle Scholar
Lafforgue, V., Estimées pour les valuations $p$ -adiques des valeurs propres des opérateurs de Hecke, Bull. Soc. Math. France 139(4) (2011), 455–477.CrossRefGoogle Scholar
Lan, K.-W., Toroidal compactifications of PEL-type Kuga families, Algebra Number Theory 6(5) (2012), 885–966.CrossRefGoogle Scholar
Lan, K.-W., Arithmetic Compactifications of PEL-Type Shimura Varieties , London Mathematical Society Monographs Series , Vol. 36 (Princeton University Press, Princeton, NJ, 2013).Google Scholar
Lan, K.-W., Integral models of toroidal compactifications with projective cone decompositions, Int. Math. Res. Not. 11 (2017), 3237–3280.Google Scholar
Lipman, J., Notes on derived functors and Grothendieck duality, in Foundations of Grothendieck Duality for Diagrams of Schemes, Lecture Notes in Math., Vol. 1960 (Springer, Berlin, 2009), 1–259.CrossRefGoogle Scholar
Milne, J. S., The action of an automorphism of $\mathrm{C}$ on a Shimura variety and its special points, Arithmetic and Geometry, Vol. I, Progr. Math., Vol. 35 (Birkhäuser, Boston, 1983), 239–265.CrossRefGoogle Scholar
Milne, J. S., Canonical models of (mixed) Shimura varieties and automorphic vector bundles, Automorphic Forms, Shimura Varieties, and $L$ -Functions, Vol. I, Perspect. Math., Vol. 10 (Academic Press, Boston, 1990), 283–414.Google Scholar
Mok, C. P., Endoscopic classification of representations of quasi-split unitary groups, Mem. Amer. Math. Soc. 235(1108) (2015).Google Scholar
Mumford, D., Fogarty, J. and Kirwan, F., Geometric Invariant Theory , 3rd ed., Ergebnisse der Mathematik und ihrer Grenzgebiete (2) [Results in Mathematics and Related Areas (2)], Vol. 34 (Springer, Berlin, 1994).Google Scholar
Neeman, A., The Grothendieck duality theorem via Bousfield’s techniques and Brown representability, J. Amer. Math. Soc. 9(1) (1996), 205–236.CrossRefGoogle Scholar
Pappas, G., Rapoport, M. and Smithling, B., Local models of Shimura varieties, I, Geometry and Combinatorics, Handbook of Moduli, Vol. III, Adv. Lect. Math. (ALM), Vol. 26 (Int. Press, Somerville, MA, 2013), 135–217.Google Scholar
Pera, K. M., Toroidal compactifications of integral models of Shimura varieties of Hodge type, Ann. Sci. Éc. Norm. Supér. (4) 52(2) (2019), 393–514.CrossRefGoogle Scholar
Pilloni, V. and Stroh, B., Cohomologie cohérente et représentations Galoisiennes, Ann. Math. Qué. 40(1) (2016), 167–202.CrossRefGoogle Scholar
Pink, R., Arithmetical Compactification of Mixed Shimura Varieties (Dissertation, Rheinische Friedrich-Wilhelms-Universität Bonn, Bonn, 1989).Google Scholar
Satake, I., Theory of spherical functions on reductive algebraic groups over $p$ -adic fields, Inst. Hautess Études Sci. Publ. Math. (18) (1963), 5–69.CrossRefGoogle Scholar
Shahidi, F., On non-vanishing of twisted symmetric and exterior square $L$ -functions for $GL(n)$ , Pacific J. Math. (1997), 311–322, Olga Taussky-Todd: In Memoriam.Google Scholar
Sorensen, C.. (2020). A Patching Lemma. In Haines, T. & Harris, M. (Eds.), Shimura Varieties (London Mathematical Society Lecture Note Series, pp. 297–305). Cambridge: Cambridge University Press. doi:10.1017/9781108649711.012.Google Scholar
Verdier, J.-L., Base change for twisted inverse image of coherent sheaves, in Algebraic Geometry (Oxford University Press, London, 1969), 393–408.Google Scholar