Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-23T02:01:12.906Z Has data issue: false hasContentIssue false
  • English
  • Français

GALOIS CONJUGATES OF SPECIAL POINTS AND SPECIAL SUBVARIETIES IN SHIMURA VARIETIES

Part of: Arithmetic algebraic geometry Arithmetic problems. Diophantine geometry

Published online by Cambridge University Press:  30 October 2019

Martin Orr Open the ORCID record for Martin Orr [Opens in a new window]
Martin Orr*
Affiliation:
Mathematics Institute, University of Warwick, CoventryCV4 7AL, UK ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $S$ be a Shimura variety with reflex field $E$. We prove that the action of 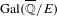$\text{Gal}(\overline{\mathbb{Q}}/E)$ on $S$ maps special points to special points and special subvarieties to special subvarieties. Furthermore, the Galois conjugates of a special point all have the same complexity (as defined in the theory of unlikely intersections). These results follow from Milne and Shih’s construction of canonical models of Shimura varieties, based on a conjecture of Langlands which was proved by Borovoi and Milne.

Keywords

Shimura varietiescanonical modelsspecial points

MSC classification

Primary: 11G18: Arithmetic aspects of modular and Shimura varieties 14G35: Modular and Shimura varieties
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 20 , Issue 3 , May 2021 , pp. 1075 - 1089
Check for updates
Copyright
© Cambridge University Press 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Borovoĭ, M. V., The Langlands conjecture on the conjugation of Shimura varieties, Funktsional. Anal. i Prilozhen. 16(4) (1982), 61–62.Google Scholar
Borovoĭ, M. V., Langlands’ conjecture concerning conjugation of connected Shimura varieties, Selecta Math. Soviet. 3(1) (1983/84), 3–39.Google Scholar
Borovoĭ, M. V., The group of points of a semisimple group over a totally real closed field, in Problems in Group Theory and Homological Algebra (Russian), Matematika, pp. 142–149 (Yaroslav. Gos. Univ., Yaroslavl, 1987).Google Scholar
Daw, C., The André–Oort conjecture via o-minimality, in O-Minimality and Diophantine Geometry, London Mathematical Society Lecture Note Series, Volume 421, pp. 129–158 (Cambridge University Press, Cambridge, 2015).CrossRefGoogle Scholar
Daw, C. and Ren, J., Applications of the hyperbolic Ax–Schanuel conjecture, Compos. Math. 154 (2018), 1843–1888.CrossRefGoogle Scholar
Deligne, P., Travaux de Shimura, in Séminaire Bourbaki, 23ème année (1970/71), Exp. No. 389, Lecture Notes in Mathematics, Volume 244, pp. 123–165 (Springer-Verlag, Berlin, 1971).CrossRefGoogle Scholar
Deligne, P., La conjecture de Weil pour les surfaces K3, Invent. Math. 15 (1972), 206–226.CrossRefGoogle Scholar
Deligne, P., Variétés de Shimura: interprétation modulaire, et techniques de construction de modèles canoniques, in Automorphic Forms, Representations and L-Functions (Part 2), Proceedings of Symposia in Pure Mathematics, Volume XXXIII, pp. 247–289 (American Mathematical Society, Providence, RI, 1979).CrossRefGoogle Scholar
Deligne, P., Motifs et groupe de Taniyama, in Hodge Cycles, Motives, and Shimura Varieties, Lecture Notes in Mathematics, Volume 900, pp. 261–279 (Springer, Berlin, 1982).CrossRefGoogle Scholar
Kazhdan, D., On arithmetic varieties. II, Israel J. Math. 44(2) (1983), 139–159.CrossRefGoogle Scholar
Langlands, R. P., Automorphic representations, Shimura varieties, and motives. Ein Märchen, in Automorphic Forms, Representations and L-Functions (Part 2), Proceedings of Symposia in Pure Mathematics, Volume XXXIII, pp. 205–246 (American Mathematical Society, Providence, RI, 1979).CrossRefGoogle Scholar
Milne, J. S., The action of an automorphism of C on a Shimura variety and its special points, in Arithmetic and Geometry, Vol. I, Progress in Mathematics, Volume 35, pp. 239–265 (Birkhäuser Boston, Boston, MA, 1983).CrossRefGoogle Scholar
Milne, J. S., Descent for Shimura varieties, Michigan Math. J. 46(1) (1999), 203–208.CrossRefGoogle Scholar
Milne, J. S. and Shih, K. Y., Langlands’s construction of the Taniyama group, in Hodge Cycles, Motives, and Shimura Varieties, Lecture Notes in Mathematics, Volume 900, pp. 229–260 (Springer, Berlin, 1982).CrossRefGoogle Scholar
Milne, J. S. and Shih, K. Y., Conjugates of Shimura varieties, in Hodge Cycles, Motives, and Shimura Varieties, Lecture Notes in Mathematics, Volume 900, pp. 280–356 (Springer, Berlin, 1982).CrossRefGoogle Scholar
Pink, R., A combination of the conjectures of Mordell–Lang and André–Oort, in Geometric Methods in Algebra and Number Theory, Progress in Mathematics, Volume 235, pp. 251–282 (Birkhäuser Boston, Boston, MA, 2005).CrossRefGoogle Scholar
Ullmo, E. and Yafaev, A., Mumford–Tate and generalised Shafarevich conjectures, Ann. Math. Qué. 37(2) (2013), 255–284.CrossRefGoogle Scholar
Ullmo, E. and Yafaev, A., Galois orbits and equidistribution of special subvarieties: towards the André–Oort conjecture, Ann. of Math. (2) 180(3) (2014), 823–865.CrossRefGoogle Scholar