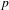 $p$-ADIC TORI
$p$-ADIC TORIPublished online by Cambridge University Press: 13 October 2015
We consider the rigid monoidal category of character sheaves on a smooth commutative group scheme 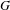 $G$ over a finite field
$G$ over a finite field 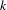 $k$, and expand the scope of the function-sheaf dictionary from connected commutative algebraic groups to this setting. We find the group of isomorphism classes of character sheaves on
$k$, and expand the scope of the function-sheaf dictionary from connected commutative algebraic groups to this setting. We find the group of isomorphism classes of character sheaves on 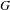 $G$, and show that it is an extension of the group of characters of
$G$, and show that it is an extension of the group of characters of 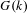 $G(k)$ by a cohomology group determined by the component group scheme of
$G(k)$ by a cohomology group determined by the component group scheme of 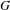 $G$. We also classify all morphisms in the category character sheaves on
$G$. We also classify all morphisms in the category character sheaves on  $G$. As an application, we study character sheaves on Greenberg transforms of locally finite type Néron models of algebraic tori over local fields. This provides a geometrization of quasicharacters of
$G$. As an application, we study character sheaves on Greenberg transforms of locally finite type Néron models of algebraic tori over local fields. This provides a geometrization of quasicharacters of 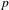 $p$-adic tori.
$p$-adic tori.