Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Breuil, Christophe
2015.
Induction parabolique et (φ,Γ)-modules.
Algebra & Number Theory,
Vol. 9,
Issue. 10,
p.
2241.
Hauseux, Julien
2018.
Parabolic induction and extensions.
Algebra & Number Theory,
Vol. 12,
Issue. 4,
p.
779.
Hauseux, Julien
2018.
On the exactness of ordinary parts over a local field of characteristic p.
Pacific Journal of Mathematics,
Vol. 295,
Issue. 1,
p.
17.
Hauseux, Julien
2019.
Sur une conjecture de Breuil–Herzig.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2019,
Issue. 751,
p.
91.
Colmez, Pierre
Dospinescu, Gabriel
Hauseux, Julien
and
Nizioł, Wiesława
2021.
p-adic étale cohomology of period domains.
Mathematische Annalen,
Vol. 381,
Issue. 1-2,
p.
105.
Gehrmann, Lennart
2021.
Automorphic ℒ‐invariants for reductive groups.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2021,
Issue. 779,
p.
57.
Heyer, Claudius
2023.
The left adjoint of derived parabolic induction.
Mathematische Zeitschrift,
Vol. 305,
Issue. 3,
Abe, Noriyuki
2023.
EXTENSION BETWEEN SIMPLE MODULES OF PRO-p-IWAHORI HECKE ALGEBRAS.
Journal of the Institute of Mathematics of Jussieu,
Vol. 22,
Issue. 6,
p.
2775.
Colmez, Pierre
Dospinescu, Gabriel
and
Nizioł, Wiesława
2023.
Factorisation de la cohomologie étale p-adique de la tour de Drinfeld.
Forum of Mathematics, Pi,
Vol. 11,
Issue. ,
Breuil, Christophe
and
Ding, Yiwen
2023.
Sur un problème de compatibilité local-global localement analytique.
Memoirs of the American Mathematical Society,
Vol. 290,
Issue. 1442,
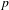 $p$-ADIQUES ET MODULO
$p$-ADIQUES ET MODULO 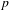 $p$ DE
$p$ DE 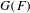 $G(F)$
$G(F)$ $G$ un groupe réductif connexe déployé sur une extension finie
$G$ un groupe réductif connexe déployé sur une extension finie  $F$ de
$F$ de 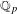 $\mathbb{Q}_{p}$. Nous déterminons les extensions entre séries principales continues unitaires
$\mathbb{Q}_{p}$. Nous déterminons les extensions entre séries principales continues unitaires 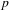 $p$-adiques et lisses modulo
$p$-adiques et lisses modulo 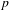 $p$ de
$p$ de  $G(F)$ dans le cas générique. Pour cela, nous calculons le delta-foncteur
$G(F)$ dans le cas générique. Pour cela, nous calculons le delta-foncteur 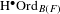 $\text{H}^{\bullet }\text{Ord}_{B(F)}$ des parties ordinaires dérivées d’Emerton relatif à un sous-groupe de Borel sur certaines représentations induites de
$\text{H}^{\bullet }\text{Ord}_{B(F)}$ des parties ordinaires dérivées d’Emerton relatif à un sous-groupe de Borel sur certaines représentations induites de 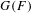 $G(F)$ en utilisant une filtration de Bruhat. Ces extensions interviennent dans le programme de Langlands
$G(F)$ en utilisant une filtration de Bruhat. Ces extensions interviennent dans le programme de Langlands  $p$-adique et modulo
$p$-adique et modulo  $p$.
$p$.