Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Dai, Irving
2019.
Connected Heegaard Floer homology of sums of Seifert fibrations.
Algebraic & Geometric Topology,
Vol. 19,
Issue. 5,
p.
2535.
Daemi, Aliakbar
2020.
Chern–Simons functional and the homology cobordism group.
Duke Mathematical Journal,
Vol. 169,
Issue. 15,
Zhou, Hugo
2021.
Homology concordance and an infinite rank free subgroup.
Journal of Topology,
Vol. 14,
Issue. 4,
p.
1369.
Karakurt, Ç.
and
Şavk, O.
2022.
Almost simple linear graphs, homology cobordism and connected Heegaard Floer homology.
Acta Mathematica Hungarica,
Vol. 168,
Issue. 2,
p.
454.
Hom, Jennifer
Kang, Sungkyung
Park, JungHwan
and
Stoffregen, Matthew
2023.
Linear independence of rationally slice knots.
Geometry & Topology,
Vol. 26,
Issue. 7,
p.
3143.
Şavk, Oğuz
2023.
A survey of the homology cobordism group.
Bulletin of the American Mathematical Society,
Dai, Irving
Hom, Jennifer
Stoffregen, Matthew
and
Truong, Linh
2023.
An infinite-rank summand of the homology cobordism group.
Duke Mathematical Journal,
Vol. 172,
Issue. 12,
Dai, Irving
Hedden, Matthew
Mallick, Abhishek
and
Stoffregen, Matthew
2024.
Rank‐expanding satellites, Whitehead doubles, and Heegaard Floer homology.
Journal of Topology,
Vol. 17,
Issue. 4,
Dai, Irving
Mallick, Abhishek
and
Zemke, Ian
2024.
Gompf’s Cork and Heegaard Floer Homology.
International Mathematics Research Notices,
Vol. 2024,
Issue. 18,
p.
12663.
Konno, Hokuto
Miyazawa, Jin
and
Taniguchi, Masaki
2024.
Involutions, links, and Floer cohomologies.
Journal of Topology,
Vol. 17,
Issue. 2,
Daemi, Aliakbar
and
Scaduto, Christopher
2024.
Equivariant aspects of singular instanton Floer homology.
Geometry & Topology,
Vol. 28,
Issue. 9,
p.
4057.
Alfieri, Antonio
and
Boyle, Keegan
2024.
Strongly Invertible Knots, Invariant Surfaces, and the Atiyah–Singer Signature Theorem.
Michigan Mathematical Journal,
Vol. 74,
Issue. 4,
Mallick, Abhishek
2024.
Knot Floer homology and surgery on equivariant knots.
Journal of Topology,
Vol. 17,
Issue. 4,
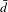 $\bar{d}$ and
$\bar{d}$ and 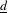 $\text{}\underline{d}$ for certain families of three-manifolds.
$\text{}\underline{d}$ for certain families of three-manifolds.