Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-23T09:24:16.114Z Has data issue: false hasContentIssue false
  • English
  • Français

SPECTRAL TRANSFER FOR METAPLECTIC GROUPS. I. LOCAL CHARACTER RELATIONS

Part of: Discontinuous groups and automorphic forms Lie groups

Published online by Cambridge University Press:  07 December 2016

Wen-Wei Li
Wen-Wei Li*
Affiliation:
Academy of Mathematics and Systems Science, Chinese Academy of Sciences, 55, Zhongguancun donglu, 100190 Beijing, People’s Republic of China University of Chinese Academy of Sciences, 19A, Yuquan lu, 100049 Beijing, People’s Republic of China ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 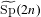$\widetilde{\text{Sp}}(2n)$ be the metaplectic covering of 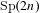$\text{Sp}(2n)$ over a local field of characteristic zero. The core of the theory of endoscopy for 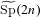$\widetilde{\text{Sp}}(2n)$ is the geometric transfer of orbital integrals to its elliptic endoscopic groups. The dual of this map, called the spectral transfer, is expected to yield endoscopic character relations which should reveal the internal structure of $L$-packets. As a first step, we characterize the image of the collective geometric transfer in the non-archimedean case, then reduce the spectral transfer to the case of cuspidal test functions by using a simple stable trace formula. In the archimedean case, we establish the character relations and determine the spectral transfer factors by rephrasing the works by Adams and Renard.

Keywords

endoscopycharacter relationmetaplectic groupArthur–Selberg trace formula

MSC classification

Primary: 22E50: Representations of Lie and linear algebraic groups over local fields
Secondary: 11F72: Spectral theory; Selberg trace formula
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 18 , Issue 1 , January 2019 , pp. 25 - 123
Copyright
© Cambridge University Press 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adams, J., Lifting of characters on orthogonal and metaplectic groups, Duke Math. J. 92(1) (1998), 129–178.Google Scholar
Arthur, J., The invariant trace formula. II. Global theory, J. Amer. Math. Soc. 1(3) (1988), 501–554.Google Scholar
Arthur, J., Unipotent automorphic representations: conjectures, Astérisque (171–172) (1989), 13–71. Orbites unipotentes et représentations, II. Astérisque, 171–172.Google Scholar
Arthur, J., On elliptic tempered characters, Acta Math. 171(1) (1993), 73–138.Google Scholar
Arthur, J., On the Fourier transforms of weighted orbital integrals, J. Reine Angew. Math. 452 (1994), 163–217.Google Scholar
Arthur, J., On local character relations, Selecta Math. (N.S.) 2(4) (1996), 501–579.Google Scholar
Arthur, J., A stable trace formula. I. General expansions, J. Inst. Math. Jussieu 1(2) (2002), 175–277.Google Scholar
Arthur, J., Germ expansions for real groups. http://www.math.toronto.edu/arthur, 2004.Google Scholar
Arthur, J., A note on L-packets, Pure Appl. Math. Q. 2(1) (2006), 199–217. Special Issue: In honor of John H. Coates. Part 1.Google Scholar
Arthur, J., The Endoscopic Classification of Representations: Orthogonal and Symplectic Groups, American Mathematical Society Colloquium Publications, Volume 61, (American Mathematical Society, Providence, RI, 2013).Google Scholar
Barbasch, D. and Moy, A., A new proof of the Howe conjecture, J. Amer. Math. Soc. 13(3) (2000), 639–650 (electronic).Google Scholar
Borel, A., Automorphic L-functions, in Automorphic Forms, Representations and L-Functions (Proc. Sympos. Pure Math., Oregon State University, Corvallis, OR, 1977), Part 2, Proceedings of Symposia in Pure Mathematics, Volume XXXIII, pp. 27–61 (American Mathematical Society, Providence, R.I., 1979).Google Scholar
Borovoi, M., Abelian Galois cohomology of reductive groups, Mem. Amer. Math. Soc. 132(626) (1998), viii+50.Google Scholar
Bouaziz, A., Intégrales orbitales sur les groupes de Lie réductifs, Ann. Sci. Éc. Norm. Supér. (4) 27(5) (1994), 573–609.Google Scholar
Chaudouard, P.-H., Le transfert lisse des intégrales orbitales d’après Waldspurger, in On the Stabilization of the Trace Formula, Stabilization of the Trace Formula, Shimura Varieties, and Arithmetic Applications, Volume 1, pp. 145–180 (International Press, Somerville, MA, 2011).Google Scholar
Clozel, L. and Delorme, P., Le théorème de Paley–Wiener invariant pour les groupes de Lie réductifs, Invent. Math. 77(3) (1984), 427–453.Google Scholar
Clozel, L., Harris, M., Labesse, J.-P. and Ngô, B.-C. (Eds.) On the Stabilization of the Trace Formula, Stabilization of the Trace Formula, Shimura Varieties, and Arithmetic Applications, Volume 1 (International Press, Somerville, MA, 2011).Google Scholar
Duflo, M., Représentations irréductibles des groupes semi-simples complexes, in Analyse harmonique sur les groupes de Lie (Sém., Nancy–Strasbourg, 1973–75), Lecture Notes in Mathematica, Volume 497, pp. 26–88 (Springer, Berlin, 1975).Google Scholar
Finis, T., Lapid, E. and Müller, W., On the spectral side of Arthur’s trace formula—absolute convergence, Ann. of Math. (2) 174(1) (2011), 173–195.Google Scholar
Henniart, G., Une preuve simple des conjectures de Langlands pour GL(n) sur un corps p-adique, Invent. Math. 139(2) (2000), 439–455.Google Scholar
Hiraga, K. and Saito, H., On L-packets for inner forms of SL n , Mem. Amer. Math. Soc. 215(1013) (2012), vi+97.Google Scholar
Knapp, A. W. and Vogan, D. A. Jr., Cohomological Induction and Unitary Representations, Princeton Mathematical Series, Volume 45 (Princeton University Press, Princeton, NJ, 1995).Google Scholar
Knapp, A. W. and Zuckerman, G. J., Classification of irreducible tempered representations of semisimple groups, Ann. of Math. (2) 116(2) (1982), 389–455.Google Scholar
Knapp, A. W. and Zuckerman, G. J., Classification of irreducible tempered representations of semisimple groups. II, Ann. of Math. (2) 116(3) (1982), 457–501.Google Scholar
Kottwitz, R. E., Rational conjugacy classes in reductive groups, Duke Math. J. 49(4) (1982), 785–806.Google Scholar
Kottwitz, R. E., Stable trace formula: elliptic singular terms, Math. Ann. 275(3) (1986), 365–399.Google Scholar
Kottwitz, R. E. and Rogawski, J. D., The distributions in the invariant trace formula are supported on characters, Canad. J. Math. 52(4) (2000), 804–814.Google Scholar
Kottwitz, R. E. and Shelstad, D., Foundations of twisted endoscopy, Astérisque 255 (1999), vi+190.Google Scholar
Labesse, J.-P., Cohomologie, stabilisation et changement de base, Astérisque 257 (1999), 161. Appendix A by Laurent Clozel and Labesse, and Appendix B by Lawrence Breen.Google Scholar
Li, W.-W., Transfert d’intégrales orbitales pour le groupe métaplectique, Compos. Math. 147(2) (2011), 524–590.Google Scholar
Li, W.-W., La formule des traces pour les revêtements de groupes réductifs connexes. II. Analyse harmonique locale, Ann. Sci. Éc. Norm. Supér. (4) 45(5) (2012), 787–859.Google Scholar
Li, W.-W., Le lemme fondamental pondéré pour le groupe métaplectique, Canad. J. Math. 64(3) (2012), 497–543.Google Scholar
Li, W.-W., La formule des traces pour les revêtements de groupes réductifs connexes III: Le développement spectral fin, Math. Ann. 356(3) (2013), 1029–1064.Google Scholar
Li, W.-W., La formule des traces pour les revêtements de groupes réductifs connexes. I. Le développement géométrique fin, J. Reine Angew. Math. 686 (2014), 37–109.Google Scholar
Li, W.-W., La formule des traces pour les revêtements de groupes réductifs connexes. IV. Distributions invariantes, Ann. Inst. Fourier 64(6) (2014), 2379–2448.Google Scholar
Li, W.-W., La formule des traces stable pour le groupe métaplectique: les termes elliptiques, Invent. Math. 202(2) (2015), 743–838.Google Scholar
Mœglin, C., Vignéras, M.-F. and Waldspurger, J.-L., Correspondances de Howe sur un corps p-adique, Lecture Notes in Mathematics, Volume 1291 (Springer-Verlag, Berlin, 1987).Google Scholar
Renard, D., Transfert d’intégrales orbitales entre Mp(2n, R) et SO(n + 1, n), Duke Math. J. 95(2) (1998), 425–450.Google Scholar
Renard, D., Endoscopy for Mp(2n, R), Amer. J. Math. 121(6) (1999), 1215–1243.Google Scholar
Shelstad, D., Characters and inner forms of a quasi-split group over R , Compos. Math. 39(1) (1979), 11–45.Google Scholar
Shelstad, D., L-indistinguishability for real groups, Math. Ann. 259(3) (1982), 385–430.Google Scholar
Shelstad, D., Tempered endoscopy for real groups. III. Inversion of transfer and L-packet structure, Represent. Theory 12 (2008), 369–402.Google Scholar
Shelstad, D., Tempered endoscopy for real groups. II. Spectral transfer factors, in Automorphic Forms and the Langlands Program, Advanced Lectures in Mathematics (ALM), Volume 9, pp. 236–276 (International Press, Somerville, MA, 2010).Google Scholar
Trèves, F., Topological Vector Spaces, Distributions and Kernels (Academic Press, New York, 1967).Google Scholar
Vignéras, M.-F., Caractérisation des intégrales orbitales sur un groupe réductif p-adique, J. Fac. Sci. Univ. Tokyo Sect. IA Math. 28(3) (1981), 945–961.Google Scholar
Vogan, D. A. Jr., The algebraic structure of the representation of semisimple Lie groups. I, Ann. of Math. (2) 109(1) (1979), 1–60.Google Scholar
Waldspurger, J.-L., Représentation métaplectique et conjectures de Howe, Astérisque 152–153(3) (1988), 85–99. 1987. Séminaire Bourbaki, Vol. 1986/87.Google Scholar
Waldspurger, J.-L., Correspondances de Shimura et quaternions, Forum Math. 3(3) (1991), 219–307.Google Scholar
Waldspurger, J.-L., L’endoscopie tordue n’est pas si tordue, Mem. Amer. Math. Soc. 194(908) (2008), x+261.Google Scholar
Waldspurger, J.-L., Préparation à la stabilisation de la formule des traces tordue IV: transfert spectral archimédien, 2013. http://www.math.jussieu.fr/∼waldspur.Google Scholar
Waldspurger, J.-L., Stabilisation de la formule des traces tordue I: endoscopie tordue sur un corps local, 2014. http://www.math.jussieu.fr/∼waldspur.Google Scholar