Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-23T11:13:53.849Z Has data issue: false hasContentIssue false
  • English
  • Français

RECOVERY OF ZEROTH ORDER COEFFICIENTS IN NON-LINEAR WAVE EQUATIONS

Part of: Miscellaneous topics - Partial differential equations

Published online by Cambridge University Press:  18 September 2020

Ali Feizmohammadi Open the ORCID record for Ali Feizmohammadi [Opens in a new window]  and
Lauri Oksanen Open the ORCID record for Lauri Oksanen [Opens in a new window]
Ali Feizmohammadi
Affiliation:
Department of Mathematics, University College London, Gower Street, LondonWC1E 6BT, UK ([email protected]; [email protected])
Lauri Oksanen
Affiliation:
Department of Mathematics, University College London, Gower Street, LondonWC1E 6BT, UK ([email protected]; [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper is concerned with the resolution of an inverse problem related to the recovery of a function $V$ from the source to solution map of the semi-linear equation 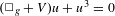$(\Box _{g}+V)u+u^{3}=0$ on a globally hyperbolic Lorentzian manifold 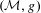$({\mathcal{M}},g)$. We first study the simpler model problem, where 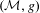$({\mathcal{M}},g)$ is the Minkowski space, and prove the unique recovery of $V$ through the use of geometric optics and a three-fold wave interaction arising from the cubic non-linearity. Subsequently, the result is generalized to globally hyperbolic Lorentzian manifolds by using Gaussian beams.

Keywords

Inverse problemsnon-linear wave equationsGaussian beamsLorentzian manifolds

MSC classification

Primary: 35R30: Inverse problems
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 21 , Issue 2 , March 2022 , pp. 367 - 393
Check for updates
Copyright
© The Author(s) 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alinhac, S., Non-unicité du problème de Cauchy, Ann. of Math. (2) 117(1) (1983), 77–108.10.2307/2006972CrossRefGoogle Scholar
Babich, V. and Ulin, V., The complex space-time ray method and quasi-photons, Zap. Nauch Semin. LOMI 117 (1981), 5–12. (Russian).Google Scholar
Belishev, M. and Katchalov, A., Boundary control and quasi-photons in the problem of a Riemannian manifold reconstruction via its dynamical data, Zap. Nauch. Semin. POMI 203 (1992), 21–50. (Russian).Google Scholar
Bernal, A. N. and Sánchez, M., Globally hyperbolic spacetimes can be defined as ‘causal’ instead of ‘strongly causal’, Class. Quantum Grav. 24 (2007), 745–749.10.1088/0264-9381/24/3/N01CrossRefGoogle Scholar
Chen, X., Lassas, M., Oksanen, L. and Paternain, G., Detection of Hermitian connections in wave equations with cubic non-linearity, preprint, 2019, arXiv:1902.05711.Google Scholar
Dos Santos Ferreira, D., Kurylev, S., Lassas, M. and Salo, M., The Calderón problem in transversally anisotropic geometries, J. Eur. Math. Soc. 18(11) (2016), 2579–2626.CrossRefGoogle Scholar
Eskin, G., Inverse hyperbolic problems with time-dependent coefficients, Commun. Partial Differential Equations 32(10–12) (2007), 1737–1758.CrossRefGoogle Scholar
Feizmohammadi, A., Ilmavirta, J., Kian, Y. and Oksanen, L., Recovery of time dependent coefficients from boundary data for hyperbolic equations, J. Spectral Theory (2020), to appear.CrossRefGoogle Scholar
de Hoop, M., Uhlmann, G. and Wang, Y., Nonlinear interaction of waves in elastodynamics and an inverse problem, Math. Ann. 376(1–2) (2020), 765–795.CrossRefGoogle Scholar
Hörmander, L., The Analysis of Linear Partial Differential Operators, Volume I (Springer, Berlin, Heidelberg, 1990).Google Scholar
Katchalov, A. and Kurylev, Y., Multidimensional inverse problem with incomplete boundary spectral data, Comm. Partial Differential Equations 23 (1998), 55–95.CrossRefGoogle Scholar
Katchalov, A., Kurylev, Y. and Lassas, M., Inverse Boundary Spectral Problems, CRC Monographs and Surveys in Pure and Applied Mathematics, Volume 123 (Chapman & Hall/CRC, Boca Raton, FL, 2001).CrossRefGoogle Scholar
Kian, Y., On the determination of nonlinear terms appearing in semilinear hyperbolic equations, preprint, 2018, arXiv:1807.02165.Google Scholar
Kurylev, Y., Lassas, M., Oksanen, L. and Uhlmann, G., Inverse problem for Einstein-scalar field equations, preprint, 2014, arXiv:1406.4776.Google Scholar
Kurylev, Y., Lassas, M. and Uhlmann, G., Inverse problems for Lorentzian manifolds and non-linear hyperbolic equations, Invent. Math. 212(3) (2018), 781–857.CrossRefGoogle Scholar
Kurylev, Y., Lassas, M. and Uhlmann, G., Inverse problems in spacetime I: Inverse problems for Einstein equations, Extended preprint version, 2014, arXiv:1405.4503.Google Scholar
Lassas, M., Uhlmann, G. and Wang, Y., Inverse problems for semilinear wave equations on Lorentzian manifolds, Commun. Math. Phys. 360 (2018), 555–609.10.1007/s00220-018-3135-7CrossRefGoogle Scholar
Lassas, M., Uhlmann, G. and Wang, Y., Determination of vacuum space-times from the Einstein–Maxwell equations, arXiv, preprint.Google Scholar
Nakamura, G. and Vashisth, M., Inverse boundary value problem for non-linear hyperbolic partial differential equations, preprint, 2017, arXiv:1712.09945.Google Scholar
O’Neill, B., Semi–Riemannian Geometry with Applications to Relativity, Pure and Applied Mathematics, Volume 103 (Academic Press, 1983).Google Scholar
Ralston, J., Gaussian beams and the propagation of singularities, in Studies in Partial Differential Equations, MAA Studies in Mathematics, Volume 23, pp. 206–248 (Math. Assoc. America, Washington, DC, 1983).Google Scholar
Stefanov, P., Uniqueness of the multi-dimensional inverse scattering problem for time dependent potentials, Math. Z. 201(4) (1989), 541–559.CrossRefGoogle Scholar
Tataru, D., Unique continuation for solutions to PDE’s; between Hörmander’s theorem and Holmgren’s theorem, Comm. Partial Differential Equations 20(5–6) (1995), 855–884.Google Scholar
Uhlmann, G. and Wang, Y., Determination of space-time structures from gravitational perturbations, Comm. Pure Appl. Math. doi:10.1002/cpa.21882.CrossRefGoogle Scholar
Wang, Y. and Zhou, T., Inverse problems for quadratic derivative nonlinear wave equations, Comm. Partial Differential Equations 44(11) (2019), 1140–1158.10.1080/03605302.2019.1612908CrossRefGoogle Scholar