Article contents
ON FUNDAMENTAL FOURIER COEFFICIENTS OF SIEGEL MODULAR FORMS
Published online by Cambridge University Press: 03 March 2021
Abstract
We prove that if F is a nonzero (possibly noncuspidal) vector-valued Siegel modular form of any degree, then it has infinitely many nonzero Fourier coefficients which are indexed by half-integral matrices having odd, square-free (and thus fundamental) discriminant. The proof uses an induction argument in the setting of vector-valued modular forms. Further, as an application of a variant of our result and complementing the work of A. Pollack, we show how to obtain an unconditional proof of the functional equation of the spinor L-function of a holomorphic cuspidal Siegel eigenform of degree
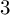 $3$
and level
$3$
and level
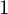 $1$
.
$1$
.
MSC classification
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 21 , Issue 6 , November 2022 , pp. 2001 - 2041
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
- 3
- Cited by



