Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-23T01:55:07.707Z Has data issue: false hasContentIssue false
  • English
  • Français

ON ANNULAR MAPS OF THE TORUS AND SUBLINEAR DIFFUSION

Part of: Topological dynamics Low-dimensional dynamical systems

Published online by Cambridge University Press:  23 June 2016

Pablo Dávalos
Pablo Dávalos*
Affiliation:
Instituto Tecnológico y de Estudios Superiores de Occidente, Periférico Sur Manuel Gómez Morín 8585, C.P. 45604, Tlaquepaque, Jalisco, México ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

A classical article by Misiurewicz and Ziemian (J. Lond. Math. Soc.40(2) (1989), 490–506) classifies the elements in Homeo$_{0}(\mathbf{T}^{2})$ by their rotation set $\unicode[STIX]{x1D70C}$, according to wether $\unicode[STIX]{x1D70C}$ is a point, a segment or a set with nonempty interior. A recent classification of nonwandering elements in Homeo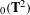$_{0}(\mathbf{T}^{2})$ by Koropecki and Tal was given in (Invent. Math.196 (2014), 339–381), according to the intrinsic underlying ambient space where the dynamics takes place: planar, annular and strictly toral maps. We study the link between these two classifications, showing that, even abroad the nonwandering setting, annular maps are characterized by rotation sets which are rational segments. Also, we obtain information on the sublinear diffusion of orbits in the—not very well understood—case that $\unicode[STIX]{x1D70C}$ has nonempty interior.

Keywords

torus homeomorphismsrotation settopological dynamics

MSC classification

Primary: 37B99: None of the above, but in this section 37E45: Rotation numbers and vectors 37E30: Homeomorphisms and diffeomorphisms of planes and surfaces
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 17 , Issue 4 , September 2018 , pp. 913 - 978
Copyright
© Cambridge University Press 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This work was partially supported by FAPESP-Brasil grant 2011/14122-5 and ITESO-México.

References

Atkinson, G., Recurrence of cocycles and random walks, J. Lond. Math. Soc. 13(2) (1976), 486–488.Google Scholar
Béguin, F., Crovisier, S., Jäger, T. and Le Roux, F., Denjoy constructions for fibered homeomorphisms of the torus, Trans. Amer. Math. Soc. 361(11) (2009), 5851–5883.Google Scholar
Boyland, P., de Carvalho, A. and Hall, T., New rotation sets in a family of torus homeomorphisms, Invent. Math. 204 (2016), 895–937.Google Scholar
Cairns, S., An elementary proof of the Jordan–Schoenflies theorem, Proc. Amer. Math. Soc. 91(2) (1951), 860–867.Google Scholar
Dávalos, P., On torus homeomorphisms whose rotation set is an interval, Math. Z. 275 (2013), 1005–1045.Google Scholar
Daverman, R. J., Decompositions of Manifolds, vol. 124 (Academic Press Inc., Orlando, FL, 1986).Google Scholar
Fayad, B., Weak mixing for reparametrized linear flows on the torus, Ergod. Th. & Dynam. Sys. 22 (2002), 187–201.Google Scholar
Franks, J., Realizing rotation vectors for torus homeomorphisms, Trans. Amer. Math. Soc. 311(1) (1989), 107–115.Google Scholar
Franks, J., The rotation set and periodic points for torus homeomorphisms, in Dynamical Systems & Chaos (ed. Aoki, Shiraiwa and Takahashi), pp. 41–48 (World Scientific, Singapore, 1995).Google Scholar
Franks, J. and Misiurewicz, M., Rotation sets of toral flows, Proc. Amer. Math. Soc. 109(1) (1990), 243–249.Google Scholar
Furstenberg, H., Strict ergodicity and transformation of the torus, Amer. J. Math. 83 (1961), 573–601.Google Scholar
Guelmanm, N., Koropecki, A. and Tal, F. A., A characterization of annularity for area preserving toral homeomorphisms, Math. Z. 276 (2014), 673–689.Google Scholar
Gutiérrez, C., Smoothing continuous flows and the converse of Denjoy–Schwartz theorem, An. Acad. Brasil. Ciênc. 51(4) (1979), 581–589.Google Scholar
Handel, M., Periodic point free homeomorphisms of T2 , Proc. Amer. Math. Soc. 107(2) (1989), 511–515.Google Scholar
Handel, M., The rotation set of a homeomorphism of the annulus is closed, Comm. Math. Phys. 127 (1990), 339–349.Google Scholar
Hirsch, M. W. and Smale, S., Differential Equations, Dynamical Systems, and Linear Algebra (Academic Press Inc., New York, 1974).Google Scholar
Jäger, T., The concept of bounded mean motion for toral homeomorphisms, Dyn. Syst. 24(3) (2009), 277–297.Google Scholar
Jäger, T., Linearisation of conservative toral homeomorphisms, Invent. Math. 176(3) (2009), 601–616.Google Scholar
Jaulent, O., Existence d’un feuilletage positivement transverse à un homeomorphism de surface, Annales de l’insitute Fourier 64 (2014), 1441–1476.Google Scholar
Kocksard, A. and Koropecki, A., Free curves and periodic points for torus homeomorphisms, Ergod. Th. & Dynam. Sys. 28 (2008), 1895–1915.Google Scholar
Kocksard, A. and Koropecki, A., A mixing-like property and inexistence of invariant foliations for minimal diffeomorphisms of the 2-torus, Proc. Amer. Math. Soc. 137(10) (2009), 3379–3386.Google Scholar
Koropecki, A. and Tal, F. A., Area preserving irrotational diffeomorphisms of the torus with sublinear diffusion, Proc. Amer. Math. Soc. 142(10) (2014), 3483–3490.Google Scholar
Koropecki, A. and Tal, F. A., Bounded and unbounded behaviour for area-preserving rational pseudorotations, Proc. Lond. Math. Soc. 109 (2014), 785–822.Google Scholar
Koropecki, A. and Tal, F. A., Strictly toral dynamics, Invent. Math. 196 (2014), 339–381.Google Scholar
Kwapisz, J., Every convex polygon with rational vertices is a rotation set, Ergod. Th. & Dynam. Sys. 12 (1992), 333–339.Google Scholar
Kwapisz, J., A toral diffeomorphism with a nonpolygonal rotation set, Nonlinearity 8(4) (1995), 461–476.Google Scholar
Le Calvez, P., Une version feuilletée équivariante du théorème de translation de Brouwer, Publ. Math. Inst. Hautes Études Sci. 102(1) (2005), 1–98.Google Scholar
Llibre, J. and Mackay, R. S., Rotation vectors and entropy for torus homeomorphisms isotopic to the identity, Ergod. Th. & Dynam. Sys. 11 (1991), 115–128.Google Scholar
Matsumoto, S., Rotation sets of surface homeomorphisms, Bol. Soc. Bras. Mat. 28(1) (1997), 89–101.Google Scholar
Misiurewicz, M. and Ziemian, K., Rotation set for maps of tori, J. Lond. Math. Soc. 40(2) (1989), 490–506.Google Scholar
Misiurewicz, M. and Ziemian, K., Rotation sets and ergodic measures for torus homeomorphisms, Fund. Math. 137(1) (1991), 45–52.Google Scholar
Moser, J., On the volume elements on a manifold, Trans. Amer. Math. Soc. 120(2) (1965), 286–294.Google Scholar
Nemitskii, V. V. and Stepanov, V. V., Qualitative Theory of Differential Equations (Dover Publications Inc., New York, 1989).Google Scholar
Poincaré, H., Oeuvres Complètes (Gauthier-Villars, Paris, 1952).Google Scholar
Pollicott, M., Rotation sets for homeomorphisms and homology, Trans. Amer. Math. Soc. 331(2) (1992), 881–894.Google Scholar
Schwartzmann, S., Assymptotic cycles, Ann. of Math. (2) 66(2) (1957), 270–284.Google Scholar
Solntzev, G., On the asymptotic behaviour of integral curves of a system of differential equations, Bull. Acad. Sci. URSS. Sér. Math. [Izv. Akad. Nauk SSSR] 9 (1945), 233–240.Google Scholar
Tal, F. A. and Addas-Zanata, S., On periodic points of area preserving homeomorphisms, Far East J. Dyn. Syst. 9(3) (2007), 371–378.Google Scholar
Whitney, H., Regular families of curves, Ann. of Math. (2) 34(2) (1933), 244–270.Google Scholar
Whitney, H., On regular families of curves, Bull. Amer. Math. Soc. 47 (1941), 145–147.Google Scholar