Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-03T00:15:05.479Z Has data issue: false hasContentIssue false
  • English
  • Français

Nearly ordinary Galois deformations over arbitrary number fields

Part of: Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  04 April 2008

Frank Calegari  and
Barry Mazur
Frank Calegari
Affiliation:
Department of Mathematics, Northwestern University, Lunt Hall, 2033 Sheridan Road, Evanston, IL 60208-2730, USA ([email protected])
Barry Mazur
Affiliation:
Department of Mathematics, Harvard University, One Oxford Street, Cambridge, MA 02138, USA ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

Let K be an arbitrary number field, and let ρ : Gal(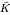/K) → GL2(E) be a nearly ordinary irreducible geometric Galois representation. In this paper, we study the nearly ordinary deformations of ρ. When K is totally real and ρ is modular, results of Hida imply that the nearly ordinary deformation space associated to ρ contains a Zariski dense set of points corresponding to ‘automorphic’ Galois representations. We conjecture that if K is not totally real, then this is never the case, except in three exceptional cases, corresponding to: (1) ‘base change’, (2) ‘CM’ forms, and (3) ‘even’ representations. The latter case conjecturally can only occur if the image of ρ is finite. Our results come in two flavours. First, we prove a general result for Artin representations, conditional on a strengthening of the Leopoldt Conjecture. Second, when K is an imaginary quadratic field, we prove an unconditional result that implies the existence of ‘many’ positive-dimensional components (of certain deformation spaces) that do not contain infinitely many classical points. Also included are some speculative remarks about ‘p-adic functoriality’, as well as some remarks on how our methods should apply to n-dimensional representations of Gal(/ℚ) when n > 2.

Keywords

Galois deformationsautomorphic formsHida familieseigenvarietiesp-adic modular forms

MSC classification

Secondary: 11F75: Cohomology of arithmetic groups 11F80: Galois representations
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 8 , Issue 1 , January 2009 , pp. 99 - 177
Copyright
Copyright © Cambridge University Press 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Ash, A., Galois representations attached to mod p cohomology of GL(n, ℤ), Duke Math. J. 65(2) (1992), 235–255.CrossRefGoogle Scholar
2.Ash, A. and Stevens, G., p-adic deformations of arithmetic cohomology, draft (available at www2.bc.edu/~ashav/).Google Scholar
3.Bellaïche, J. and Chenevier, G., p-Adic families of Galois representations and higher rank Selmer groups, to be published in Astérisque.Google Scholar
4.Brumer, A., On the units of algebraic number fields, Mathematika 14 (1967), 121–124.CrossRefGoogle Scholar
5.Buzzard, K., Dickinson, M., Shepherd-Barron, N. and Taylor, R., On icosahedral Artin representations, Duke Math. J. 109(2) (2001), 283–318.CrossRefGoogle Scholar
6.Coleman, R. and Mazur, B., The eigencurve, in Galois Representations in Algebraic Geometry, Durham, 1996, London Mathematical Society Lecture Note Series, Volume 254, pp. 1–113 (Cambridge University Press, 1998).Google Scholar
7.Cremona, J., Hyperbolic tessellations, modular symbols, and elliptic curves over complex quadratic fields, Compositio Math. 51(3) (1984), 275–324.Google Scholar
8.Elstrodt, J., Grunewald, F. and Mennicke, J., On the group PSL2(ℤ[i]), in Number Theory Days, Exeter, 1980, London Mathematical Society Lecture Note Series, Volume 56, pp. 255–283 (Cambridge University Press, 1982).Google Scholar
9.Feit, W., The current situation in the theory of finite simple groups, in Actes du Congrès International des Mathématiciens, Nice, 1970, Tome 1, pp. 55–93 (Gauthier-Villars, Paris, 1971).Google Scholar
10.Gouvêa, F., Deformations of Galois representations, in Arithmetic Algebraic Geometry, IAS/Park City, UT, 1999, Mathematics Series, Volume 9, pp. 233–406 (American Mathematical Society, Providence, RI, 2001).Google Scholar
11.Grunewald, F., Helling, F. and Mennicke, J., SL2 over complex quadratic number fields, I, Algebra Logika 17(5) (1978), 512–580, 622.CrossRefGoogle Scholar
12.Harder, G., Eisenstein cohomology of arithmetic groups: the case GL2, Invent. Math. 89(1) (1987), 37–118.CrossRefGoogle Scholar
13.Harris, M., Soudry, D. and Taylor, R., l-adic representations associated to modular forms over imaginary quadratic fields, I, Lifting to GSp4(ℚ), Invent. Math. 112(2) (1993), 377–411.CrossRefGoogle Scholar
14.Hida, H., Galois representations into GL2(ℤp[[X]]) attached to ordinary cusp forms, Invent. Math. 85(3) (1986), 545–613.CrossRefGoogle Scholar
15.Hida, H., On p-adic Hecke algebras for GL2 over totally real fields, Annals Math. (2) 128(2) (1988), 295–384.CrossRefGoogle Scholar
16.Hida, H., On nearly ordinary Hecke algebras for GL(2) over totally real fields, in Algebraic number theory, Advanced Studies in Pure Mathematics, Volume 17, pp. 139–169 (Academic Press, Boston, MA, 1989).Google Scholar
17.Hida, H., p-ordinary cohomology groups for SL(2) over number fields, Duke Math. J. 69(2) (1993), 259–314.CrossRefGoogle Scholar
18.Hida, H., p-adic ordinary Hecke algebras for GL(2), Annales Inst. Fourier 44(5) (1994), 1289–1322.CrossRefGoogle Scholar
19.Hida, H., p-adic automorphic forms on reductive group, in Automorphic forms, Volume I, Astérisque 298 (2005), 147–254.Google Scholar
20.Kisin, M., Overconvergent modular forms and the Fontaine–Mazur conjecture, Invent. Math. 153(2) (2003), 373–454.CrossRefGoogle Scholar
21.Kudla, S., Rallis, S. and Soudry, D., On the degree 5 L-function for Sp(2), Invent. Math. 107(3) (1992), 483–541.CrossRefGoogle Scholar
22.Lang, S., Algebraic number theory, 2nd edn, Graduate Texts in Mathematics, Volume 110 (Springer, 1994).CrossRefGoogle Scholar
23.Laurent, M., Rang p-adique d'unité et actions de groupes, J. Reine Angew. Math. 399 (1989), 81–108.Google Scholar
24.Mahler, K., Ein beweis der Transzendence der p-adische Exponentialfunktion, J. Mathematik 166 (1932), 61–66.Google Scholar
25.Mahler, K., Über die transzendente p-adische Zahlen, Compositio Math. 2 (1935), 259–275 (a correction was published in the same journal in 1948).Google Scholar
26.Matzat, B. H., Zwei Aspekte konstruktiver Galoistheorie, J. Alg. 96(2) (1985), 499–531.CrossRefGoogle Scholar
27.Mazur, B., An introduction to the deformation theory of Galois representations, in Modular Forms and Fermat's Last Theorem, Boston, MA, 1995, pp. 243–311 (Springer, 1997).Google Scholar
28.Mazur, B., The theme of p-adic variations, in Mathematics: frontiers and perspectives, pp. 433–459 (American Mathematical Society, Providence, RI, 2000).Google Scholar
29.Milne, J. S., Arithmetic duality theorems (Academic Press, 1986).Google Scholar
30.Mokrane, A. and Tilouine, J., Cohomology of Siegel varieties with p-adic integral coef-ficients and applications, Astérisque 280 (2002), 1–95.Google Scholar
31.Priplata, C., Inaugural dissertation, Mathematisch–Naturwissenschaftlichen Fakultät der Heinrich–Heine–Universität Düsseldorf.Google Scholar
32.Ramakrishna, R., Lifting Galois representations, Invent. Math. 138(3) (1999), 537–562.CrossRefGoogle Scholar
33.Ramakrishna, R., Deforming Galois representations and the conjectures of Serre and Fontaine–Mazur, Annals Math. 156 (2002), 115–154.CrossRefGoogle Scholar
34.Roy, D., Matrices whose coefficients are linear forms in logarithms, J. Number Theory 41 (1992), 22–47.CrossRefGoogle Scholar
35.Roy, D., On the v-adic independence of algebraic numbers, in Advances in Number Theory, Kingston, ON, 1991, pp. 441–451, Oxford Science Publications (Oxford University Press, 1993).Google Scholar
36.Sen, S., The analytic variation of p-adic Hodge structure, Annals Math. 127(3) (1988), 647–661.CrossRefGoogle Scholar
37.Serre, J.-P., Sur les représentations modulaires de degré 2 de Gal(/ℚ), Duke Math. J. 54 (1987), 179–230.CrossRefGoogle Scholar
38.Taylor, R., On congruences between modular forms, PhD thesis, Princeton University (1988; available at http://abel.math.harvard.edu/~rtaylor/).Google Scholar
39.Taylor, R., ℓ-adic representations associated to modular forms over imaginary quadratic fields, II, Invent. Math. 116 (1994), 619–643.CrossRefGoogle Scholar
40.Urban, E., Sur les représentations p-adiques associée aux représentations cuspidales de GSp4/ℚ, in Formes automorphes, II, Le cas du groupe GSp(4), Astérisque 302 (2005), 151–176.Google Scholar