Article contents
LIE ALGEBROIDS AS 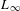 $L_{\infty }$ SPACES
$L_{\infty }$ SPACES
Published online by Cambridge University Press: 13 February 2018
Abstract
In this paper, we relate Lie algebroids to Costello’s version of derived geometry. For instance, we show that each Lie algebroid – and the natural generalization to dg Lie algebroids – provides an (essentially unique) 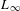 $L_{\infty }$ space. More precisely, we construct a faithful functor from the category of Lie algebroids to the category of
$L_{\infty }$ space. More precisely, we construct a faithful functor from the category of Lie algebroids to the category of  $L_{\infty }$ spaces. Then we show that for each Lie algebroid
$L_{\infty }$ spaces. Then we show that for each Lie algebroid  $L$, there is a fully faithful functor from the category of representations up to homotopy of
$L$, there is a fully faithful functor from the category of representations up to homotopy of  $L$ to the category of vector bundles over the associated
$L$ to the category of vector bundles over the associated 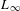 $L_{\infty }$ space. Indeed, this functor sends the adjoint complex of
$L_{\infty }$ space. Indeed, this functor sends the adjoint complex of  $L$ to the tangent bundle of the
$L$ to the tangent bundle of the  $L_{\infty }$ space. Finally, we show that a shifted symplectic structure on a dg Lie algebroid produces a shifted symplectic structure on the associated
$L_{\infty }$ space. Finally, we show that a shifted symplectic structure on a dg Lie algebroid produces a shifted symplectic structure on the associated 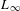 $L_{\infty }$ space.
$L_{\infty }$ space.
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 19 , Issue 2 , March 2020 , pp. 487 - 535
- Copyright
- © Cambridge University Press 2018
Footnotes
The first author was partially supported by the National Science Foundation under Award DMS-1309118. The second author was partially supported as a postdoctoral fellow by the National Science Foundation under Award DMS-1204826.
References
- 3
- Cited by


