Article contents
CENTRAL LIMIT THEOREM FOR THE MODULUS OF CONTINUITY OF AVERAGES OF OBSERVABLES ON TRANSVERSAL FAMILIES OF PIECEWISE EXPANDING UNIMODAL MAPS
Published online by Cambridge University Press: 13 July 2016
Abstract
Consider a  $C^{2}$ family of mixing
$C^{2}$ family of mixing 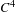 $C^{4}$ piecewise expanding unimodal maps
$C^{4}$ piecewise expanding unimodal maps 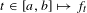 $t\in [a,b]\mapsto f_{t}$, with a critical point
$t\in [a,b]\mapsto f_{t}$, with a critical point  $c$, that is transversal to the topological classes of such maps. Given a Lipchitz observable
$c$, that is transversal to the topological classes of such maps. Given a Lipchitz observable  $\unicode[STIX]{x1D719}$ consider the function
$\unicode[STIX]{x1D719}$ consider the function 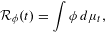 $$\begin{eqnarray}{\mathcal{R}}_{\unicode[STIX]{x1D719}}(t)=\int \unicode[STIX]{x1D719}\,d\unicode[STIX]{x1D707}_{t},\end{eqnarray}$$
$$\begin{eqnarray}{\mathcal{R}}_{\unicode[STIX]{x1D719}}(t)=\int \unicode[STIX]{x1D719}\,d\unicode[STIX]{x1D707}_{t},\end{eqnarray}$$ $\unicode[STIX]{x1D707}_{t}$ is the unique absolutely continuous invariant probability of
$\unicode[STIX]{x1D707}_{t}$ is the unique absolutely continuous invariant probability of  $f_{t}$. Suppose that
$f_{t}$. Suppose that  $\unicode[STIX]{x1D70E}_{t}>0$ for every
$\unicode[STIX]{x1D70E}_{t}>0$ for every 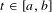 $t\in [a,b]$, where
$t\in [a,b]$, where  $$\begin{eqnarray}\unicode[STIX]{x1D70E}_{t}^{2}=\unicode[STIX]{x1D70E}_{t}^{2}(\unicode[STIX]{x1D719})=\lim _{n\rightarrow \infty }\int \left(\frac{\mathop{\sum }_{j=0}^{n-1}\left(\unicode[STIX]{x1D719}\circ f_{t}^{j}-\int \unicode[STIX]{x1D719}\,d\unicode[STIX]{x1D707}_{t}\right)}{\sqrt{n}}\right)^{2}\,d\unicode[STIX]{x1D707}_{t}.\end{eqnarray}$$
$$\begin{eqnarray}\unicode[STIX]{x1D70E}_{t}^{2}=\unicode[STIX]{x1D70E}_{t}^{2}(\unicode[STIX]{x1D719})=\lim _{n\rightarrow \infty }\int \left(\frac{\mathop{\sum }_{j=0}^{n-1}\left(\unicode[STIX]{x1D719}\circ f_{t}^{j}-\int \unicode[STIX]{x1D719}\,d\unicode[STIX]{x1D707}_{t}\right)}{\sqrt{n}}\right)^{2}\,d\unicode[STIX]{x1D707}_{t}.\end{eqnarray}$$
We show that  $$\begin{eqnarray}m\left\{t\in [a,b]:t+h\in [a,b]\text{ and }\frac{1}{\unicode[STIX]{x1D6F9}(t)\sqrt{-\log |h|}}\left(\frac{{\mathcal{R}}_{\unicode[STIX]{x1D719}}(t+h)-{\mathcal{R}}_{\unicode[STIX]{x1D719}}(t)}{h}\right)\leqslant y\right\}\end{eqnarray}$$
$$\begin{eqnarray}m\left\{t\in [a,b]:t+h\in [a,b]\text{ and }\frac{1}{\unicode[STIX]{x1D6F9}(t)\sqrt{-\log |h|}}\left(\frac{{\mathcal{R}}_{\unicode[STIX]{x1D719}}(t+h)-{\mathcal{R}}_{\unicode[STIX]{x1D719}}(t)}{h}\right)\leqslant y\right\}\end{eqnarray}$$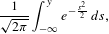 $$\begin{eqnarray}\frac{1}{\sqrt{2\unicode[STIX]{x1D70B}}}\int _{-\infty }^{y}e^{-\frac{s^{2}}{2}}\,ds,\end{eqnarray}$$
$$\begin{eqnarray}\frac{1}{\sqrt{2\unicode[STIX]{x1D70B}}}\int _{-\infty }^{y}e^{-\frac{s^{2}}{2}}\,ds,\end{eqnarray}$$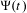 $\unicode[STIX]{x1D6F9}(t)$ is a dynamically defined function and
$\unicode[STIX]{x1D6F9}(t)$ is a dynamically defined function and 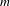 $m$ is the Lebesgue measure on
$m$ is the Lebesgue measure on 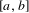 $[a,b]$, normalized in such way that
$[a,b]$, normalized in such way that 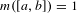 $m([a,b])=1$. As a consequence, we show that
$m([a,b])=1$. As a consequence, we show that 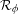 ${\mathcal{R}}_{\unicode[STIX]{x1D719}}$ is not a Lipchitz function on any subset of
${\mathcal{R}}_{\unicode[STIX]{x1D719}}$ is not a Lipchitz function on any subset of  $[a,b]$ with positive Lebesgue measure.
$[a,b]$ with positive Lebesgue measure.
Keywords
MSC classification
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 17 , Issue 3 , June 2018 , pp. 673 - 733
- Copyright
- © Cambridge University Press 2016
References
- 8
- Cited by


