Published online by Cambridge University Press: 01 March 2013
In this paper we investigate the zeros of the Estermann zeta function  $E(s; k/ \ell , \alpha )= { \mathop{\sum }\nolimits}_{n= 1}^{\infty } {\sigma }_{\alpha } (n) \exp (2\pi ink/ \ell ){n}^{- s} $ as a function of a complex variable
$E(s; k/ \ell , \alpha )= { \mathop{\sum }\nolimits}_{n= 1}^{\infty } {\sigma }_{\alpha } (n) \exp (2\pi ink/ \ell ){n}^{- s} $ as a function of a complex variable  $s$, where
$s$, where 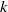 $k$ and
$k$ and 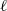 $\ell $ are coprime integers and
$\ell $ are coprime integers and  ${\sigma }_{\alpha } (n)= {\mathop{\sum }\nolimits}_{d\vert n} {d}^{\alpha } $ is the generalized divisor function with a fixed complex number
${\sigma }_{\alpha } (n)= {\mathop{\sum }\nolimits}_{d\vert n} {d}^{\alpha } $ is the generalized divisor function with a fixed complex number  $\alpha $. In particular, we study the question on how the zeros of
$\alpha $. In particular, we study the question on how the zeros of 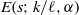 $E(s; k/ \ell , \alpha )$ depend on the parameters
$E(s; k/ \ell , \alpha )$ depend on the parameters 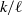 $k/ \ell $ and
$k/ \ell $ and  $\alpha $. It turns out that for some zeros there is a continuous dependency whereas for other zeros there is not.
$\alpha $. It turns out that for some zeros there is a continuous dependency whereas for other zeros there is not.