Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-24T16:47:35.024Z Has data issue: false hasContentIssue false
  • English
  • Français

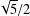A ZERO- $\!\sqrt{5}/2$ LAW FOR COSINE FAMILIES

Part of: Commutative Banach algebras and commutative topological algebras

Published online by Cambridge University Press:  17 April 2017

JEAN ESTERLE
JEAN ESTERLE*
Affiliation:
IMB, UMR 5251, Université de Bordeaux, 351, cours de la Libération, 33405 Talence, France email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

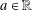Let $a\in \mathbb{R}$ , and let $k(a)$ be the largest constant such that 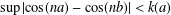$\sup |\text{cos}(na)-\cos (nb)|<k(a)$ for 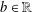$b\in \mathbb{R}$ implies that 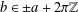$b\in \pm a+2\unicode[STIX]{x1D70B}\mathbb{Z}$ . We show that if a cosine sequence $(C(n))_{n\in \mathbb{Z}}$ with values in a Banach algebra 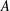$A$ satisfies $\sup _{n\geq 1}\Vert C(n)-\cos (na).1_{A}\Vert <k(a)$ , then 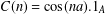$C(n)=\cos (na).1_{A}$ for 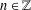$n\in \mathbb{Z}$ . Since $\!\sqrt{5}/2\leq k(a)\leq 8/3\!\sqrt{3}$ for every 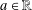$a\in \mathbb{R}$ , this shows that if some cosine family 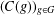$(C(g))_{g\in G}$ over an abelian group $G$ in a Banach algebra satisfies 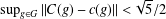$\sup _{g\in G}\Vert C(g)-c(g)\Vert <\!\sqrt{5}/2$ for some scalar cosine family 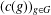$(c(g))_{g\in G}$ , then $C(g)=c(g)$ for $g\in G$ , and the constant $\!\sqrt{5}/2$ is optimal. We also describe the set of all real numbers 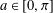$a\in [0,\unicode[STIX]{x1D70B}]$ satisfying 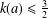$k(a)\leq \frac{3}{2}$ .

Keywords

cosine sequencescalar cosine sequenceKronecker’s theoremcommutative Banach algebracyclotomic polynomial

MSC classification

Primary: 46J45: Radical Banach algebras
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 104 , Issue 2 , April 2018 , pp. 195 - 217
Copyright
© 2017 Australian Mathematical Publishing Association Inc. 

References

Arendt, W., Batty, C. J. K., Hieber, M. and Neubrander, F., Vector-Valued Laplace Transforms and Cauchy Problems (Birkhäuser, Basel, 2001).CrossRefGoogle Scholar
Arkhangel’skii, A. V. and Ponomarev, V. I., Fundamentals of General Topology: Problems and Exercises (D. Reidel Publishing Company/Hindustan Publishing Company, 1984), (translated from Russian).Google Scholar
Batkai, A., Engel, K.-J. and Haase, M., ‘Cosine families generated by second order differential operators on W 1, 1(0, 1) with generalized Wentzell boundary conditions’, Appl. Anal. 84 (2005), 867–876.Google Scholar
Bobrowski, A. and Chojnacki, W., ‘Isolated points of some sets of bounded cosine families, bounded semigroups, and bounded groups on a Banach space’, Studia Math. 217 (2013), 219–241.CrossRefGoogle Scholar
Bobrowski, A., Chojnacki, W. and Gregosiewicz, A., ‘On close-to-scalar one-parameter cosine families’, J. Math. Anal. Appl. 429 (2015), 383–394.Google Scholar
Chojnacki, W., ‘On cosine families close to scalar cosine families’, J. Aust. Math. Soc. 99 (2015), 166–174.Google Scholar
Cox, R. H., ‘Matrices all of whose powers lie close to the identity’, Amer. Math. Monthly 73 (1966), 813.Google Scholar
Dales, H. G., Banach Algebras and Automatic Continuity, London Mathematical Society Monographs, 24 (Clarendon Press, Oxford, 2001).CrossRefGoogle Scholar
Esterle, J., ‘Bounded cosine functions close to continuous scalar bounded cosine functions’, Int. Eq. Op. Th. 85 (2016), 347–357.CrossRefGoogle Scholar
Haase, M., ‘The group reduction for bounded cosine functions on UMD spaces’, Math. Z. 262(2) (2009), 281–299.CrossRefGoogle Scholar
Haase, M., ‘The functional calculus approach to cosine operator functions’, in: Recent Trends in Analysis, Proceedings of the Conference in honour of N. K. Nikolski held in Bordeaux 2011 (Theta Foundation, Bucharest, 2013), 123–147.Google Scholar
Hirschfeld, R. A., ‘On semi-groups in Banach algebras close to the identity’, Proc. Japan Acad. Ser. A Math. Sci. 44 (1968), 755.Google Scholar
Kahane, J. P. and Salem, R., Ensembles Parfaits et Séries Trigonométriques (Hermann, Paris, 1963).Google Scholar
Nagy, B., ‘Cosine operator functions and the abstract Cauchy problem’, Period. Math. Hungar. 7 (1976), 15–18.Google Scholar
Nakamura, M. and Yoshida, M., ‘On a generalization of a theorem of Cox’, Proc. Japan Acad. Ser. A Math. Sci. 43 (1967), 108–110.Google Scholar
Schwenninger, F. and Zwart, H., ‘Less than one, implies zero’, Studia Math. 229 (2015), 181–188.Google Scholar
Schwenninger, F. and Zwart, H., ‘Zero-two law for cosine families’, J. Evol. Equ. 15 (2015), 559–569.Google Scholar
Travis, C. and Webb, G., ‘Cosine families and abstract nonlinear second order differential equation’, Acta Math. Acad. Sci. Hungar. 32 (1978), 75–96.Google Scholar
Wallen, L. J., ‘On the magnitude of ∥x n - 1∥ in a normed algebra’, Proc. Amer. Math. Soc. 18 (1967), 956.Google Scholar
Washington, L. C., Cyclotomic Fields, 2nd edn, Graduate Texts in Mathematics, 83 (Springer, New York–Berlin–Heidelberg, 1997).CrossRefGoogle Scholar