Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-23T23:12:25.975Z Has data issue: false hasContentIssue false
  • English
  • Français

THE VECTOR-VALUED TENT SPACES $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}T^1$ AND 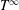$T^{\infty }$

Part of: Harmonic analysis in several variables

Published online by Cambridge University Press:  15 May 2014

MIKKO KEMPPAINEN
MIKKO KEMPPAINEN*
Affiliation:
Department of Mathematics and Statistics,University of Helsinki, Gustaf Hällströmin katu 2b, FI-00014 Helsinki, Finland email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Tent spaces of vector-valued functions were recently studied by Hytönen, van Neerven and Portal with an eye on applications to 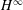$H^{\infty }$-functional calculi. This paper extends their results to the endpoint cases 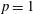$p=1$ and 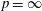$p=\infty $ along the lines of earlier work by Harboure, Torrea and Viviani in the scalar-valued case. The main result of the paper is an atomic decomposition in the case 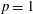$p=1$, which relies on a new geometric argument for cones. A result on the duality of these spaces is also given.

Keywords

vector-valued harmonic analysisatomic decompositionstochastic integration

MSC classification

Secondary: 42B35: Function spaces arising in harmonic analysis
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 97 , Issue 1 , August 2014 , pp. 107 - 126
Copyright
Copyright © 2014 Australian Mathematical Publishing Association Inc. 

References

Blasco, O., ‘Hardy spaces of vector-valued functions: duality’, Trans. Amer. Math. Soc. 308 (1988), 495–507.CrossRefGoogle Scholar
Bourgain, J., ‘Vector-valued singular integrals and the H 1-BMO duality’, in: Probability Theory and Harmonic Analysis (Cleveland, Ohio, 1983), Monographs and Textbooks in Pure and Applied Mathematics, 98 (Dekker, New York, 1986), 1–19.Google Scholar
Clément, P., de Pagter, B., Sukochev, F. A. and Witvliet, H., ‘Schauder decomposition and multiplier theorems’, Studia Math. 138 (2000), 135–163.Google Scholar
Coifman, R. R., Meyer, Y. and Stein, E. M., ‘Some new function spaces and their applications to harmonic analysis’, J. Funct. Anal. 62(2) (1985), 304–335.CrossRefGoogle Scholar
Harboure, E., Torrea, J. L. and Viviani, B. E., ‘A vector-valued approach to tent spaces’, J. Anal. Math. 56 (1991), 125–140.CrossRefGoogle Scholar
Hytönen, T., ‘Vector-valued wavelets and the Hardy space H 1(ℝn, X)’, Studia Math. 172 (2006), 125–147.CrossRefGoogle Scholar
Hytönen, T., van Neerven, J. M. A. M. and Portal, P., ‘Conical square function estimates in UMD Banach spaces and applications to H ∞-functional calculi’, J. Anal. Math. 106 (2008), 317–351.CrossRefGoogle Scholar
Hytönen, T. and Weis, L., ‘The Banach space-valued BMO, Carleson’s condition, and paraproducts’, J. Fourier Anal. Appl. 16 495–513.CrossRefGoogle Scholar
Janson, S., Gaussian Hilbert Spaces, Cambridge Tracts in Mathematics, 129 (Cambridge University Press, Cambridge, 1997).CrossRefGoogle Scholar
Kalton, N. and Weis, L., The $H^\infty $-functional calculus and square function estimates, in preparation.Google Scholar
Mei, T., ‘BMO is the intersection of two translates of dyadic BMO’, C. R. Math. Acad. Sci. Paris. 336 (2003), 1003–1006.CrossRefGoogle Scholar
Meyer, Y. and Coifman, R. R., ‘Wavelets’, Calderón–Zygmund and multilinear operators, Translated from the 1990 and 1991 French originals by David Salinger, Cambridge Studies in Advanced Mathematics, 48 (Cambridge University Press, Cambridge, 1997).Google Scholar
van Neerven, J. M. A. M., ‘γ-radonifying operators—a survey’, in: The AMSI–ANU Workshop on Spectral Theory and Harmonic Analysis, Proceedings of the Centre for Mathematics and its Applications, 44 (Australian National University, Canberra, 2010), 1–61.Google Scholar
van Neerven, J. M. A. M. and Weis, L., ‘Stochastic integration of functions with values in a Banach space’, Studia Math. 166 (2005), 131–170.CrossRefGoogle Scholar
Rosiński, J. and Suchanecki, Z., ‘On the space of vector-valued functions integrable with respect to the white noise’, Colloq. Math. 43 (1980), 183–201; 1981.CrossRefGoogle Scholar