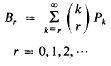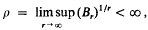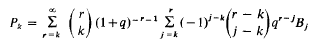Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Takács, Lajos
1991.
A Moment Convergence Theorem.
The American Mathematical Monthly,
Vol. 98,
Issue. 8,
p.
742.