Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-27T04:18:42.505Z Has data issue: false hasContentIssue false
  • English
  • Français

Unique solvability of the strong Hamburger moment problem

Part of: Miscellaneous topics of analysis in the complex domain

Published online by Cambridge University Press:  09 April 2009

Olav Njåstad  and
W. J. Thron
Olav Njåstad
Affiliation:
Department of Mathematics, University of Trondeim, N-7034 Trondheim-NTH, Norway
W. J. Thron
Affiliation:
Department of Mathematics, Campus Box 426, University of Colorado, Boulder, Colorado 80309, U.S.A.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

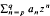Methods from the theory of orthogonal polynomials are extended to L-polynomials . By this means the authors and W. B. Jones (J. Math. Anal. Appl. 98 (1984), 528–554) solved the strong Hamburger moment problem, that is, given a double sequence 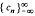, to find a distribution function ψ(t), non-decreasing, with an infinitenumber of points of increase and bounded on −∞ < t < ∞, such that for all integers . In this article further menthods such as analogues of the Lioville-Ostrogradski formula and of the Christoffel-Darboux formula are developed to investigated When the moment porblem has a unique solution. This will be the case if and only if a sequence of nested disks associated with the sequence 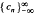has only a point as its intersection (the so called limit point case).

MSC classification

Secondary: 30E05: Moment problems, interpolation problems
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 40 , Issue 1 , February 1986 , pp. 5 - 19
Copyright
Copyright © Australian Mathematical Society 1986

References

[1]Ajkiezer, N. I., The classical moment problem and some related questions in analysis (Hafner Publishing Company, New York, 1965).Google Scholar
[2]Brezinski, C., Padé-type approximation and general orthogonal polynomials (Birkhäuser Verlag, Basel, Boston, Stuttgart, 1980).CrossRefGoogle Scholar
[3]Cobindarajula, Z., ‘Recurrence relations for the inverse moments of the positive binomial variable’, J. Amer. Statist. Assoc. 57 (1963), 468–473.Google Scholar
[4]Jones, William B., Njåstad, Olav and Thron, W. J., ‘Orthogonal Laurent polynomials and the strong Hamburger Moment Problem’, J. Math. Anal. Appl. 98 (1984), 528–554.CrossRefGoogle Scholar
[5]Jones, William B., Njåstad, Olav and Thron, W. J., ‘Continued fractions and strong Hamburger moment problem’, Proc. London Math. Soc. (3), 47 (1983), 363–384.CrossRefGoogle Scholar
[6]Jones, William B., Njåstad, Olav and Thron, W. J., ‘Two-point Padé expansions for a family of analytic functions’, J. Comput. Appl. Math. 9 (1983), 105–123.CrossRefGoogle Scholar
[7]Jones, William B. and Thron, W. J., ‘Orthogonal Laurent polynomials and Gaussian quadrature’, pp. 449–455, Quantum mechanics in mathematics, chemistry and physics, (Eds., Gustafson, K. and Reinhardt, W. P.), Plenum Publishing Corp., New York, (1981).CrossRefGoogle Scholar
[8]Jones, William B. and Thron, W. J., ‘Survey of contiuned fractions methods of solving moment problems and related topics’, pp. 4–37, Analytic theory of continued fractions, Proceedings, Loen, Norway, 1981, Lecture Notes in Mathematics, No. 932, Springer Verlag, Berlin (1982).Google Scholar
[9]Jones, William B., Thron, W. J. and Waadeland, H., ‘A strong Stieltjes moment problem’, Trans. Amer. Math. Soc. 261 (1980), 503–528.CrossRefGoogle Scholar
[10]Kabe, D. G., ‘Inverse moments and discrete distributions’, Canad. J. Statist. 4 (1976), 133–142.CrossRefGoogle Scholar
[11]Mendenhall, W. and Lehman, E. H. Jr, ‘An approximation of the negative moments of the positive binomial useful in life testing’, Technometrics 2 (1960), 227–242.CrossRefGoogle Scholar
[12]Landau, H. J., ‘The classical moment problem: Hilbertian proofs’, J. Functional Analysis 38 (1980), 255–272.CrossRefGoogle Scholar
[13]Njåstad, Olav and Thron, W. J., ‘The theory of sequences of orthogonal L-polynomials’, Padé approximants and continued fractions, 54–91, (Eds., Haakon, Waadeland and Hans, Wallin), Det Kongelige Norske Videnskabers Selskab, Skrifter (1983), No. 1.Google Scholar
[14]Njåstad, Olav and Thron, W. J., ‘Completely convergent APT-fractions and strong Hamburger moment problems with a unique solution’, Det Kongelige Norske Videnskabers Selskab, Skrifter, No. 2 (1984), 1–7.Google Scholar
[15]Shohat, J. A. and Tamarkin, J. D., The problem of moments (Mathematical Surveys No. 1, Amer. Math. Soc., Providence, R. I., 1943).CrossRefGoogle Scholar
[16]Thomas, D. L., ‘Reciprocal moments of linear combinations of exponential variates’, J. Amer. Statist. Assoc. 71 (1976), 506–512.CrossRefGoogle Scholar