Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-25T16:25:56.656Z Has data issue: false hasContentIssue false
  • English
  • Français

Unique Hahn-Banach theorems for spaces of homogeneous polynomials

Part of: Topological linear spaces and related structures Measures, integration, derivative, holomorphy

Published online by Cambridge University Press:  09 April 2009

R. Aron ,
C. Boyd  and
Y. S. Choi
R. Aron
Affiliation:
Department of Mathematics Kent State UniversityKent Ohio 44242USA e-mail: [email protected]
C. Boyd
Affiliation:
Department of Mathematics University College DublinBelfield Dublin 4Ireland e-mail: [email protected]
Y. S. Choi
Affiliation:
Department of Mathematics Pohang University of Science and TechnologyPohang 790South Korea e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

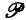We investigate certain norm and continuity conditions that provide us with ‘uniqe Hahn-Banch Theorems’ from (nc0) to 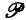(nℓ∞) and from 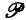N(nE) to 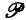N(nE″). We show that there is a unique norm-preserving extension for norm-attaining 2-homogeneous polynomials on complex c0 to ℓ∈ but there is no unique norm-preserving extension from 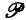(3c0) to 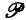(3ℓ∈).

MSC classification

Secondary: 46G25: (Spaces of) multilinear mappings, polynomials 46A22: Theorems of Hahn-Banach type; extension and lifting of functionals and operators
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 70 , Issue 3 , June 2001 , pp. 387 - 400
Copyright
Copyright © Australian Mathematical Society 2001

References

[1]Aron, R. M. and Berner, P., ‘A Hahn-Banach extension theorem for analytic mappings’, Bull. Math. Soc. France 106 (1978), 3–24.CrossRefGoogle Scholar
[2]Aron, R. M., Herves, C. and Valdivia, M., ‘Weakly continuous mappings on Banach spaces’, J. Funct. Anal. 52 (1983), 198–204.CrossRefGoogle Scholar
[3]Bogdanowicz, W., ‘On the weak continuity of the polynomial functionals on the spaces c 0’, Bull. Acad. Polon. Sci. Sér. Math. Astronom. Phys. 5 (1957), 243–246 (in Russian).Google Scholar
[4]Carne, T. K., Cole, B. and Gamelin, T. W., ‘A uniform algebra of analytic functions on a Banach space’, Trans. Amer. Math. Soc. 314 (1989), 639–659.Google Scholar
[5]Davie, A. M. and Gamelin, T. W., ‘A theorem on polynomial-star approximation’, Proc. Amer. Math. Soc. 106 (1989), 351–356.CrossRefGoogle Scholar
[6]Dimant, V. and Dineen, S., ‘Banach subspaces of spaces of holomorphic functions and related topics’, Math. Scand. 83 (1998), 142–160.CrossRefGoogle Scholar
[7]Dineen, S., Complex analysis on infinite dimensional spaces, Monographs in Math. (Springer, London, 1999).Google Scholar
[8]Dineen, S. and Timoney, R., ‘Complex geodesics on convex domains’, in: Progress in Functional Analysis (ed. Bierstedt, K.el al.), Math. Studies 170 (North-Holland, Amsterdam, 1992) pp. 333–365.Google Scholar
[9]Galindo, P., García, D., Maestre, M. and Mujica, J., ‘Extension of multilinear mappings on Banach spaces’, Studia Math. 108 (1994), 55–76.CrossRefGoogle Scholar
[10]Godefroy, G., ‘Points de Namioka, espaces normante, applications à la Théorie isométrique de la dualité’, Israel J. Math. 38 (1981), 209–220.CrossRefGoogle Scholar
[11]Harmand, P., Werner, D. and Werner, W., M-ideal in Banach spaces and Banach algebras, Lecture Notes in Math. 1547 (Springer, Berlin, 1993).CrossRefGoogle Scholar
[12]Kirwan, P., Complexification of multilinear and polynomial mappings on normed spaces (Ph.D. Thesis, National University of Ireland, Galway, 1997).Google Scholar
[13]Lindström, M. and Ryan, R., ‘Applications of ultraproducts to infinite dimensional holomorphy’, Math. Scand. 71 (1992), 229–242.CrossRefGoogle Scholar
[14]Pelczyński, A., ‘A property of multilinear operations’, Studia Math. 16 (1957), 173–182.CrossRefGoogle Scholar
[15]Smith, M. A. and Sullivan, F., ‘Extremely smooth Banach spaces’, in: Banach spaces of analytic functions. Proc. Conf. Kent, Ohio 1976 (eds. Baker, J., Cleaver, C. and Diestel, J.), Lecture Notes in Math. 604 (Springer, Berlin, 1977) pp. 125–137.Google Scholar
[16]Sullivan, F., ‘Geomentric properties determined by higher duals of a Banach space’, Illinois J. Math. 21 (1977), 315–331.CrossRefGoogle Scholar
[17]Zalduendo, I., ‘A canonical extension for analytic functions on Banach spaces’, Trans. Amer. Math. Soc. 320 (1990), 747–763.CrossRefGoogle Scholar