No CrossRef data available.
Article contents
Unbiased distinguishing of translation families and integrability with respect to a convolution of measures
Published online by Cambridge University Press: 09 April 2009
Extract
Let M1 and M2 be two sets of probability measures defined on Rn. Ameasurable R1 valued function h (l ≧1) is said to distinguish M1from M2unbiasedly if there are numbers or vectors I1 and I2 (I1≠I2) such that ∫Rnh(x)m(dx) = Ii if m is in Mi (i = 1, 2). Here we shall be concerned with the case where M1 and M2 are translation families, in that all of the elements of Mi are translates of a single measure mi. This means that if, for any t in Rn, mtt is the measure defined by  , where E−t {x−t: x ∈ E}, then Mi =
, where E−t {x−t: x ∈ E}, then Mi =  , where T is a subset of Rn. If M1 and M2 are of this type, we will investigate the conditions under which there does not exist a function to distinguishing M1 from M2 unbiasedly. A case of special interest arises if m2(E) = m1(BE) = m1
, where T is a subset of Rn. If M1 and M2 are of this type, we will investigate the conditions under which there does not exist a function to distinguishing M1 from M2 unbiasedly. A case of special interest arises if m2(E) = m1(BE) = m1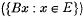 with B a non-degenerate n × n matrix, and particularly a nonzero multiple (scale parameter) of the identity matrix, cf. [1], [2]. For simplicity, take l = 1.
with B a non-degenerate n × n matrix, and particularly a nonzero multiple (scale parameter) of the identity matrix, cf. [1], [2]. For simplicity, take l = 1.
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1968


