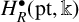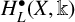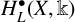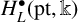1 Introduction
Let L be a topological group acting continuously on a topological space X. For any commutative ring
![]() $\Bbbk $
, the L-equivariant (sheaf) cohomology
$\Bbbk $
, the L-equivariant (sheaf) cohomology
![]() $H^{\bullet }_L(X,\Bbbk )$
is naturally a left
$H^{\bullet }_L(X,\Bbbk )$
is naturally a left
![]() $H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-module, where
$H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-module, where
![]() $\mathrm {pt}$
is the singleton trivially acted upon by L. This module structure can explicitly be described as follows:
$\mathrm {pt}$
is the singleton trivially acted upon by L. This module structure can explicitly be described as follows:
where
![]() $c\in H^{\bullet }_L(\mathrm {pt},\Bbbk )$
,
$c\in H^{\bullet }_L(\mathrm {pt},\Bbbk )$
,
![]() $m\in H^{\bullet }_L(X,\Bbbk )$
,
$m\in H^{\bullet }_L(X,\Bbbk )$
,
![]() $a_X:X\to \mathrm {pt}$
is the constant map,
$a_X:X\to \mathrm {pt}$
is the constant map,
![]() $a_X^{\star }$
is the equivariant pull-back, and
$a_X^{\star }$
is the equivariant pull-back, and
![]() $\cup $
denotes the cup product.
$\cup $
denotes the cup product.
The main idea of this paper is to define the structure of a right
![]() $H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module on
$H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module on
![]() $H^{\bullet }_L(X,\Bbbk )$
possibly for
$H^{\bullet }_L(X,\Bbbk )$
possibly for
![]() $R\ne L$
. Of course, if
$R\ne L$
. Of course, if
![]() $L=R$
and we do not have any additional information about X, then we can do it, simply setting
$L=R$
and we do not have any additional information about X, then we can do it, simply setting
![]() $ md=m \cup a_X^{\star }(d) $
. This construction is however very restrictive. Therefore, we define the right
$ md=m \cup a_X^{\star }(d) $
. This construction is however very restrictive. Therefore, we define the right
![]() $H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module structure on
$H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module structure on
![]() $H^{\bullet }_L(X,\Bbbk )$
with the help of an L-equivariant map
$H^{\bullet }_L(X,\Bbbk )$
with the help of an L-equivariant map
![]() $A:X\to G/R$
, which we call the twisting map (Section 3.1). In this definition, refer to (3-2), the groups L and R are contained in a larger group G, which we assume to be a compact Lie group for the most part of the paper.
$A:X\to G/R$
, which we call the twisting map (Section 3.1). In this definition, refer to (3-2), the groups L and R are contained in a larger group G, which we assume to be a compact Lie group for the most part of the paper.
The main result of the paper is the proof of the following equivariant form of the Künneth isomorphism. Let X and Y be topological spaces, and
![]() $L,R,P,Q$
be closed subgroups of a compact Lie group G such that
$L,R,P,Q$
be closed subgroups of a compact Lie group G such that
![]() $R\subset P$
and
$R\subset P$
and
![]() $Q\subset P$
, the groups L and R act commutatively and continuously on X on the left and on the right, respectively, and Q acts continuously on Y on the left. Then we consider the space
$Q\subset P$
, the groups L and R act commutatively and continuously on X on the left and on the right, respectively, and Q acts continuously on Y on the left. Then we consider the space
where
![]() $\sim $
is the equivalence relation defined by
$\sim $
is the equivalence relation defined by
![]() $(x,p,y)\sim (xr,r^{-1}pq,q^{-1}y)$
for any
$(x,p,y)\sim (xr,r^{-1}pq,q^{-1}y)$
for any
![]() $r\in R$
and
$r\in R$
and
![]() $q\in Q$
. Let also
$q\in Q$
. Let also
![]() $\alpha :X\to G$
be a continuous L- and R-equivariant map. It induces the morphism of left L-spaces
$\alpha :X\to G$
be a continuous L- and R-equivariant map. It induces the morphism of left L-spaces
![]() $A:X/R\to G/R$
by
$A:X/R\to G/R$
by
![]() $A(xR)=\alpha (x)R$
. Considering A as a twisting map, we get the structure of a right
$A(xR)=\alpha (x)R$
. Considering A as a twisting map, we get the structure of a right
![]() $H_R^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
$H_R^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
![]() $H_L^{\bullet }(X/R,\mathrm {pt})$
and thus the structure of a right
$H_L^{\bullet }(X/R,\mathrm {pt})$
and thus the structure of a right
![]() $H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module through the natural morphism
$H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module through the natural morphism
![]() $H_P^{\bullet }(\mathrm {pt},\Bbbk )\to H_R^{\bullet }(\mathrm {pt},\Bbbk )$
. Similarly, the natural morphism
$H_P^{\bullet }(\mathrm {pt},\Bbbk )\to H_R^{\bullet }(\mathrm {pt},\Bbbk )$
. Similarly, the natural morphism
![]() $H_P^{\bullet }(\mathrm {pt},\Bbbk )\to H_Q^{\bullet }(\mathrm {pt},\Bbbk )$
defines the structure of a left
$H_P^{\bullet }(\mathrm {pt},\Bbbk )\to H_Q^{\bullet }(\mathrm {pt},\Bbbk )$
defines the structure of a left
![]() $H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
$H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
![]() $H^{\bullet }_Q(Y,\Bbbk )$
by modifying the canonical left module structure. Then there exists a homomorphism of rings and bimodules
$H^{\bullet }_Q(Y,\Bbbk )$
by modifying the canonical left module structure. Then there exists a homomorphism of rings and bimodules
It is an isomorphism under certain restrictions (I)–(VIII) close to equivariant formality.
The theory we develop is illustrated by two basic examples: computation of the equivariant cohomology of the flag variety (Section 4.6) and the realization of the cohomology of the point with a twisted right action as a standard bimodule (Section 4.7).
Our main example however concerns Bott–Samelson varieties
![]() $\mathrm {BS}(\underline {t})$
in Section 5 for a sequence of reflections
$\mathrm {BS}(\underline {t})$
in Section 5 for a sequence of reflections
![]() $\underline {t}$
. Here we use the original definition of these varieties by Bott and Samelson [Reference Bott and SamelsonBS]. Note that
$\underline {t}$
. Here we use the original definition of these varieties by Bott and Samelson [Reference Bott and SamelsonBS]. Note that
![]() $\mathrm {BS}(\underline {t})$
is a left K-space for the maximal compact torus K. Thus, we can consider the K-equivariant cohomology
$\mathrm {BS}(\underline {t})$
is a left K-space for the maximal compact torus K. Thus, we can consider the K-equivariant cohomology
![]() $H_K^{\bullet }(\mathrm {BS}(\underline {t}),\Bbbk )$
for any commutative ring
$H_K^{\bullet }(\mathrm {BS}(\underline {t}),\Bbbk )$
for any commutative ring
![]() $\Bbbk $
. In Theorem 5.3, we establish the isomorphism of rings and
$\Bbbk $
. In Theorem 5.3, we establish the isomorphism of rings and
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules
${\mathcal R}$
-bimodules
where
![]() $\underline {t}=(t_1,\ldots ,t_n)$
and
$\underline {t}=(t_1,\ldots ,t_n)$
and
![]() ${\mathcal R}^{t_i}$
denotes the subring of
${\mathcal R}^{t_i}$
denotes the subring of
![]() $t_i$
-invariants of the K-equivariant cohomology of the point
$t_i$
-invariants of the K-equivariant cohomology of the point
![]() ${\mathcal R}$
. We call the tensor product in the left-hand side the Bott–Samelson bimodule. Compared with other constructions of similar isomorphisms (for example, [Reference Webster and WilliamsonWW, Theorem 1.6]), our construction has the following advantages:
${\mathcal R}$
. We call the tensor product in the left-hand side the Bott–Samelson bimodule. Compared with other constructions of similar isomorphisms (for example, [Reference Webster and WilliamsonWW, Theorem 1.6]), our construction has the following advantages:
-
• the reflections
 $t_i$
are not necessarily simple;
$t_i$
are not necessarily simple; -
• the proof is localization free;
-
•
 $\theta _{\underline {t}}$
is constructed explicitly as a quotient of the composition of a pull-back and a (nonequivariant) Künneth isomorphism;
$\theta _{\underline {t}}$
is constructed explicitly as a quotient of the composition of a pull-back and a (nonequivariant) Künneth isomorphism; -
• the ring of coefficients
 $\Bbbk $
can be any commutative ring of finite global dimension.
$\Bbbk $
can be any commutative ring of finite global dimension.
The isomorphisms
![]() $\theta _{\underline {t}}$
satisfy the quite expected concatenation property (Theorem 5.4) and their restrictions to the K-fixed points are computed (Theorem 5.7).
$\theta _{\underline {t}}$
satisfy the quite expected concatenation property (Theorem 5.4) and their restrictions to the K-fixed points are computed (Theorem 5.7).
The explicit construction of the isomorphisms
![]() $\theta _{\underline {t}}$
pays off in Section 6, where we consider the morphisms of
$\theta _{\underline {t}}$
pays off in Section 6, where we consider the morphisms of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules
${\mathcal R}$
-bimodules
![]() $H_K^{\bullet }(\mathrm {BS}(\underline {t}),\Bbbk )\to H_K^{\bullet }(\mathrm {BS}(\underline {r}),\Bbbk )$
. The morphisms we consider are built up from the elementary morphisms, which fall into two types: one-color morphisms and two-color morphisms. The former morphisms (Sections 6.2–6.3) exist for arbitrary reflections. However, we require the reflections to be simple for the latter morphisms (Sections 6.4–6.9), as the generalization to arbitrary reflections would require varieties more general than those considered in this paper.
$H_K^{\bullet }(\mathrm {BS}(\underline {t}),\Bbbk )\to H_K^{\bullet }(\mathrm {BS}(\underline {r}),\Bbbk )$
. The morphisms we consider are built up from the elementary morphisms, which fall into two types: one-color morphisms and two-color morphisms. The former morphisms (Sections 6.2–6.3) exist for arbitrary reflections. However, we require the reflections to be simple for the latter morphisms (Sections 6.4–6.9), as the generalization to arbitrary reflections would require varieties more general than those considered in this paper.
Composing elementary morphisms horizontally as well as vertically, we get more general morphisms that are convenient to represent by planar diagrams [Reference Elias and WilliamsonEW]. We assume here that such diagrams do not have horizontal tangent lines and that no two vertices have the same y-coordinate (Soergel graphs in the terminology of [Reference Elias and WilliamsonEW]). We can cut any such diagram by horizontal lines into strips containing only one vertex. A typical picture looks like this:

We find a geometric description for any such horizontal strip as a pull-back or a push-forward or the composition of both. The first two cases are considered and computed in coordinates in Sections 6.2 and 6.3, and the compositions are considered in Section 6.5 and are extended horizontally in Section 6.9. One can see that the compositions are necessary only for strips containing two-color vertices. Such strips can be further cut to the upper and the lower parts, which are given by pull-backs and push-forwards, respectively. For example, the diagram above receives an additional cut. The corresponding strip turns into the following diagram:

This picture shows that we need to consider varieties more general than Bott–Samelson varieties (Section 6.4). We also prove that the normalization criterion for two-color morphisms holds (Lemma 6.4), which allows us to identify them with the morphism
![]() $f_{s,r}$
defined by Libedinsky in [Reference LibedinskyLi, Lemme 4.7]. Note that Libedinsky proved in the same paper that in the case of simple reflections, all morphisms between Bott–Samelson modules are generated (as linear combinations) by morphisms corresponding to planar diagrams.
$f_{s,r}$
defined by Libedinsky in [Reference LibedinskyLi, Lemme 4.7]. Note that Libedinsky proved in the same paper that in the case of simple reflections, all morphisms between Bott–Samelson modules are generated (as linear combinations) by morphisms corresponding to planar diagrams.
If we compose two-color diagrams differently as in Section 6.7, we obtain the Jones–Wenzl projectors, which we also represent by two-color vertices of the same valency as the vertices representing the morphisms
![]() $f_{s,r}$
. Some relations can be proved using the direct construction of the morphisms. The examples are given in Sections 6.3, 6.7, and 6.8. Note we prove these relations on the level of topology of the underlying varieties unlike [Reference EliasE, Reference Elias and KhovanovEKh], which argue on the level of polynomial algebras. We conjecture that all other relations defining the diagrammatic category [Reference Elias and WilliamsonEW, (5.1)–(5.11)] as well as their versions for nonsimple reflections can also be proved similarly. In the case of sequences of simple reflections, these relations were proved in [Reference Riche and WilliamsonRW] for morphisms between (shifted) Bott–Samelson parity complexes [Reference Riche and WilliamsonRW, Ch. 10].
$f_{s,r}$
. Some relations can be proved using the direct construction of the morphisms. The examples are given in Sections 6.3, 6.7, and 6.8. Note we prove these relations on the level of topology of the underlying varieties unlike [Reference EliasE, Reference Elias and KhovanovEKh], which argue on the level of polynomial algebras. We conjecture that all other relations defining the diagrammatic category [Reference Elias and WilliamsonEW, (5.1)–(5.11)] as well as their versions for nonsimple reflections can also be proved similarly. In the case of sequences of simple reflections, these relations were proved in [Reference Riche and WilliamsonRW] for morphisms between (shifted) Bott–Samelson parity complexes [Reference Riche and WilliamsonRW, Ch. 10].
It also would be interesting to find a geometric description for morphisms represented by strips containing more than one vertex, at least in the case when all vertices of this strip are represented either by pull-backs or push-forwards.
2 Notation and basic constructions
2.1 Set theory
To avoid confusion with quotients, we denote the difference of sets by
![]() $-$
. For a sequence of indices
$-$
. For a sequence of indices
![]() $1\leqslant i_1<i_2<\cdots <i_k\leqslant n$
and sets
$1\leqslant i_1<i_2<\cdots <i_k\leqslant n$
and sets
![]() $X_1,\ldots ,X_n$
, we denote by
$X_1,\ldots ,X_n$
, we denote by
![]() $\mathop{\rm pr}\nolimits _{i_1,i_2,\ldots ,i_k}$
the projection
$\mathop{\rm pr}\nolimits _{i_1,i_2,\ldots ,i_k}$
the projection
![]() $X_1\times \cdots \times X_n\to X_{i_1}\times \cdots \times X_{i_k}$
to the corresponding coordinates.
$X_1\times \cdots \times X_n\to X_{i_1}\times \cdots \times X_{i_k}$
to the corresponding coordinates.
Let X, Y, and S be sets, and
![]() $f:X\to S$
and
$f:X\to S$
and
![]() $g:Y\to S$
be maps. The fiber product of X and Y with respect to f and g is the set
$g:Y\to S$
be maps. The fiber product of X and Y with respect to f and g is the set
We often use the singleton
![]() $\mathrm {pt}$
whose unique element is denoted by
$\mathrm {pt}$
whose unique element is denoted by
![]() ${pt}$
.
${pt}$
.
2.2 Sequences
We denote sequences by
![]() $\underline {s}$
,
$\underline {s}$
,
![]() $\underline {t}$
,
$\underline {t}$
,
![]() $\underline {r}$
, and so forth. The length n of a finite sequence
$\underline {r}$
, and so forth. The length n of a finite sequence
![]() $\underline {s}$
is denoted by
$\underline {s}$
is denoted by
![]() $|\underline {s}|$
and its ith element is denoted by
$|\underline {s}|$
and its ith element is denoted by
![]() $s_i$
. Thus,
$s_i$
. Thus,
![]() $\underline {s}=(s_1,\ldots ,s_n)$
. The case
$\underline {s}=(s_1,\ldots ,s_n)$
. The case
![]() $|\underline {s}|=0$
corresponds to the empty sequence
$|\underline {s}|=0$
corresponds to the empty sequence
![]() $\underline {\varnothing }$
. If however
$\underline {\varnothing }$
. If however
![]() $|\underline {s}|>0$
, then we get the truncated sequence
$|\underline {s}|>0$
, then we get the truncated sequence
![]() $\underline {s}'=(s_1,\ldots ,s_{n-1})$
. If
$\underline {s}'=(s_1,\ldots ,s_{n-1})$
. If
![]() $\underline {t}=(t_1,\ldots ,t_m)$
is another sequence, we consider their concatenation
$\underline {t}=(t_1,\ldots ,t_m)$
is another sequence, we consider their concatenation
![]() $\underline {st}=(s_1,\ldots ,s_n,t_1,\ldots ,t_m)$
. Obviously,
$\underline {st}=(s_1,\ldots ,s_n,t_1,\ldots ,t_m)$
. Obviously,
![]() $|\underline {st}|=|\underline {s}|+|\underline {t}|$
. In Section 5.3, we use a similar notation for collection of integers
$|\underline {st}|=|\underline {s}|+|\underline {t}|$
. In Section 5.3, we use a similar notation for collection of integers
![]() $\underline {c}$
thought of as upper triangular matrices.
$\underline {c}$
thought of as upper triangular matrices.
2.3 Modules
Let M and
![]() $M'$
be right modules over rings R and
$M'$
be right modules over rings R and
![]() $R'$
, respectively. Suppose that there is an isomorphism of rings
$R'$
, respectively. Suppose that there is an isomorphism of rings
![]() $\iota :R\stackrel \sim \to R'$
. Then a map
$\iota :R\stackrel \sim \to R'$
. Then a map
![]() $\mu :M\to M'$
is called an isomorphism of modules if it is an isomorphism of the underlying abelian groups and
$\mu :M\to M'$
is called an isomorphism of modules if it is an isomorphism of the underlying abelian groups and
for any
![]() $m\in M$
and
$m\in M$
and
![]() $r\in R$
. We assume similar definitions for left modules and bimodules.
$r\in R$
. We assume similar definitions for left modules and bimodules.
The tensor product of a right R-module M by a left R-module N is denoted as usual by
![]() $M\otimes _R N$
. If R is commutative, we can also consider the nth tensor power
$M\otimes _R N$
. If R is commutative, we can also consider the nth tensor power
![]() $N\otimes _R\cdots \otimes _RN$
with n factors, which we denote by
$N\otimes _R\cdots \otimes _RN$
with n factors, which we denote by
![]() $N^{\otimes _Rn}$
.
$N^{\otimes _Rn}$
.
As the rings and (bi)modules in this paper are represented by cohomologies, they are naturally graded. The component of degree i of M is denoted by
![]() $M^i$
. In Section 6, we also consider the shift of grading: if M is a graded (bi)module and n is an integer, then we denote by
$M^i$
. In Section 6, we also consider the shift of grading: if M is a graded (bi)module and n is an integer, then we denote by
![]() $M(n)$
the graded (bi)module such that
$M(n)$
the graded (bi)module such that
![]() $M(n)^i=M^{n+i}$
.
$M(n)^i=M^{n+i}$
.
2.4 Group actions and quotients
Let a group G act on a set X on the left. We denote this fact by
![]() $G\circlearrowright X$
. Then we denote by
$G\circlearrowright X$
. Then we denote by
![]() $G\mathop {\backslash } X$
the set of left G-orbits and by
$G\mathop {\backslash } X$
the set of left G-orbits and by
![]() ${}^{G\!}X$
the set of G-fixed points of X, that is, of points
${}^{G\!}X$
the set of G-fixed points of X, that is, of points
![]() $x\in X$
such that
$x\in X$
such that
![]() $gx=x$
for any
$gx=x$
for any
![]() $g\in G$
. We have the quotient map
$g\in G$
. We have the quotient map
![]() $X\to G\mathop {\backslash } X$
that sends x to
$X\to G\mathop {\backslash } X$
that sends x to
![]() $Gx$
. We use the notation
$Gx$
. We use the notation
![]() $X/G$
and
$X/G$
and
![]() $X^G$
for right group actions. For example, let
$X^G$
for right group actions. For example, let
![]() $P^1(\mathbb C)=({\mathbb C}^2-\{(0,0)\})/{\mathbb C}^\times $
, where
$P^1(\mathbb C)=({\mathbb C}^2-\{(0,0)\})/{\mathbb C}^\times $
, where
![]() ${\mathbb C}^\times $
acts as follows:
${\mathbb C}^\times $
acts as follows:
![]() $(z,w)c=(zc,wc)$
. This space is called the complex projective line. We denote the orbit in
$(z,w)c=(zc,wc)$
. This space is called the complex projective line. We denote the orbit in
![]() $P^1(\mathbb C)$
containing the pair
$P^1(\mathbb C)$
containing the pair
![]() $(z,w)$
by
$(z,w)$
by
![]() $[z,w]$
.
$[z,w]$
.
If groups L and R act on a set X on the left and on the right, respectively, then we say that the actions of L and R commute if
![]() $l(xr)=(lx)r$
for any
$l(xr)=(lx)r$
for any
![]() $l\in L$
,
$l\in L$
,
![]() $r\in R$
, and
$r\in R$
, and
![]() $x\in X$
. In this case, L acts on
$x\in X$
. In this case, L acts on
![]() $X/R$
on the left and R acts on
$X/R$
on the left and R acts on
![]() $L\mathop {\backslash } X$
on the right by
$L\mathop {\backslash } X$
on the right by
![]() $l(xR)=(lx)R$
and
$l(xR)=(lx)R$
and
![]() $(Lx)r=L(xr)$
, respectively.
$(Lx)r=L(xr)$
, respectively.
Suppose that G acts on the left on both X and E. Then G acts on the left on the product
![]() $X\times E$
diagonally:
$X\times E$
diagonally:
![]() $g(x,e)=(gx,ge)$
. We set
$g(x,e)=(gx,ge)$
. We set
We call the map
![]() $X\,{}_G\!\times \,E\to G\mathop {\backslash } E$
that maps any orbit
$X\,{}_G\!\times \,E\to G\mathop {\backslash } E$
that maps any orbit
![]() $G(x,e)$
to
$G(x,e)$
to
![]() $Ge$
the canonical projection. We denote canonical projections by
$Ge$
the canonical projection. We denote canonical projections by
![]() $\mathop {\mathbf {can}}\nolimits $
. If
$\mathop {\mathbf {can}}\nolimits $
. If
![]() $X'$
and
$X'$
and
![]() $E'$
are other sets endowed with left G-actions, and
$E'$
are other sets endowed with left G-actions, and
![]() $\alpha :X\to X'$
and
$\alpha :X\to X'$
and
![]() $\beta :E\to E'$
are G-equivariant maps, then we denote by
$\beta :E\to E'$
are G-equivariant maps, then we denote by
![]() $\alpha {}\,{}_G\!\times \beta $
the map from
$\alpha {}\,{}_G\!\times \beta $
the map from
![]() $X\,{}_G\!\times \,E$
to
$X\,{}_G\!\times \,E$
to
![]() $X'\,{}_G\!\times \,E'$
given by
$X'\,{}_G\!\times \,E'$
given by
![]() $G(x,e)\mapsto G(\alpha (x),\beta (e))$
.
$G(x,e)\mapsto G(\alpha (x),\beta (e))$
.
2.5 Quotient products
Let
![]() $X_1,\ldots ,X_n$
be topological spaces and
$X_1,\ldots ,X_n$
be topological spaces and
![]() $G_1,\ldots ,G_{n-1}$
be topological groups such that each
$G_1,\ldots ,G_{n-1}$
be topological groups such that each
![]() $G_i$
acts on the right on
$G_i$
acts on the right on
![]() $X_i$
and on the left on
$X_i$
and on the left on
![]() $X_{i+1}$
. Suppose additionally that the actions of
$X_{i+1}$
. Suppose additionally that the actions of
![]() $G_{i-1}$
and
$G_{i-1}$
and
![]() $G_i$
on
$G_i$
on
![]() $X_i$
commute for each
$X_i$
commute for each
![]() ${i=2,\ldots ,n-1}$
. Then we consider the following equivalence relation
${i=2,\ldots ,n-1}$
. Then we consider the following equivalence relation
![]() $\sim $
on
$\sim $
on
![]() ${X_1\times \cdots \times X_n}$
:
${X_1\times \cdots \times X_n}$
:
for any
![]() $g_1\in G_1$
, …,
$g_1\in G_1$
, …,
![]() $g_{n-1}\in G_{n-1}$
. The quotient product is the following quotient space:
$g_{n-1}\in G_{n-1}$
. The quotient product is the following quotient space:
If
![]() $n=0$
, this space is just the singleton and if
$n=0$
, this space is just the singleton and if
![]() $n=1$
, this space is
$n=1$
, this space is
![]() $X_1$
. The equivalence class containing
$X_1$
. The equivalence class containing
![]() $(x_1,x_2,\ldots ,x_n)$
is denoted by
$(x_1,x_2,\ldots ,x_n)$
is denoted by
![]() $[x_1:x_2:\cdots :x_n]$
. This product is clearly associative, that is,
$[x_1:x_2:\cdots :x_n]$
. This product is clearly associative, that is,
Moreover,
where we assume that
![]() $[x_1:\cdots :x_n]=[x_1:\cdots :x_i:1:x_{i+1}:\cdots :x_n]$
.
$[x_1:\cdots :x_n]=[x_1:\cdots :x_i:1:x_{i+1}:\cdots :x_n]$
.
If a group
![]() $G_0$
acts on the left on
$G_0$
acts on the left on
![]() $X_1$
so that this action commutes with the right action of
$X_1$
so that this action commutes with the right action of
![]() $G_1$
for
$G_1$
for
![]() $n>1$
, then
$n>1$
, then
![]() $G_0$
also acts on the left on the above quotient product by
$G_0$
also acts on the left on the above quotient product by
![]() $g_0[x_1:x_2:\cdots :x_n]=[g_0x_1:x_2:\cdots :x_n]$
. A similar fact is true if
$g_0[x_1:x_2:\cdots :x_n]=[g_0x_1:x_2:\cdots :x_n]$
. A similar fact is true if
![]() $G_n$
acts on
$G_n$
acts on
![]() $X_n$
on the right commuting with
$X_n$
on the right commuting with
![]() $G_{n-1}$
. In that case, we also consider the following quotient space:
$G_{n-1}$
. In that case, we also consider the following quotient space:
where the equivalence relation
![]() $\kern1pt{\approx}\kern1pt $
is given by
$\kern1pt{\approx}\kern1pt $
is given by
![]() $(x_1,\ldots ,x_n\kern-0.1pt)\kern1.3pt{\approx}\kern1.3pt (x_1g_1,g_1^{-1}x_2g_2,\ldots ,g_{n-1}^{-1}x_ng_n\kern-0.1pt)$
for any
$(x_1,\ldots ,x_n\kern-0.1pt)\kern1.3pt{\approx}\kern1.3pt (x_1g_1,g_1^{-1}x_2g_2,\ldots ,g_{n-1}^{-1}x_ng_n\kern-0.1pt)$
for any
![]() $g_1\in G_1, \ldots,$
$g_1\in G_1, \ldots,$
![]() $g_{n-1}\in G_{n-1}$
,
$g_{n-1}\in G_{n-1}$
,
![]() $g_n\in G_n$
. The equivalence class containing
$g_n\in G_n$
. The equivalence class containing
![]() $(x_1,x_2,\ldots ,x_n)$
is denoted by
$(x_1,x_2,\ldots ,x_n)$
is denoted by ![]() . In view of the isomorphism
. In view of the isomorphism
we assume ![]() . It follows from (2-2) that
. It follows from (2-2) that
where we assume that ![]() . We make this identification, when appropriate.
. We make this identification, when appropriate.
2.6 Topology
We are going to use the following topological result.
Proposition 2.1. Let X and Y be topological spaces and
![]() $f:X\to Y$
be a continuous bijection. Suppose that there exists an open covering
$f:X\to Y$
be a continuous bijection. Suppose that there exists an open covering
![]() $Y=\bigcup _{i\in I}U_i$
and continuous functions
$Y=\bigcup _{i\in I}U_i$
and continuous functions
![]() $g_i:U_i\to X$
for each
$g_i:U_i\to X$
for each
![]() $i\in I$
such that
$i\in I$
such that
![]() $fg_i(y)=y$
for any
$fg_i(y)=y$
for any
![]() $i\in I$
and
$i\in I$
and
![]() $y\in U_i$
. Then f is a homeomorphism.
$y\in U_i$
. Then f is a homeomorphism.
Proof. As the restrictions of
![]() $g_i$
and
$g_i$
and
![]() $g_j$
to
$g_j$
to
![]() $U_i\cap U_j$
coincide, we can glue these maps to the single continuous map g ([Reference DugundjiDu, Theorem III.9.4]). Then we get
$U_i\cap U_j$
coincide, we can glue these maps to the single continuous map g ([Reference DugundjiDu, Theorem III.9.4]). Then we get
![]() $fg=\mathrm {id}$
, whence
$fg=\mathrm {id}$
, whence
![]() $f^{-1}=g$
.
$f^{-1}=g$
.
In this paper, we use the following terminology: a topological space X is called simply connected if it is path-connected, locally path-connected, and for any continuous function on the unit circle
![]() $f:S^1\to X$
, there exists a continuous function
$f:S^1\to X$
, there exists a continuous function
![]() $g:D^1\to X$
on the closed unit disk such that
$g:D^1\to X$
on the closed unit disk such that
![]() $g|_{S^1}=f$
. Note that this definition deviates from the classical one in that we require local path-connectedness, which however is true for all cases we encounter (for example, topological manifolds).
$g|_{S^1}=f$
. Note that this definition deviates from the classical one in that we require local path-connectedness, which however is true for all cases we encounter (for example, topological manifolds).
Let E and B be G-spaces, and
![]() $p:E\to B$
be a continuous G-equivariant map. We say that p is a principal G-bundle if for each point
$p:E\to B$
be a continuous G-equivariant map. We say that p is a principal G-bundle if for each point
![]() $b\in B$
, there exist an open neighborhood
$b\in B$
, there exist an open neighborhood
![]() $U\subset B$
and a G-equivariant homeomorphism
$U\subset B$
and a G-equivariant homeomorphism
![]() $h:G\times U\stackrel \sim \to p^{-1}(U)$
for the left G-action or
$h:G\times U\stackrel \sim \to p^{-1}(U)$
for the left G-action or
![]() $h:U\times G\stackrel \sim \to p^{-1}(U)$
for the right G-action such that the respective diagram
$h:U\times G\stackrel \sim \to p^{-1}(U)$
for the right G-action such that the respective diagram

is commutative. The G-equivariance of h means that for any
![]() $u\in U$
and
$u\in U$
and
![]() $g_1,g_2\in G$
, there holds
$g_1,g_2\in G$
, there holds
![]() $h(g_1g_2,u)=g_1h(u,g_2)$
for the left G-action and
$h(g_1g_2,u)=g_1h(u,g_2)$
for the left G-action and
![]() $h(u,g_1g_2)=h(u,g_1)g_2$
for the right G-action.
$h(u,g_1g_2)=h(u,g_1)g_2$
for the right G-action.
Proposition 2.2 [Reference HusemoellerHu, Ch. 4, Proposition 5.3].
Let X and E be left G-spaces. Suppose that the quotient map
![]() $E\to G\mathop {\backslash } E$
is a principal G-bundle. Then the canonical projection
$E\to G\mathop {\backslash } E$
is a principal G-bundle. Then the canonical projection
![]() $X\,{}_G\!\times \,E\to G\mathop {\backslash } E$
is a fiber bundle with fiber X.
$X\,{}_G\!\times \,E\to G\mathop {\backslash } E$
is a fiber bundle with fiber X.
Proposition 2.3. Let X and Y be right and left G-spaces, respectively. Let, moreover, H act continuously on Y on the right so that this action and the left action of G commute. Suppose that the quotient maps
![]() $X\to X/G$
and
$X\to X/G$
and
![]() $Y\to Y/H$
are principal G- and H-bundles, respectively. Then the quotient map
$Y\to Y/H$
are principal G- and H-bundles, respectively. Then the quotient map
![]() $f:X\mathop {\times }\limits _G Y\to X\mathop {\times }\limits _G Y/H$
is a principal H-bundle.
$f:X\mathop {\times }\limits _G Y\to X\mathop {\times }\limits _G Y/H$
is a principal H-bundle.
Proof. We use the notation of Section 2.5. Thus, ![]() . We also denote the quotient maps
. We also denote the quotient maps
![]() $X\to X/G$
and
$X\to X/G$
and
![]() $Y\to Y/H$
by p and q, respectively.
$Y\to Y/H$
by p and q, respectively.
Let ![]() be an arbitrary point of
be an arbitrary point of
![]() $X\mathop {\times }\limits _G Y/H$
. By the hypothesis of the lemma, there exist an open neighborhood U of
$X\mathop {\times }\limits _G Y/H$
. By the hypothesis of the lemma, there exist an open neighborhood U of
![]() $x_0G$
and a G-equivariant homeomorphism
$x_0G$
and a G-equivariant homeomorphism
![]() $\sigma $
such that the diagram
$\sigma $
such that the diagram

is commutative. Let
![]() $\mathbf g=\mathop{\rm pr}\nolimits _2\sigma ^{-1}$
. This is a continuous map from
$\mathbf g=\mathop{\rm pr}\nolimits _2\sigma ^{-1}$
. This is a continuous map from
![]() $p^{-1}(U)$
to G such that
$p^{-1}(U)$
to G such that
![]() $\mathbf g(xg)=\mathbf g(x)g$
for any
$\mathbf g(xg)=\mathbf g(x)g$
for any
![]() $x\in p^{-1}(U)$
and
$x\in p^{-1}(U)$
and
![]() $g\in G$
.
$g\in G$
.
There exists an open neighborhood V of
![]() $\mathbf g(x_0)y_0H$
and an H-equivariant homeomorphism
$\mathbf g(x_0)y_0H$
and an H-equivariant homeomorphism
![]() $\tau $
such that the diagram
$\tau $
such that the diagram

is commutative. Let
![]() $\mathbf h=\mathop{\rm pr}\nolimits _2\tau ^{-1}$
. This is a continuous map from
$\mathbf h=\mathop{\rm pr}\nolimits _2\tau ^{-1}$
. This is a continuous map from
![]() $q^{-1}(V)$
to H such that
$q^{-1}(V)$
to H such that
![]() $\mathbf h(yh)=\mathbf h(y)h$
for any
$\mathbf h(yh)=\mathbf h(y)h$
for any
![]() $y\in q^{-1}(V)$
and
$y\in q^{-1}(V)$
and
![]() $h\in H$
.
$h\in H$
.
Let
![]() $\lambda :X\times Y/H\to X/G$
be the map given by
$\lambda :X\times Y/H\to X/G$
be the map given by ![]() and
and
![]() ${\mu :\lambda ^{-1}(U)\to Y/H}$
be the map given by
${\mu :\lambda ^{-1}(U)\to Y/H}$
be the map given by ![]() . Obviously, both these maps are well defined and continuous. We set
. Obviously, both these maps are well defined and continuous. We set
![]() $W=\mu ^{-1}(V)$
. By our choice of U and V, we get
$W=\mu ^{-1}(V)$
. By our choice of U and V, we get ![]() . We define the function
. We define the function
![]() $\xi :W\times H\to X\mathop {\times }\limits _G Y$
by
$\xi :W\times H\to X\mathop {\times }\limits _G Y$
by ![]() One can easily check that this map is well defined, continuous, and H-equivariant. Composing it with f,
One can easily check that this map is well defined, continuous, and H-equivariant. Composing it with f,
This calculation proves that
![]() $\xi $
is actually a map to
$\xi $
is actually a map to
![]() $f^{-1}(W)$
and that the following diagram is commutative:
$f^{-1}(W)$
and that the following diagram is commutative:

It remains to prove that
![]() $\xi $
is a homeomorphism. This is true, as the inverse map is given by
$\xi $
is a homeomorphism. This is true, as the inverse map is given by ![]()
2.7 Equivariant cohomology
Let G be a topological group. A principal G-bundle
![]() $E\to B$
is called universal if the total space E is contractible. We define the G-equivariant cohomology of a left G-space X with coefficients in a commutative ring
$E\to B$
is called universal if the total space E is contractible. We define the G-equivariant cohomology of a left G-space X with coefficients in a commutative ring
![]() $\Bbbk $
by
$\Bbbk $
by
Considering the canonical projection
![]() $\mathop {\mathbf {can}}\nolimits :X\,{}_G\!\times \,E\to G\mathop {\backslash } E\cong \mathrm {pt}\,{}_G\!\times \,E$
, we obtain the map
$\mathop {\mathbf {can}}\nolimits :X\,{}_G\!\times \,E\to G\mathop {\backslash } E\cong \mathrm {pt}\,{}_G\!\times \,E$
, we obtain the map
![]() $\mathop {\mathbf {can}}\nolimits ^*:H^{\bullet }_G(\mathrm {pt},\Bbbk )\to H^{\bullet }_G(X,\Bbbk )$
. This map makes
$\mathop {\mathbf {can}}\nolimits ^*:H^{\bullet }_G(\mathrm {pt},\Bbbk )\to H^{\bullet }_G(X,\Bbbk )$
. This map makes
![]() $H^{\bullet }_G(X,\Bbbk )$
into a left
$H^{\bullet }_G(X,\Bbbk )$
into a left
![]() $H^{\bullet }_G(\mathrm {pt},\Bbbk )$
-module by
$H^{\bullet }_G(\mathrm {pt},\Bbbk )$
-module by
where
![]() $a\in H^{\bullet }_G(\mathrm {pt},\Bbbk )$
and
$a\in H^{\bullet }_G(\mathrm {pt},\Bbbk )$
and
![]() $m\in H^{\bullet }_G(X,\Bbbk )$
. This module does not depend on the choice of the universal bundle
$m\in H^{\bullet }_G(X,\Bbbk )$
. This module does not depend on the choice of the universal bundle
![]() $E\to B$
. This fact is discussed in Corollary 3.2. We call this left action of
$E\to B$
. This fact is discussed in Corollary 3.2. We call this left action of
![]() $H^{\bullet }_G(\mathrm {pt},\Bbbk )$
canonical.
$H^{\bullet }_G(\mathrm {pt},\Bbbk )$
canonical.
The similar right action of
![]() $H^{\bullet }_G(\mathrm {pt},\Bbbk )$
on
$H^{\bullet }_G(\mathrm {pt},\Bbbk )$
on
![]() $H^{\bullet }_G(X,\Bbbk )$
given by
$H^{\bullet }_G(X,\Bbbk )$
given by
![]() $ma=m\cup \mathop {\mathbf {can}}\nolimits ^*(a)$
is also called canonical. We use it in Section 4.6.
$ma=m\cup \mathop {\mathbf {can}}\nolimits ^*(a)$
is also called canonical. We use it in Section 4.6.
If
![]() $X'$
is another left G-space and
$X'$
is another left G-space and
![]() $f:X\to X'$
is a continuous G-equivariant map, then we have the continuous map
$f:X\to X'$
is a continuous G-equivariant map, then we have the continuous map
![]() $f\,{}_G\!\times \,\mathrm {id}:X\,{}_G\!\times \,E\to X'\,{}_G\!\times \,E$
. This map induces the pull-back
$f\,{}_G\!\times \,\mathrm {id}:X\,{}_G\!\times \,E\to X'\,{}_G\!\times \,E$
. This map induces the pull-back
between cohomologies. We use the notation
![]() $f^{\star }=(f\,{}_G\!\times \,\mathrm {id})^*$
to distinguish between the ordinary and the equivariant pull-backs. For example,
$f^{\star }=(f\,{}_G\!\times \,\mathrm {id})^*$
to distinguish between the ordinary and the equivariant pull-backs. For example,
![]() $a_X^{\star }=\mathop {\mathbf {can}}\nolimits ^*$
, where
$a_X^{\star }=\mathop {\mathbf {can}}\nolimits ^*$
, where
![]() ${a_X:X\to \mathrm {pt}}$
is the constant map.
${a_X:X\to \mathrm {pt}}$
is the constant map.
2.8 Stiefel manifolds
Let G be a compact Lie group. As G can be considered a closed subgroup of the unitary group
![]() $U({\mathfrak n}_{G})$
for
$U({\mathfrak n}_{G})$
for
![]() ${\mathfrak n}_{G}$
big enough which will be thought of as a function of
${\mathfrak n}_{G}$
big enough which will be thought of as a function of
![]() $G$
throughout the paper, we explain how to find a universal principal
$G$
throughout the paper, we explain how to find a universal principal
![]() $U({\mathfrak n}_{G})$
-bundle. For any natural number
$U({\mathfrak n}_{G})$
-bundle. For any natural number
![]() $N>{\mathfrak n}_{G}$
, we consider the Stiefel manifold (this space is usually denoted by
$N>{\mathfrak n}_{G}$
, we consider the Stiefel manifold (this space is usually denoted by
![]() $W_{N,{\mathfrak n}_{G}}$
or
$W_{N,{\mathfrak n}_{G}}$
or
![]() $V_{{\mathfrak n}_{G}}({\mathbb C}^N)$
or
$V_{{\mathfrak n}_{G}}({\mathbb C}^N)$
or
![]() ${\mathbb C} V_{N,{{\mathfrak n}_{G}}}$
. We also transpose matrices, as we want to have a left action of
${\mathbb C} V_{N,{{\mathfrak n}_{G}}}$
. We also transpose matrices, as we want to have a left action of
![]() $U({\mathfrak n}_{G})$
)
$U({\mathfrak n}_{G})$
)
where
![]() $\mathop{\rm Mat}\nolimits _{{{\mathfrak n}_{G}},N}({\mathbb C})$
is the space of
$\mathop{\rm Mat}\nolimits _{{{\mathfrak n}_{G}},N}({\mathbb C})$
is the space of
![]() ${\mathfrak n}_{G}\times N$
complex matrices with respect to the metric topology and
${\mathfrak n}_{G}\times N$
complex matrices with respect to the metric topology and
![]() $I_{{\mathfrak n}_{G}}$
is the identity
$I_{{\mathfrak n}_{G}}$
is the identity
![]() ${\mathfrak n}_{G}\times {\mathfrak n}_{G}$
matrix. The group
${\mathfrak n}_{G}\times {\mathfrak n}_{G}$
matrix. The group
![]() $U({\mathfrak n}_{G})$
acts on
$U({\mathfrak n}_{G})$
acts on
![]() $E^N$
on the left by multiplication. Similarly,
$E^N$
on the left by multiplication. Similarly,
![]() $U(N)$
acts on
$U(N)$
acts on
![]() $E^N$
on the right by multiplication. The last action is transitive and commutes with the left action of
$E^N$
on the right by multiplication. The last action is transitive and commutes with the left action of
![]() $U({\mathfrak n}_{G})$
. The quotient space
$U({\mathfrak n}_{G})$
. The quotient space
![]() $\mathop{\rm Gr}\nolimits ^N=U({\mathfrak n}_{G})\mathop {\backslash } E^N$
is called the Grassmanian and the corresponding quotient map
$\mathop{\rm Gr}\nolimits ^N=U({\mathfrak n}_{G})\mathop {\backslash } E^N$
is called the Grassmanian and the corresponding quotient map
![]() ${E^N\to \mathop{\rm Gr}\nolimits ^N}$
is a principal
${E^N\to \mathop{\rm Gr}\nolimits ^N}$
is a principal
![]() $U({\mathfrak n}_{G})$
-bundle. Note that the group
$U({\mathfrak n}_{G})$
-bundle. Note that the group
![]() $U(N)$
also acts on
$U(N)$
also acts on
![]() $\mathop{\rm Gr}\nolimits ^N$
by the right multiplication. For
$\mathop{\rm Gr}\nolimits ^N$
by the right multiplication. For
![]() $N<N'$
, we have the smooth embedding
$N<N'$
, we have the smooth embedding
![]() $E^N\hookrightarrow E^{N'}$
by adding
$E^N\hookrightarrow E^{N'}$
by adding
![]() $N'-N$
zero columns to the right.
$N'-N$
zero columns to the right.
Taking the direct limits
we get a universal principal
![]() $U({\mathfrak n}_{G})$
-bundle
$U({\mathfrak n}_{G})$
-bundle
![]() $E\to \mathop{\rm Gr}\nolimits $
. These spaces satisfy the following properties [Reference BorelBo, Proposition 9.1].
$E\to \mathop{\rm Gr}\nolimits $
. These spaces satisfy the following properties [Reference BorelBo, Proposition 9.1].
Proposition 2.4
-
(1)
 $E^N$
is simply connected.
$E^N$
is simply connected. -
(2)
 $H^n(E^N,\Bbbk )=0$
for
$H^n(E^N,\Bbbk )=0$
for
 $0<n\leqslant 2(N-{\mathfrak n}_{G})$
.
$0<n\leqslant 2(N-{\mathfrak n}_{G})$
. -
(3)
 $H^n(E^N,\Bbbk )$
is free of finite rank for any n.
$H^n(E^N,\Bbbk )$
is free of finite rank for any n.
Corollary 2.5. For any closed connected subgroup L of G, the space
![]() $L\mathop {\backslash } E^N$
is simply connected.
$L\mathop {\backslash } E^N$
is simply connected.
Proof. As
![]() $E^N\to L\mathop {\backslash } E^N$
is a fiber bundle with fiber L, the result follows from Propositions 2.4, (1), and the long exact sequence of homotopy groups
$E^N\to L\mathop {\backslash } E^N$
is a fiber bundle with fiber L, the result follows from Propositions 2.4, (1), and the long exact sequence of homotopy groups
Proposition 2.6. Let L be a closed subgroup of G and X be a left L-space. Then the restriction map
is an isomorphism for
![]() $n\leqslant 2(N-{\mathfrak n}_{G})$
.
$n\leqslant 2(N-{\mathfrak n}_{G})$
.
Proof. Let us consider the triple product
![]() $X\times E^N\times E$
with respect to the diagonal action of L, the natural embedding
$X\times E^N\times E$
with respect to the diagonal action of L, the natural embedding
![]() $\iota :X\,{}_L\!\times E^N\hookrightarrow X\,{}_L\!\times E$
, and the map
$\iota :X\,{}_L\!\times E^N\hookrightarrow X\,{}_L\!\times E$
, and the map
![]() $\Delta :X\times E^N\to X\times E^N\times E$
given by
$\Delta :X\times E^N\to X\times E^N\times E$
given by
![]() $(x,e)\mapsto (x,e,e)$
. Then we have the following commutative diagram:
$(x,e)\mapsto (x,e,e)$
. Then we have the following commutative diagram:

Here, the isomorphisms follow from Propositions 2.4, (2), and the Vietoris–Begle theorem in the form [Reference ShchigolevS2, Corollary 14], as the quotient maps
are fiber bundles with fibers E and
![]() $E^N$
, respectively. From the left triangle, it follows that
$E^N$
, respectively. From the left triangle, it follows that
![]() $(L\mathop {\backslash } \Delta )^*$
is an isomorphism. Therefore, it follows from the right triangle that
$(L\mathop {\backslash } \Delta )^*$
is an isomorphism. Therefore, it follows from the right triangle that
![]() $\iota ^*$
is also an isomorphism.
$\iota ^*$
is also an isomorphism.
Corollary 2.7. Let L be a closed subgroup of G, X be a left L-space, and
![]() ${{\mathfrak n}_{G}<N' <N}$
. Then the restriction map
${{\mathfrak n}_{G}<N' <N}$
. Then the restriction map
is an isomorphism for
![]() $n\leqslant 2(N-{\mathfrak n}_{G})$
.
$n\leqslant 2(N-{\mathfrak n}_{G})$
.
Remark 2.8. We always mean the choice of
![]() $E$
as the direct limit of
$E$
as the direct limit of
![]() $E^N$
in the remainder of the paper. If, however, we replace it by
$E^N$
in the remainder of the paper. If, however, we replace it by
![]() $E^N$
, then we write
$E^N$
, then we write
![]() $f^N$
instead of f, where f is either a map between spaces whose definitions depend on E or a subset of such a space. We refer to
$f^N$
instead of f, where f is either a map between spaces whose definitions depend on E or a subset of such a space. We refer to
![]() $f^N$
as a compact version of f.
$f^N$
as a compact version of f.
2.9 Ordinary push-forward
For any topological space X, we consider the dualizing complex
![]() $\omega _X=a_X^!\Bbbk $
[Reference Kashiwara and SchapiraKS, Definition 3.1.16]. This complex allows us to define the Borel–Moore homology
$\omega _X=a_X^!\Bbbk $
[Reference Kashiwara and SchapiraKS, Definition 3.1.16]. This complex allows us to define the Borel–Moore homology
![]() $H^{\mathrm {BM}}_i(X,\Bbbk )={\mathbb H}^{-i}(X,\omega _X)$
, where
$H^{\mathrm {BM}}_i(X,\Bbbk )={\mathbb H}^{-i}(X,\omega _X)$
, where
![]() ${\mathbb H}^{\bullet }(X,\_\!\_\,)$
is the hypercohomology functor [Reference DimcaDi, Definition 2.1.4].
${\mathbb H}^{\bullet }(X,\_\!\_\,)$
is the hypercohomology functor [Reference DimcaDi, Definition 2.1.4].
Any proper map
![]() $f:X\to Y$
induces the push-forward map
$f:X\to Y$
induces the push-forward map
![]() $f_*:H^{\mathrm {BM}}_i(X,\Bbbk )\to H^{\mathrm {BM}}_i(Y,\Bbbk )$
. Suppose additionally that X and Y are orientable topological manifolds of dimensions n and m, respectively. This means that there exist isomorphisms
$f_*:H^{\mathrm {BM}}_i(X,\Bbbk )\to H^{\mathrm {BM}}_i(Y,\Bbbk )$
. Suppose additionally that X and Y are orientable topological manifolds of dimensions n and m, respectively. This means that there exist isomorphisms
![]() $\omega _X\cong \Bbbk [n]$
and
$\omega _X\cong \Bbbk [n]$
and
![]() $\omega _Y\cong \Bbbk [m]$
, which we fix. Then we get the following map between the cohomologies:
$\omega _Y\cong \Bbbk [m]$
, which we fix. Then we get the following map between the cohomologies:
which we also denote by
![]() $f_*$
. This map is also called the Gysin map. It has the following property [Reference IversenI, IX.7.3].
$f_*$
. This map is also called the Gysin map. It has the following property [Reference IversenI, IX.7.3].
Proposition 2.9 (Projection formula).
For any proper continuous map
![]() $f:X\to Y$
between oriented topological manifolds,
$f:X\to Y$
between oriented topological manifolds,
![]() $\xi \in H^{\bullet }(X,\Bbbk )$
and
$\xi \in H^{\bullet }(X,\Bbbk )$
and
![]() $\eta \in H^{\bullet }(Y,\Bbbk )$
, there holds
$\eta \in H^{\bullet }(Y,\Bbbk )$
, there holds
2.10 Orientation of quotient spaces
Let U be an oriented subspace of an oriented real vector space V. We fix the following orientation of the quotient space
![]() $V/U$
. Let
$V/U$
. Let
![]() $v_1,\ldots ,v_n$
be an oriented basis of V such that
$v_1,\ldots ,v_n$
be an oriented basis of V such that
![]() $v_1,\ldots ,v_m$
is an oriented basis of U for some
$v_1,\ldots ,v_m$
is an oriented basis of U for some
![]() $m\leqslant n$
. Then the orientation of
$m\leqslant n$
. Then the orientation of
![]() $V/U$
is defined by declaring the basis
$V/U$
is defined by declaring the basis
![]() ${v_{m+1}+U,\ldots ,v_n+U}$
to be oriented. We define the orientation of the quotient of oriented vector bundles by defining the orientation of each fiber using the rule just described.
${v_{m+1}+U,\ldots ,v_n+U}$
to be oriented. We define the orientation of the quotient of oriented vector bundles by defining the orientation of each fiber using the rule just described.
2.11 Compatibly oriented squares
We follow here the presentation of this topic by Fulton [Reference FultonF, Appendix A: Algebraic topology, Section 5]. For an embedding
![]() $X\hookrightarrow Y$
of smooth manifolds, we denote by
$X\hookrightarrow Y$
of smooth manifolds, we denote by
![]() $\nu _{X,Y}$
the normal bundle of X in Y. If X and Y are oriented, then
$\nu _{X,Y}$
the normal bundle of X in Y. If X and Y are oriented, then
![]() $\nu _{X,Y}$
is orientated as described in Section 2.10.
$\nu _{X,Y}$
is orientated as described in Section 2.10.
Consider a Cartesian square

where
![]() $X,X',Y,Y'$
are compact smooth oriented manifolds and
$X,X',Y,Y'$
are compact smooth oriented manifolds and
![]() $f,f',g,g'$
are smooth maps. By the Whitney embedding theorem (for example, [Reference LeeLee, Theorem 6.15]), there exists a closed embedding
$f,f',g,g'$
are smooth maps. By the Whitney embedding theorem (for example, [Reference LeeLee, Theorem 6.15]), there exists a closed embedding
![]() $\iota :X\hookrightarrow {\mathbb R}^M$
, where
$\iota :X\hookrightarrow {\mathbb R}^M$
, where
![]() $M=2\dim X+1$
. Then we get the closed embedding
$M=2\dim X+1$
. Then we get the closed embedding
![]() $f\times \iota :X\hookrightarrow Y\times {\mathbb R}^M$
and also the closed embedding
$f\times \iota :X\hookrightarrow Y\times {\mathbb R}^M$
and also the closed embedding
![]() $f'\times \iota g':X'\hookrightarrow Y'\times {\mathbb R}^M$
.
$f'\times \iota g':X'\hookrightarrow Y'\times {\mathbb R}^M$
.
We say that the above square is compatibly oriented if
as oriented vector bundles. If this isomorphism holds and
![]() $\dim X-\dim Y=\dim X'-\dim Y'$
, then
$\dim X-\dim Y=\dim X'-\dim Y'$
, then
We refer to this property as the naturality of the push-forward.
2.12 Smooth structures on Borel constructions
Let L be a closed connected subgroup of a compact Lie group G. By the quotient manifold theorem [Reference LeeLee, Theorem 21.10], the space
![]() $L\mathop {\backslash } E^N$
is a smooth manifold. It is orientable being simply connected by Corollary 2.5. Therefore, we consider only local coordinates on
$L\mathop {\backslash } E^N$
is a smooth manifold. It is orientable being simply connected by Corollary 2.5. Therefore, we consider only local coordinates on
![]() $L\mathop {\backslash } E^N$
from a chosen oriented atlas.
$L\mathop {\backslash } E^N$
from a chosen oriented atlas.
Let X be any smooth manifold acted upon smoothly by L on the left. Applying the quotient manifold theorem once again, we get that the space
![]() $X\;{}_L\! \times E^N$
is a smooth manifold. Its smooth structure can be described as follows. Let
$X\;{}_L\! \times E^N$
is a smooth manifold. Its smooth structure can be described as follows. Let
![]() $L(x_0,e_0)$
be an arbitrary point of
$L(x_0,e_0)$
be an arbitrary point of
![]() $X\;{}_L\! \times E^N$
. There exists a coordinate chart U of
$X\;{}_L\! \times E^N$
. There exists a coordinate chart U of
![]() $L\mathop {\backslash } E^N$
containing
$L\mathop {\backslash } E^N$
containing
![]() $Le_0$
. Let
$Le_0$
. Let
![]() $(u^1,\ldots ,u^k)$
be the coordinates on U.
$(u^1,\ldots ,u^k)$
be the coordinates on U.
Shrinking U if necessary, we may suppose that the restriction of the fiber bundle
![]() $p:E^N\to L\mathop {\backslash } E^N$
to U is a trivial bundle, that is, there exists an L-equivariant diffeomorphism h such that the diagram
$p:E^N\to L\mathop {\backslash } E^N$
to U is a trivial bundle, that is, there exists an L-equivariant diffeomorphism h such that the diagram

is commutative. We set
![]() ${\mathbf l}=\mathop{\rm pr}\nolimits _1h^{-1}$
. This map is smooth and has the usual (refer to the proof of Proposition 2.3) property
${\mathbf l}=\mathop{\rm pr}\nolimits _1h^{-1}$
. This map is smooth and has the usual (refer to the proof of Proposition 2.3) property
![]() ${\mathbf l}(le)=l{\mathbf l}(e)$
for any
${\mathbf l}(le)=l{\mathbf l}(e)$
for any
![]() $l\in L$
and
$l\in L$
and
![]() $e\in p^{-1}(U)$
.
$e\in p^{-1}(U)$
.
The restriction of the fiber bundle
![]() $\mathop {\mathbf {can}}\nolimits :X\;{}_L\! \times E^N\to L\mathop {\backslash } E^N$
to U is also trivial. This trivialization is given by
$\mathop {\mathbf {can}}\nolimits :X\;{}_L\! \times E^N\to L\mathop {\backslash } E^N$
to U is also trivial. This trivialization is given by
![]() $L(x,e)\mapsto ({\mathbf l}(e)^{-1}x,Le)$
. Then the coordinates in the vicinity of
$L(x,e)\mapsto ({\mathbf l}(e)^{-1}x,Le)$
. Then the coordinates in the vicinity of
![]() $L(x_0,e_0)$
are given by the local coordinates
$L(x_0,e_0)$
are given by the local coordinates
![]() $(x^1,\ldots ,x^n,u^1,\ldots ,u^k)$
, where
$(x^1,\ldots ,x^n,u^1,\ldots ,u^k)$
, where
![]() $(x^1,\ldots ,x^n)$
are the local coordinates on X in the vicinity of
$(x^1,\ldots ,x^n)$
are the local coordinates on X in the vicinity of
![]() ${\mathbf l}(e_0)^{-1}x_0$
. If
${\mathbf l}(e_0)^{-1}x_0$
. If
![]() $(\tilde x^1,\ldots ,\tilde x^n,\tilde u^1,\ldots ,\tilde u^k)$
are different local coordinates in a vicinity of
$(\tilde x^1,\ldots ,\tilde x^n,\tilde u^1,\ldots ,\tilde u^k)$
are different local coordinates in a vicinity of
![]() $L(x_0,e_0)$
with a possibly different trivialization function
$L(x_0,e_0)$
with a possibly different trivialization function
![]() $\tilde {\mathbf l}$
, then the Jacobian matrix of the base change is equal to
$\tilde {\mathbf l}$
, then the Jacobian matrix of the base change is equal to
 $$ \begin{align*} \frac{\partial(\tilde x^1,\ldots,\tilde x^n,\tilde u^1,\ldots,\tilde u^k)}{\partial(x^1,\ldots,x^n,u^1,\ldots,u^k)}(L(x,e)) = \left( \begin{array}{@{}cc@{}} \dfrac{\partial(\tilde f^1,\ldots,\tilde f^n)}{\partial(x^1,\ldots,x^n)}({\mathbf l}(e)^{-1}x)&0\\[8pt] *& \dfrac{\partial(\tilde u^1,\ldots,\tilde u^k)}{\partial(u^1,\ldots,u^k)}(Le) \end{array} \right), \end{align*} $$
$$ \begin{align*} \frac{\partial(\tilde x^1,\ldots,\tilde x^n,\tilde u^1,\ldots,\tilde u^k)}{\partial(x^1,\ldots,x^n,u^1,\ldots,u^k)}(L(x,e)) = \left( \begin{array}{@{}cc@{}} \dfrac{\partial(\tilde f^1,\ldots,\tilde f^n)}{\partial(x^1,\ldots,x^n)}({\mathbf l}(e)^{-1}x)&0\\[8pt] *& \dfrac{\partial(\tilde u^1,\ldots,\tilde u^k)}{\partial(u^1,\ldots,u^k)}(Le) \end{array} \right), \end{align*} $$
where f is the map
![]() $f(x)=\tilde {\mathbf l}(e)^{-1}{\mathbf l}(e)x$
and
$f(x)=\tilde {\mathbf l}(e)^{-1}{\mathbf l}(e)x$
and
![]() $\tilde f^i=\tilde x^if$
. Note that the multiplication constant
$\tilde f^i=\tilde x^if$
. Note that the multiplication constant
![]() $\tilde {\mathbf l}(e)^{-1}{\mathbf l}(e)$
smoothly depends only on
$\tilde {\mathbf l}(e)^{-1}{\mathbf l}(e)$
smoothly depends only on
![]() $Le$
. The multiplication by this constant is responsible to the
$Le$
. The multiplication by this constant is responsible to the
![]() $*$
-part in the above matrix. This part is the zero matrix if we do not change the local trivialization of
$*$
-part in the above matrix. This part is the zero matrix if we do not change the local trivialization of
![]() $E^N\to L\mathop {\backslash } E^N$
.
$E^N\to L\mathop {\backslash } E^N$
.
If X is oriented, then L acts on X by orientation-preserving diffeomorphisms. Hence,
 $$ \begin{align*} \det\frac{\partial(\tilde f^1,\ldots,\tilde f^n)}{\partial(x^1,\ldots,x^n)}({\mathbf l}(e)^{-1}x)>0 \end{align*} $$
$$ \begin{align*} \det\frac{\partial(\tilde f^1,\ldots,\tilde f^n)}{\partial(x^1,\ldots,x^n)}({\mathbf l}(e)^{-1}x)>0 \end{align*} $$
and the atlas described above on
![]() $X\;{}_L\! \times E^N$
is oriented.
$X\;{}_L\! \times E^N$
is oriented.
2.13 Equivariant push-forward
Let X and Y be compact smooth oriented manifolds acted upon smoothly on the left by a closed connected subgroup L of a compact Lie group G. Let
![]() $f:Y\to X$
be a smooth L-equivariant map. For any
$f:Y\to X$
be a smooth L-equivariant map. For any
![]() $N>{\mathfrak n}_{G}$
, we get the smooth proper map
$N>{\mathfrak n}_{G}$
, we get the smooth proper map
![]() $f\;{}_L\! \times \mathrm {id}:Y\;{}_L\! \times E^N\to X\;{}_L\! \times E^N$
, which we denote by
$f\;{}_L\! \times \mathrm {id}:Y\;{}_L\! \times E^N\to X\;{}_L\! \times E^N$
, which we denote by
![]() $f^N$
. As both the domain and codomain of this map are smooth oriented manifolds, we can consider the ordinary push-forward
$f^N$
. As both the domain and codomain of this map are smooth oriented manifolds, we can consider the ordinary push-forward
where
![]() $d=\dim X-\dim Y$
. So by Proposition 2.6, we actually get the map
$d=\dim X-\dim Y$
. So by Proposition 2.6, we actually get the map
as soon as
![]() $n\leqslant \min \{2(N-{\mathfrak n}_{G}),2(N-{\mathfrak n}_{G})-d\}$
, defined by
$n\leqslant \min \{2(N-{\mathfrak n}_{G}),2(N-{\mathfrak n}_{G})-d\}$
, defined by
for
![]() $m\in H^n_L(Y,\Bbbk )$
, where
$m\in H^n_L(Y,\Bbbk )$
, where
![]() $i_X:X\;{}_L\! \times E^N\hookrightarrow X\;{}_L\! \times E$
and
$i_X:X\;{}_L\! \times E^N\hookrightarrow X\;{}_L\! \times E$
and
![]() $i_Y:Y\;{}_L\! \times E^N\hookrightarrow Y\;{}_L\! \times E$
are the natural embeddings.
$i_Y:Y\;{}_L\! \times E^N\hookrightarrow Y\;{}_L\! \times E$
are the natural embeddings.
We claim that the definition of
![]() $f_\star $
does not depend of N. Indeed, let
$f_\star $
does not depend of N. Indeed, let
![]() $N'>N$
. In this case, we have the map
$N'>N$
. In this case, we have the map
![]() $f^{\prime }_\star $
defined by a formula similar to (2-5). To prove that
$f^{\prime }_\star $
defined by a formula similar to (2-5). To prove that
![]() $f^{\prime }_\star =f_\star $
, we consider the diagram
$f^{\prime }_\star =f_\star $
, we consider the diagram

As both triangles and trapezoids are commutative, it suffices to prove that the perimeter is also so. This fact follows from (2-4) as soon as we prove that the square

is compatibly oriented.
First note that the embedding
![]() $E^N\hookrightarrow E^{N'}$
produces an embedding
$E^N\hookrightarrow E^{N'}$
produces an embedding
![]() $L\mathop {\backslash } E^N\hookrightarrow L\mathop {\backslash } E^{N'}$
. Therefore, we always choose the coordinates
$L\mathop {\backslash } E^N\hookrightarrow L\mathop {\backslash } E^{N'}$
. Therefore, we always choose the coordinates
![]() $(u^1,\ldots ,u^{k'})$
on an open subspace
$(u^1,\ldots ,u^{k'})$
on an open subspace
![]() ${U'\subset L\mathop {\backslash } E^{N'}}$
so that the intersection
${U'\subset L\mathop {\backslash } E^{N'}}$
so that the intersection
![]() $U'\cap E^N$
is defined by the equalities
$U'\cap E^N$
is defined by the equalities
![]() ${u^{k+1}=0,\ldots ,u^{k'}=0}$
. Shrinking
${u^{k+1}=0,\ldots ,u^{k'}=0}$
. Shrinking
![]() $U'$
if necessary, we assume that the fiber bundle
$U'$
if necessary, we assume that the fiber bundle
![]() $p':E^{N'}\to L\mathop {\backslash } E^{N'}$
is trivial and consider the trivialization function
$p':E^{N'}\to L\mathop {\backslash } E^{N'}$
is trivial and consider the trivialization function
![]() ${\mathbf l}':(p')^{-1}(U')\to L$
as in Section 2.12.
${\mathbf l}':(p')^{-1}(U')\to L$
as in Section 2.12.
Let
![]() $\iota :Y\;{}_L\! \times E^{N'}\hookrightarrow {\mathbb R}^M$
be an embedding. We denote by
$\iota :Y\;{}_L\! \times E^{N'}\hookrightarrow {\mathbb R}^M$
be an embedding. We denote by
![]() $x^1,\ldots ,x^n$
,
$x^1,\ldots ,x^n$
,
![]() $y^1,\ldots ,y^m$
, and
$y^1,\ldots ,y^m$
, and
![]() $z^1,\ldots ,z^M$
local coordinates on X, Y, and
$z^1,\ldots ,z^M$
local coordinates on X, Y, and
![]() ${\mathbb R}^M$
, respectively.
${\mathbb R}^M$
, respectively.
We abbreviate
According to the general plan described in Section 2.11, we need to consider the embedding
![]() $ \beta '=f^{N'}\times \iota :Y\;{}_L\! \times E^{N'}\hookrightarrow \mathbb B' $
, and its pullback
$ \beta '=f^{N'}\times \iota :Y\;{}_L\! \times E^{N'}\hookrightarrow \mathbb B' $
, and its pullback
![]() $ \beta :Y\;{}_L\! \times E^N\hookrightarrow \mathbb B $
being in our case simply the restriction of
$ \beta :Y\;{}_L\! \times E^N\hookrightarrow \mathbb B $
being in our case simply the restriction of
![]() $\beta '$
, that is,
$\beta '$
, that is,
![]() $\beta =f^N\times \iota |_{Y{}_L\! \times E^N}$
.
$\beta =f^N\times \iota |_{Y{}_L\! \times E^N}$
.
Let
![]() $a\in Y{}_L\! \times E^N$
be an arbitrary point. We set
$a\in Y{}_L\! \times E^N$
be an arbitrary point. We set
![]() $b=\beta (a)=\beta '(a)$
. Choosing the representation
$b=\beta (a)=\beta '(a)$
. Choosing the representation
![]() $a=L(y,e)$
with
$a=L(y,e)$
with
![]() $\mathbf l'(e)=1$
, we compute the differential
$\mathbf l'(e)=1$
, we compute the differential
in coordinates as follows:
 $$ \begin{align} T_a\beta'\bigg(\frac{\partial}{\partial y^i}\bigg)=\sum_{j=1}^n\frac{\partial f^j}{\partial y^i}(y)\frac{\partial}{\partial x^j}+\sum_{q=1}^M\frac{\partial\iota^q}{\partial y^i}(a)\frac{\partial}{\partial z^q},\quad T_a\beta'\bigg(\frac{\partial}{\partial u^i}\bigg)=\frac{\partial}{\partial u^i}+\sum_{q=1}^M\frac{\partial\iota^q}{\partial u^i}(a)\frac{\partial}{\partial z^q}. \end{align} $$
$$ \begin{align} T_a\beta'\bigg(\frac{\partial}{\partial y^i}\bigg)=\sum_{j=1}^n\frac{\partial f^j}{\partial y^i}(y)\frac{\partial}{\partial x^j}+\sum_{q=1}^M\frac{\partial\iota^q}{\partial y^i}(a)\frac{\partial}{\partial z^q},\quad T_a\beta'\bigg(\frac{\partial}{\partial u^i}\bigg)=\frac{\partial}{\partial u^i}+\sum_{q=1}^M\frac{\partial\iota^q}{\partial u^i}(a)\frac{\partial}{\partial z^q}. \end{align} $$
Therefore, we can choose
![]() $v_1,\ldots ,v_r\in T_b\mathbb B'$
in the span of
$v_1,\ldots ,v_r\in T_b\mathbb B'$
in the span of
![]() ${\partial }/{\partial x^j}$
and
${\partial }/{\partial x^j}$
and
![]() ${\partial }/{\partial z^q}$
so that the sequence
${\partial }/{\partial z^q}$
so that the sequence
 $$ \begin{align} \bigg(T_a\beta'\bigg(\frac{\partial}{\partial y^1}\bigg),\ldots,T_a\beta'\bigg(\frac{\partial}{\partial y^m}\bigg),T_a\beta'\bigg(\frac{\partial}{\partial u^1}\bigg),\ldots,T_a\beta'\bigg(\frac{\partial}{\partial u^{k'}}\bigg),v_1,\ldots,v_r\bigg) \end{align} $$
$$ \begin{align} \bigg(T_a\beta'\bigg(\frac{\partial}{\partial y^1}\bigg),\ldots,T_a\beta'\bigg(\frac{\partial}{\partial y^m}\bigg),T_a\beta'\bigg(\frac{\partial}{\partial u^1}\bigg),\ldots,T_a\beta'\bigg(\frac{\partial}{\partial u^{k'}}\bigg),v_1,\ldots,v_r\bigg) \end{align} $$
is an oriented basis of
![]() $T_b\mathbb B'$
. Note that our choice guarantees that
$T_b\mathbb B'$
. Note that our choice guarantees that
![]() $v_1,\ldots ,v_r\in T_a\mathbb B$
. By the convention of Section 2.10, the sequence
$v_1,\ldots ,v_r\in T_a\mathbb B$
. By the convention of Section 2.10, the sequence
![]() $(v_1+\mathop{\rm im} T_a\beta ',\ldots ,v_r+\mathop{\rm im} T_a\beta ')$
is an oriented basis of the fiber
$(v_1+\mathop{\rm im} T_a\beta ',\ldots ,v_r+\mathop{\rm im} T_a\beta ')$
is an oriented basis of the fiber
![]() $(\nu _{Y{}_L\! \times E^{N'},\mathbb B'})_b=T_b\mathbb B'/\mathop{\rm im} T_a\beta '$
. By (2-6), the transition matrix from (2-7) to the oriented basis
$(\nu _{Y{}_L\! \times E^{N'},\mathbb B'})_b=T_b\mathbb B'/\mathop{\rm im} T_a\beta '$
. By (2-6), the transition matrix from (2-7) to the oriented basis
of
![]() $T_b\mathbb B'$
has the form
$T_b\mathbb B'$
has the form
 $$ \begin{align*} \left( \begin{array}{ccc} F&0&V\\ 0&I_{k'}&0\\ Z_1&Z_2&Z_3 \end{array} \right) \end{align*} $$
$$ \begin{align*} \left( \begin{array}{ccc} F&0&V\\ 0&I_{k'}&0\\ Z_1&Z_2&Z_3 \end{array} \right) \end{align*} $$
for the matrices F, V,
![]() $Z_1$
,
$Z_1$
,
![]() $Z_2$
,
$Z_2$
,
![]() $Z_3$
of sizes
$Z_3$
of sizes
![]() $n\times m$
,
$n\times m$
,
![]() $n\times r$
,
$n\times r$
,
![]() $M\times n$
,
$M\times n$
,
![]() $M\times k'$
,
$M\times k'$
,
![]() $M\times r$
, respectively. By construction, the determinant of the above matrix is positive. Note that
$M\times r$
, respectively. By construction, the determinant of the above matrix is positive. Note that
![]() $k'-k=2(N'-N){\mathfrak n}_{G}$
is even. Therefore, if we cross out the rows and columns corresponding to coordinates
$k'-k=2(N'-N){\mathfrak n}_{G}$
is even. Therefore, if we cross out the rows and columns corresponding to coordinates
![]() $u^{k+1},\ldots ,u^{k'}$
, we get a matrix with positive determinant. Repeating the above arguments for the space
$u^{k+1},\ldots ,u^{k'}$
, we get a matrix with positive determinant. Repeating the above arguments for the space
![]() $\mathbb B$
(in reverse order), we get that
$\mathbb B$
(in reverse order), we get that
![]() $(v_1+\mathop{\rm im} T_a\beta ,\ldots ,v_r+\mathop{\rm im} T_a\beta )$
is an oriented basis of the fiber
$(v_1+\mathop{\rm im} T_a\beta ,\ldots ,v_r+\mathop{\rm im} T_a\beta )$
is an oriented basis of the fiber
![]() $(\nu _{Y{}_L\! \times E^N,\mathbb B})_b$
. This fact proves (2-3).
$(\nu _{Y{}_L\! \times E^N,\mathbb B})_b$
. This fact proves (2-3).
2.14 Semisimple groups
Let G be a semisimple compact Lie group. Then G can be described externally as the set of points fixed by some analytic automorphism of the corresponding complex Chevalley group
![]() $G^c$
[Reference SteinbergSt, Remark following Theorem 16].
$G^c$
[Reference SteinbergSt, Remark following Theorem 16].
We consider a maximal torus
![]() $K<G$
and the Weyl group
$K<G$
and the Weyl group
![]() $W=N_G(K)/K$
. For any
$W=N_G(K)/K$
. For any
![]() $w\in W$
, we arbitrarily choose its lifting
$w\in W$
, we arbitrarily choose its lifting
![]() $\dot w\in N_G(K)$
. We use the abbreviation
$\dot w\in N_G(K)$
. We use the abbreviation
![]() $wK=\dot {w}K$
.
$wK=\dot {w}K$
.
The group G has the Bruhat decomposition
![]() $G=\bigsqcup _{w\in W}K_w$
, where
$G=\bigsqcup _{w\in W}K_w$
, where
![]() $K_w=G\cap (BwB)$
and B is the Borel subgroup of
$K_w=G\cap (BwB)$
and B is the Borel subgroup of
![]() $G^c$
corresponding to a chosen Bruhat order
$G^c$
corresponding to a chosen Bruhat order
![]() $\leqslant $
on W [Reference SteinbergSt, Corollary 5 of Lemma 45]. Taking the quotient, we get the decomposition
$\leqslant $
on W [Reference SteinbergSt, Corollary 5 of Lemma 45]. Taking the quotient, we get the decomposition
![]() ${G/K=\bigsqcup _{w\in W}K_w/K}$
into Schubert cells. We can compute their closures, called Schubert varieties, as
${G/K=\bigsqcup _{w\in W}K_w/K}$
into Schubert cells. We can compute their closures, called Schubert varieties, as
![]() $\overline {K_w/K}=\bigsqcup _{w'\leqslant w}K_{w'}$
.
$\overline {K_w/K}=\bigsqcup _{w'\leqslant w}K_{w'}$
.
We have
![]() $K\cong (S^1)^d$
for some d, where
$K\cong (S^1)^d$
for some d, where
![]() $S^1$
is the unit circle. We get the map
$S^1$
is the unit circle. We get the map
![]() ${\rho _w:E\to E}$
given by
${\rho _w:E\to E}$
given by
![]() $\rho _w(e)=\dot we$
. This map factors through the action of K as
$\rho _w(e)=\dot we$
. This map factors through the action of K as
![]() ${\rho _w(ke)=k'\rho _w(e)}$
, where
${\rho _w(ke)=k'\rho _w(e)}$
, where
![]() $k'=\dot wk\dot w^{-1}\in K$
, and we get the map
$k'=\dot wk\dot w^{-1}\in K$
, and we get the map
![]() $K\mathop {\backslash }\rho _w:K\mathop {\backslash } E\to K\mathop {\backslash } E$
and hence its pull-back
$K\mathop {\backslash }\rho _w:K\mathop {\backslash } E\to K\mathop {\backslash } E$
and hence its pull-back
![]() $(K\mathop {\backslash }\rho _w)^*:H^{\bullet }_K(\mathrm {pt},\Bbbk )\to H^{\bullet }_K(\mathrm {pt},\Bbbk )$
. We think of this map as a left action of W on
$(K\mathop {\backslash }\rho _w)^*:H^{\bullet }_K(\mathrm {pt},\Bbbk )\to H^{\bullet }_K(\mathrm {pt},\Bbbk )$
. We think of this map as a left action of W on
![]() $H^{\bullet }_K(\mathrm {pt},\Bbbk )$
:
$H^{\bullet }_K(\mathrm {pt},\Bbbk )$
:
This formula agrees with the following standard representation of
![]() $H^{\bullet }_K(\mathrm {pt},\Bbbk )$
as a polynomial ring, refer to [Reference Brion, Broer, Daigneault and SabidussiBr, Section 1, Example 2] and [Reference JantzenJ, 1.7, 1.8]. Let us consider for each character
$H^{\bullet }_K(\mathrm {pt},\Bbbk )$
as a polynomial ring, refer to [Reference Brion, Broer, Daigneault and SabidussiBr, Section 1, Example 2] and [Reference JantzenJ, 1.7, 1.8]. Let us consider for each character
![]() $\lambda :K\to S^1$
the representation
$\lambda :K\to S^1$
the representation
![]() ${\mathbb C}_\lambda $
being the field of complex numbers
${\mathbb C}_\lambda $
being the field of complex numbers
![]() ${\mathbb C}$
as the vector space and having the following left K-action:
${\mathbb C}$
as the vector space and having the following left K-action:
![]() $kc=\lambda (k)c$
. We denote the line bundle
$kc=\lambda (k)c$
. We denote the line bundle
![]() $\mathop {\mathbf {can}}\nolimits :({\mathbb C}_\lambda ){}_K\! \times E\to K\mathop {\backslash } E$
by
$\mathop {\mathbf {can}}\nolimits :({\mathbb C}_\lambda ){}_K\! \times E\to K\mathop {\backslash } E$
by
![]() $\mathcal L(\lambda )$
. Its Euler class (that is, the first Chern class)
$\mathcal L(\lambda )$
. Its Euler class (that is, the first Chern class)
![]() $\mathop{\rm Eu}\nolimits (\mathcal L(\lambda ))$
is identified with
$\mathop{\rm Eu}\nolimits (\mathcal L(\lambda ))$
is identified with
![]() $\lambda $
itself. Thus, choosing
$\lambda $
itself. Thus, choosing
![]() $\varepsilon _1,\ldots ,\varepsilon _d$
freely generating the character group of K, we get that the cohomology ring
$\varepsilon _1,\ldots ,\varepsilon _d$
freely generating the character group of K, we get that the cohomology ring
![]() $H_K^{\bullet }(\mathrm {pt},\Bbbk )$
is a polynomial ring over
$H_K^{\bullet }(\mathrm {pt},\Bbbk )$
is a polynomial ring over
![]() $\Bbbk $
in variables
$\Bbbk $
in variables
![]() $\varepsilon _i$
.
$\varepsilon _i$
.
For any root
![]() $\alpha $
, we denote by
$\alpha $
, we denote by
![]() $G_\alpha $
the subgroup of G generated by the image of the root homomorphism
$G_\alpha $
the subgroup of G generated by the image of the root homomorphism
![]() $\varphi _\alpha :\mathop {\rm SU}\nolimits _2\to G$
and the torus K [Reference Bott and SamelsonBS, Ch. III (3.1)]. An alternative (external) description of this subgroup is given in [Reference SteinbergSt, Lemma 45]. As
$\varphi _\alpha :\mathop {\rm SU}\nolimits _2\to G$
and the torus K [Reference Bott and SamelsonBS, Ch. III (3.1)]. An alternative (external) description of this subgroup is given in [Reference SteinbergSt, Lemma 45]. As
![]() $G_\alpha =G_{-\alpha }$
, we can denote
$G_\alpha =G_{-\alpha }$
, we can denote
![]() $G_{\omega _\alpha }=G_\alpha $
, where
$G_{\omega _\alpha }=G_\alpha $
, where
![]() $\omega _\alpha \in W$
is the reflection through the plane perpendicular to
$\omega _\alpha \in W$
is the reflection through the plane perpendicular to
![]() $\alpha $
. We call elements
$\alpha $
. We call elements
![]() $\omega _\alpha $
reflections. In what follows, for any reflection t, we denote by
$\omega _\alpha $
reflections. In what follows, for any reflection t, we denote by
![]() $\alpha _t$
the positive root such that
$\alpha _t$
the positive root such that
![]() $t=\omega _{\alpha _t}$
. We also reserve the letter s (with indices or accents, primed or underlined) to denote simple reflections or sequences of simple reflections. This notation is used only in Sections 6.4–6.9.
$t=\omega _{\alpha _t}$
. We also reserve the letter s (with indices or accents, primed or underlined) to denote simple reflections or sequences of simple reflections. This notation is used only in Sections 6.4–6.9.
3 The tensor product morphism
3.1 Twisted action
Let G be a topological group, and L and R be subgroups. We assume that L and R act on G on the left and on the right, respectively, by multiplication. As both actions commute, the group L acts continuously on the quotient space
![]() $G/R$
on the left.
$G/R$
on the left.
Let X be another left L-space and
![]() $A:X\to G/R$
be an L-equivariant continuous map. We call A a twisting map. For any left G-space E, the map A allows us to define the map
$A:X\to G/R$
be an L-equivariant continuous map. We call A a twisting map. For any left G-space E, the map A allows us to define the map
by
Note that
![]() $A(x)^{-1}\in R\mathop {\backslash } G$
. The reader can easily check that
$A(x)^{-1}\in R\mathop {\backslash } G$
. The reader can easily check that
![]() $v_A$
is well defined and continuous. We call this map the projection twisted by A.
$v_A$
is well defined and continuous. We call this map the projection twisted by A.
Let us suppose that the quotient maps
![]() $E\to L\mathop {\backslash } E$
and
$E\to L\mathop {\backslash } E$
and
![]() $E\to R\mathop {\backslash } E$
are universal principal L- and R-bundles, respectively. Then we get the map between cohomologies
$E\to R\mathop {\backslash } E$
are universal principal L- and R-bundles, respectively. Then we get the map between cohomologies
![]() $v_A^*:H^{\bullet }_R(\mathrm {pt},\Bbbk )\to H^{\bullet }_L(X,\Bbbk )$
, which induces the following right
$v_A^*:H^{\bullet }_R(\mathrm {pt},\Bbbk )\to H^{\bullet }_L(X,\Bbbk )$
, which induces the following right
![]() $H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-action on
$H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-action on
![]() $H^{\bullet }_L(X,\Bbbk )$
:
$H^{\bullet }_L(X,\Bbbk )$
:
We call this action the action twisted by A.
A priori, this construction of a right module depends on the choice of E. However, we get the following result.
Lemma 3.1. Up to isomorphism, the right
![]() $H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module
$H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module
![]() $H^{\bullet }_L(X,\Bbbk )$
is independent of the choice of E.
$H^{\bullet }_L(X,\Bbbk )$
is independent of the choice of E.
Proof. Let
![]() $\widetilde E$
be another left G-space such that
$\widetilde E$
be another left G-space such that
![]() $\widetilde E\to L\mathop {\backslash }\widetilde E$
and
$\widetilde E\to L\mathop {\backslash }\widetilde E$
and
![]() $\tilde E\to R\mathop {\backslash }\tilde E$
are universal principal L- and R-bundles, respectively. We use the following notation only in this proof:
$\tilde E\to R\mathop {\backslash }\tilde E$
are universal principal L- and R-bundles, respectively. We use the following notation only in this proof:
We also have the map
![]() $\tilde v_A:X{}_L\!\times \widetilde E\to R\mathop {\backslash }\widetilde E$
similar to
$\tilde v_A:X{}_L\!\times \widetilde E\to R\mathop {\backslash }\widetilde E$
similar to
![]() $v_A$
also given by (3-1). This map defines the structure of a right
$v_A$
also given by (3-1). This map defines the structure of a right
![]() $\widetilde H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module on
$\widetilde H^{\bullet }_R(\mathrm {pt},\Bbbk )$
-module on
![]() $\widetilde H^{\bullet }_L(X,\Bbbk )$
by
$\widetilde H^{\bullet }_L(X,\Bbbk )$
by
Let us make L and R act on the products
![]() $X\times E\times \widetilde E$
and
$X\times E\times \widetilde E$
and
![]() $E\times \widetilde E$
, respectively, diagonally:
$E\times \widetilde E$
, respectively, diagonally:
![]() $l(x,e,\tilde e)=(lx,le,l\tilde e)$
and
$l(x,e,\tilde e)=(lx,le,l\tilde e)$
and
![]() $r(e,\tilde e)=(re,r\tilde e)$
. Let
$r(e,\tilde e)=(re,r\tilde e)$
. Let
![]() $q_A$
be the map given by
$q_A$
be the map given by
![]() $L(x,e,\tilde e)\mapsto A(x)^{-1}(e,\tilde e)$
. Then we get the following diagram:
$L(x,e,\tilde e)\mapsto A(x)^{-1}(e,\tilde e)$
. Then we get the following diagram:

If we forget about the dashed arrows, then we get a commutative diagram (as the underlying diagram for sets is commutative). Therefore, the central rectangle (with the dashed arrows) is also commutative, because all slant arrows are isomorphisms. As all maps preserve the additive structure on cohomologies, it remains to check Condition (2-1): for
![]() $m\in H_L^{\bullet }(X,\Bbbk )$
and
$m\in H_L^{\bullet }(X,\Bbbk )$
and
![]() $b\in H_R^{\bullet }(\mathrm {pt},\Bbbk )$
,
$b\in H_R^{\bullet }(\mathrm {pt},\Bbbk )$
,
where we use the multiplication rules (3-2) and (3-3), and the fact that
![]() $\mu $
preserves the cup product.
$\mu $
preserves the cup product.
Corollary 3.2 (of Lemma 3.1).
Up to isomorphism, the
![]() $H_L^{\bullet }(\mathrm {pt},\Bbbk )$
-
$H_L^{\bullet }(\mathrm {pt},\Bbbk )$
-
![]() $H^{\bullet }_R(\mathrm {pt},\Bbbk )$
- bimodule
$H^{\bullet }_R(\mathrm {pt},\Bbbk )$
- bimodule
![]() $H^{\bullet }_L(X,\Bbbk )$
is independent of the choice of E.
$H^{\bullet }_L(X,\Bbbk )$
is independent of the choice of E.
Proof. It suffices to prove a result similar to Lemma 3.1 for the canonical action. We can do it if in (3-4), we replace: R with L;
![]() $v_A$
and
$v_A$
and
![]() $\tilde v_A$
with the canonical projections
$\tilde v_A$
with the canonical projections
![]() $X\,{}_L\!\times \,E\to L\mathop {\backslash } E$
and
$X\,{}_L\!\times \,E\to L\mathop {\backslash } E$
and
![]() $X\,{}_L\!\times \,\tilde E\to L\mathop {\backslash } \tilde E$
, respectively;
$X\,{}_L\!\times \,\tilde E\to L\mathop {\backslash } \tilde E$
, respectively;
![]() $q_A$
with
$q_A$
with
![]() $L\mathop {\backslash }\mathop{\rm pr}\nolimits _{2,3}$
.
$L\mathop {\backslash }\mathop{\rm pr}\nolimits _{2,3}$
.
Remark 3.3. The above stipulation that both
![]() $E\to L\mathop {\backslash } E$
and
$E\to L\mathop {\backslash } E$
and
![]() $E\to R\mathop {\backslash } E$
are universal principal L- and R-bundles usually is the result of the fact that G is a Lie group, L and R are its closed subgroups, and the quotient map
$E\to R\mathop {\backslash } E$
are universal principal L- and R-bundles usually is the result of the fact that G is a Lie group, L and R are its closed subgroups, and the quotient map
![]() $E\to G\mathop {\backslash } E$
is a universal principal G-bundle.
$E\to G\mathop {\backslash } E$
is a universal principal G-bundle.
Remark 3.4. If
![]() $R=L$
and
$R=L$
and
![]() $A:X\to G/L$
is defined by
$A:X\to G/L$
is defined by
![]() $A(x)=L$
for any
$A(x)=L$
for any
![]() $x\in X$
, then
$x\in X$
, then
![]() $v_A$
is the canonical projection. In this case, the corresponding right action (3-2) of
$v_A$
is the canonical projection. In this case, the corresponding right action (3-2) of
![]() $H^{\bullet }_L(\mathrm {pt},\Bbbk )$
on
$H^{\bullet }_L(\mathrm {pt},\Bbbk )$
on
![]() $H_L^{\bullet }(X,\Bbbk )$
is canonical.
$H_L^{\bullet }(X,\Bbbk )$
is canonical.
3.2 Pull-back as a bimodule homomorphism
Keeping the notation of the previous section, let additionally Y be another L-space and
![]() $f:Y\to X$
be an L-equivariant continuous map. Then the composition
$f:Y\to X$
be an L-equivariant continuous map. Then the composition
![]() $Af:Y\to G/R$
is also L-equivariant.
$Af:Y\to G/R$
is also L-equivariant.
Lemma 3.5. The equivariant pull-back
![]() $f^{\star }:H^{\bullet }_L(X,\Bbbk )\to H^{\bullet }_L(Y,\Bbbk )$
is a homomorphism of bimodules, where the left actions are canonical and the right actions on the domain and codomain are twisted by A and
$f^{\star }:H^{\bullet }_L(X,\Bbbk )\to H^{\bullet }_L(Y,\Bbbk )$
is a homomorphism of bimodules, where the left actions are canonical and the right actions on the domain and codomain are twisted by A and
![]() $Af$
, respectively.
$Af$
, respectively.
Proof. First, we prove the claim about the left actions. We get the following commutative diagram:

Let
![]() $a\in H_L^{\bullet }(\mathrm {pt},\Bbbk )$
and
$a\in H_L^{\bullet }(\mathrm {pt},\Bbbk )$
and
![]() $m\in H^{\bullet }_L(X,\Bbbk )$
. It follows from the above diagram that
$m\in H^{\bullet }_L(X,\Bbbk )$
. It follows from the above diagram that
Now let us deal with the right actions. It follows directly from definition that
Hence, for any
![]() $m\in H^{\bullet }_L(X,\Bbbk )$
and
$m\in H^{\bullet }_L(X,\Bbbk )$
and
![]() $b\in H_R^{\bullet }(\mathrm {pt},\Bbbk )$
,
$b\in H_R^{\bullet }(\mathrm {pt},\Bbbk )$
,
3.3 Push-forward as a bimodule homomorphism
Suppose we are in the situation of Section 2.13, so
![]() $f\;{}_L\! \times \mathrm {id}:Y\;{}_L\! \times E^N\to X\;{}_L\! \times E^N$
is denoted by
$f\;{}_L\! \times \mathrm {id}:Y\;{}_L\! \times E^N\to X\;{}_L\! \times E^N$
is denoted by
![]() $f^N$
.
$f^N$
.
Let R be a closed subgroup of G and
![]() $A:X\to G/R$
be an L-equivariant continuous map (as in Section 3.1). We get the commutative diagrams (refer to Remark 2.8 about the notation)
$A:X\to G/R$
be an L-equivariant continuous map (as in Section 3.1). We get the commutative diagrams (refer to Remark 2.8 about the notation)

Let
![]() $m\in H_L^n(Y,\Bbbk )$
and
$m\in H_L^n(Y,\Bbbk )$
and
![]() $b\in H_R^l(\mathrm {pt},\Bbbk )$
. For N big enough, we get by (2-5), the commutativity of diagrams (3-7), the projection formula (Proposition 2.9), and a compact version of (3-6) that
$b\in H_R^l(\mathrm {pt},\Bbbk )$
. For N big enough, we get by (2-5), the commutativity of diagrams (3-7), the projection formula (Proposition 2.9), and a compact version of (3-6) that
 $$ \begin{align*} f_\star(mb)&=f_\star(m\cup v_{Af}^*(b))=(i_X^*)^{-1}f_*^Ni_Y^*(m\cup v_{Af}^*(b))\\ &=(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup(v_{Af}i_Y)^*(b)) =(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup(v^N_{Af})^*i_R^*(b))\\ &=(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup (f^N)^*(v^N_A)^*i_R^*(b)) =(i_X^*)^{-1}(f_*^Ni_Y^*(m)\cup(i_Rv^N_A)^*(b))\\ &=(i_X^*)^{-1}(f_*^Ni_Y^*(m)\cup i_X^*v_A^*(b)) =(i_X^*)^{-1}f_*^Ni_Y^*(m)\cup v_A^*(b)=f_\star(m)b. \end{align*} $$
$$ \begin{align*} f_\star(mb)&=f_\star(m\cup v_{Af}^*(b))=(i_X^*)^{-1}f_*^Ni_Y^*(m\cup v_{Af}^*(b))\\ &=(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup(v_{Af}i_Y)^*(b)) =(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup(v^N_{Af})^*i_R^*(b))\\ &=(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup (f^N)^*(v^N_A)^*i_R^*(b)) =(i_X^*)^{-1}(f_*^Ni_Y^*(m)\cup(i_Rv^N_A)^*(b))\\ &=(i_X^*)^{-1}(f_*^Ni_Y^*(m)\cup i_X^*v_A^*(b)) =(i_X^*)^{-1}f_*^Ni_Y^*(m)\cup v_A^*(b)=f_\star(m)b. \end{align*} $$
We also get the commutative diagrams

Suppose that the cohomology
![]() $H_L^{\bullet }(\mathrm {pt},\Bbbk )$
vanishes in odd degrees. Let
$H_L^{\bullet }(\mathrm {pt},\Bbbk )$
vanishes in odd degrees. Let
![]() $a\in H_R^{2l}(\mathrm {pt},\Bbbk )$
and
$a\in H_R^{2l}(\mathrm {pt},\Bbbk )$
and
![]() $m\in H_L^n(Y,\Bbbk )$
. For N big enough, we get by (2-5), the commutativity of diagrams (3-8), the projection formula (Proposition 2.9), the graded-commutativity of the cup product, and a compact version of (3-5) that
$m\in H_L^n(Y,\Bbbk )$
. For N big enough, we get by (2-5), the commutativity of diagrams (3-8), the projection formula (Proposition 2.9), the graded-commutativity of the cup product, and a compact version of (3-5) that
 $$ \begin{align*} f_\star(am)&=f_\star(\mathop{\mathbf{can}}\nolimits^*(a)\cup m)=(i_X^*)^{-1}f_*^Ni_Y^*(\mathop{\mathbf{can}}\nolimits^*(a)\cup m)\\ &=(i_X^*)^{-1}f_*^N((\mathop{\mathbf{can}}\nolimits i_Y)^*(a)\cup i_Y^*(m))\\ &=(i_X^*)^{-1}f_*^N(\mathop{\mathbf{can}}\nolimits^* i_L^*(a)\cup i_Y^*(m))=(i_X^*)^{-1}f_*^N((f^N)^*\mathop{\mathbf{can}}\nolimits^*i_L^*(a)\cup i_Y^*(m))\\ &=(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup(f^N)^*(i_L\mathop{\mathbf{can}}\nolimits)^*(a))=(i_X^*)^{-1}(f_*^Ni_Y^*(m)\cup(\mathop{\mathbf{can}}\nolimits i_X)^*(a))\\ &=(i_X^*)^{-1}f_*^Ni_Y^*(m)\cup\mathop{\mathbf{can}}\nolimits^*(a)=\mathop{\mathbf{can}}\nolimits^*(a)\cup f_\star(m)=af_\star(m). \end{align*} $$
$$ \begin{align*} f_\star(am)&=f_\star(\mathop{\mathbf{can}}\nolimits^*(a)\cup m)=(i_X^*)^{-1}f_*^Ni_Y^*(\mathop{\mathbf{can}}\nolimits^*(a)\cup m)\\ &=(i_X^*)^{-1}f_*^N((\mathop{\mathbf{can}}\nolimits i_Y)^*(a)\cup i_Y^*(m))\\ &=(i_X^*)^{-1}f_*^N(\mathop{\mathbf{can}}\nolimits^* i_L^*(a)\cup i_Y^*(m))=(i_X^*)^{-1}f_*^N((f^N)^*\mathop{\mathbf{can}}\nolimits^*i_L^*(a)\cup i_Y^*(m))\\ &=(i_X^*)^{-1}f_*^N(i_Y^*(m)\cup(f^N)^*(i_L\mathop{\mathbf{can}}\nolimits)^*(a))=(i_X^*)^{-1}(f_*^Ni_Y^*(m)\cup(\mathop{\mathbf{can}}\nolimits i_X)^*(a))\\ &=(i_X^*)^{-1}f_*^Ni_Y^*(m)\cup\mathop{\mathbf{can}}\nolimits^*(a)=\mathop{\mathbf{can}}\nolimits^*(a)\cup f_\star(m)=af_\star(m). \end{align*} $$
So we have proved the following counterpart of Lemma 3.5.
Lemma 3.6. The equivariant push-forward
![]() $f_\star :H^{\bullet }_L(Y,\Bbbk )\to H^{\bullet +\dim X-\dim Y}_L(X,\Bbbk )$
is a homomorphism of right
$f_\star :H^{\bullet }_L(Y,\Bbbk )\to H^{\bullet +\dim X-\dim Y}_L(X,\Bbbk )$
is a homomorphism of right
![]() $H_R^{\bullet }(\mathrm {pt},\Bbbk )$
-modules, where the right actions on the domain and codomain are twisted by
$H_R^{\bullet }(\mathrm {pt},\Bbbk )$
-modules, where the right actions on the domain and codomain are twisted by
![]() $Af$
and A, respectively.
$Af$
and A, respectively.
If, moreover, the cohomology
![]() $H_L^{\bullet }(\mathrm {pt},\Bbbk )$
vanishes in odd degrees, then
$H_L^{\bullet }(\mathrm {pt},\Bbbk )$
vanishes in odd degrees, then
![]() $f_\star $
is a homomorphism of left
$f_\star $
is a homomorphism of left
![]() $H_L^{\bullet }(\mathrm {pt},\Bbbk )$
-modules, where both left actions of
$H_L^{\bullet }(\mathrm {pt},\Bbbk )$
-modules, where both left actions of
![]() $H_L^{\bullet }(\mathrm {pt},\Bbbk )$
are canonical.
$H_L^{\bullet }(\mathrm {pt},\Bbbk )$
are canonical.
3.4 The embedding of Borel constructions
We fix the setup that we preserve until the end of Section 3. Let
![]() $L,R,P,Q$
be subgroups of a topological group G such that
$L,R,P,Q$
be subgroups of a topological group G such that
![]() $R\subset P$
and
$R\subset P$
and
![]() $Q\subset P$
. Let X and Y be topological spaces such that:
$Q\subset P$
. Let X and Y be topological spaces such that:
-
(i) L acts on the left on X;
-
(ii) R acts on the right on X;
-
(iii) Q acts on the left on Y.
Assuming that R and Q act on P on the left and on the right, respectively, via multiplication, we can write these data as follows:
We also assume that:
-
(iv) the actions of L and R on X commute.
Let additionally:
-
(v)
 $\alpha :X\to G$
be a continuous R- and L-equivariant map.
$\alpha :X\to G$
be a continuous R- and L-equivariant map.
In that case, we get the morphism of left L-spaces
![]() $A:X/R\to G/R$
given by
$A:X/R\to G/R$
given by
Let E be a left G-space. Then we are in the situation of Section 3.1 (with X replaced by
![]() $X/R$
) and we have the map
$X/R$
) and we have the map
![]() $v_A:(X/R)\,{}_L\!\times \,E\to R\mathop {\backslash } E$
given by (3-1).
$v_A:(X/R)\,{}_L\!\times \,E\to R\mathop {\backslash } E$
given by (3-1).
In view of item (iv), the group L acts on the left on
![]() $X\mathop {\times }\limits _R P\mathop {\times }\limits _QY$
by
$X\mathop {\times }\limits _R P\mathop {\times }\limits _QY$
by
![]() $ l[x:p:y]=[lx:p:y] $
. We consider the map
$ l[x:p:y]=[lx:p:y] $
. We consider the map
defined by
The reader can easily check that this map is well defined and continuous.
We get the following diagram:

where
![]() $\pi _R$
and
$\pi _R$
and
![]() $\pi _Q$
are the natural quotient maps. In general, the rectangle on the right is not commutative. However, we get the following result.
$\pi _Q$
are the natural quotient maps. In general, the rectangle on the right is not commutative. However, we get the following result.
Lemma 3.7. The image of
![]() $\varphi $
is equal to
$\varphi $
is equal to
![]() $((X/R)\,{}_L\!\times \,E)\times _{\pi _R\,v_A\mathop{\rm pr}\nolimits _1=\pi _Q\mathop {\mathbf {can}}\nolimits \mathop{\rm pr}\nolimits _2}(Y\,{}_Q\!\times \,E)$
.
$((X/R)\,{}_L\!\times \,E)\times _{\pi _R\,v_A\mathop{\rm pr}\nolimits _1=\pi _Q\mathop {\mathbf {can}}\nolimits \mathop{\rm pr}\nolimits _2}(Y\,{}_Q\!\times \,E)$
.
Proof. It follows from (3-10) that the two maximal paths of diagram (3-11) commute, that is,
![]() $\pi _R\,v_A\mathop{\rm pr}\nolimits _1\varphi =\pi _Q \mathop {\mathbf {can}}\nolimits \mathop{\rm pr}\nolimits _2\varphi $
. Thus, the image of
$\pi _R\,v_A\mathop{\rm pr}\nolimits _1\varphi =\pi _Q \mathop {\mathbf {can}}\nolimits \mathop{\rm pr}\nolimits _2\varphi $
. Thus, the image of
![]() $\varphi $
is contained in the fiber product.
$\varphi $
is contained in the fiber product.
Conversely, let
![]() $a=(L(xR,e),Q(y,e'))$
be an arbitrary point of the fiber product. Hence,
$a=(L(xR,e),Q(y,e'))$
be an arbitrary point of the fiber product. Hence,
![]() $P\alpha (x)^{-1}e=Pe'$
. Thus, there exists an element
$P\alpha (x)^{-1}e=Pe'$
. Thus, there exists an element
![]() $p\in P$
such that
$p\in P$
such that
![]() $pe'=\alpha (x)^{-1}e$
. We get
$pe'=\alpha (x)^{-1}e$
. We get
![]() $\varphi (L([x:p:y],e))=a$
.
$\varphi (L([x:p:y],e))=a$
.
Lemma 3.8. Suppose that P acts freely on E. Then
![]() $\varphi $
is an embedding.
$\varphi $
is an embedding.
Proof. Let
![]() $L([x:p:y],e)$
and
$L([x:p:y],e)$
and
![]() $L([x':p':y'],e')$
be two orbits mapped to the same pair by
$L([x':p':y'],e')$
be two orbits mapped to the same pair by
![]() $\varphi $
. As
$\varphi $
. As
![]() $L(xR,e)=L(x'R,e')$
, there exists
$L(xR,e)=L(x'R,e')$
, there exists
![]() $l\in L$
such that
$l\in L$
such that
![]() $lxR=x'R$
and
$lxR=x'R$
and
![]() $le=e'$
. From the first equality, it follows that there exists
$le=e'$
. From the first equality, it follows that there exists
![]() $r\in R$
such that
$r\in R$
such that
![]() $lxr=x'$
. We get
$lxr=x'$
. We get
 $$ \begin{align}L([x:p:y],e)&=L([lx:p:y],le)=L([lxr:r^{-1}p:y],e') \nonumber\\ &=L([x':r^{-1}p:y],e')\mathop{\longmapsto}\limits^{\ \ {\rm pr}_2\varphi\ \ }Q(y,p^{-1}r\alpha(x')^{-1}e'). \end{align} $$
$$ \begin{align}L([x:p:y],e)&=L([lx:p:y],le)=L([lxr:r^{-1}p:y],e') \nonumber\\ &=L([x':r^{-1}p:y],e')\mathop{\longmapsto}\limits^{\ \ {\rm pr}_2\varphi\ \ }Q(y,p^{-1}r\alpha(x')^{-1}e'). \end{align} $$
As
![]() $L([x':p':y'],e')$
is mapped by
$L([x':p':y'],e')$
is mapped by
![]() $\mathop{\rm pr}\nolimits _2\varphi $
to the same orbit,
$\mathop{\rm pr}\nolimits _2\varphi $
to the same orbit,
Therefore, there exists
![]() $q\in Q$
such that
$q\in Q$
such that
![]() $qy=y'$
and
$qy=y'$
and
![]() $qp^{-1}r\alpha (x')^{-1}e'=(p')^{-1}\alpha (x')^{-1}e'$
. From the last equality and the freeness of the action of P on E, we get
$qp^{-1}r\alpha (x')^{-1}e'=(p')^{-1}\alpha (x')^{-1}e'$
. From the last equality and the freeness of the action of P on E, we get
![]() $qp^{-1}r=(p')^{-1}$
. Hence,
$qp^{-1}r=(p')^{-1}$
. Hence,
![]() $r^{-1}p=p'q$
and, applying (3-12),
$r^{-1}p=p'q$
and, applying (3-12),
 $$ \begin{align*} L([x:p:y],e)&=L([x':r^{-1}p:y],e')=L([x':p'q:y],e')\\ &=L([x':p':qy],e')=L([x':p':y'],e'), \end{align*} $$
$$ \begin{align*} L([x:p:y],e)&=L([x':r^{-1}p:y],e')=L([x':p'q:y],e')\\ &=L([x':p':qy],e')=L([x':p':y'],e'), \end{align*} $$
as required.
Theorem 3.9. Suppose that the quotient map
![]() $E\to P\mathop {\backslash } E$
is a principal P-bundle. Then
$E\to P\mathop {\backslash } E$
is a principal P-bundle. Then
![]() $\varphi $
is a topological embedding.
$\varphi $
is a topological embedding.
Proof. We denote this quotient map by
![]() $\pi $
and by D the fiber product from the formulation of Lemma 3.7. Let a be any point of D. The assumption of this lemma implies that there exists an open neighborhood
$\pi $
and by D the fiber product from the formulation of Lemma 3.7. Let a be any point of D. The assumption of this lemma implies that there exists an open neighborhood
![]() $U\subset P\mathop {\backslash } E$
of the point
$U\subset P\mathop {\backslash } E$
of the point
![]() $\pi _Rv_A\mathop{\rm pr}\nolimits _1(a)=\pi _Q\mathop {\mathbf {can}}\nolimits \mathop{\rm pr}\nolimits _2(a)$
and a P-equivariant homeomorphism
$\pi _Rv_A\mathop{\rm pr}\nolimits _1(a)=\pi _Q\mathop {\mathbf {can}}\nolimits \mathop{\rm pr}\nolimits _2(a)$
and a P-equivariant homeomorphism
![]() $h:P \times U\stackrel \sim \to \pi ^{-1}(U)$
such that the diagram
$h:P \times U\stackrel \sim \to \pi ^{-1}(U)$
such that the diagram

is commutative. We set
![]() $\mathbf p=\mathop{\rm pr}\nolimits _1 h^{-1}$
. Clearly,
$\mathbf p=\mathop{\rm pr}\nolimits _1 h^{-1}$
. Clearly,
![]() $\mathbf p(pe)=p\mathbf p(e)$
for any
$\mathbf p(pe)=p\mathbf p(e)$
for any
![]() $p\in P$
and
$p\in P$
and
![]() ${e\in \pi ^{-1}(U)}$
.
${e\in \pi ^{-1}(U)}$
.
We define the continuous function
as follows. Let
![]() $d=(L(xR,e),Q(y,e'))$
be a point of the domain of
$d=(L(xR,e),Q(y,e'))$
be a point of the domain of
![]() $\xi $
. We set
$\xi $
. We set
One can easily check that this definition does not depend on the choice of
![]() $x,y,e,e'$
in the representation of d.
$x,y,e,e'$
in the representation of d.
Now suppose that
![]() $d\in D$
. Then we get
$d\in D$
. Then we get
![]() $P\alpha (x)^{-1}e=Pe'$
. Let us write
$P\alpha (x)^{-1}e=Pe'$
. Let us write
![]() $\alpha (x)^{-1}e=pe'$
for the corresponding
$\alpha (x)^{-1}e=pe'$
for the corresponding
![]() $p\in P$
. We get
$p\in P$
. We get
 $$ \begin{align*} \varphi\xi(d)&=(L(xR,e),Q(y ,\mathbf p(e')\mathbf p(\alpha(x)^{-1}e)^{-1}\alpha(x)^{-1}e))\\ &=(L(xR,e),Q(y ,\mathbf p(e')\mathbf p(pe')^{-1}pe'))\\ &=(L(xR,e),Q(y ,\mathbf p(e')\mathbf p(e')^{-1}p^{-1}pe')) =(L(xR,e),Q(y ,e'))=d. \end{align*} $$
$$ \begin{align*} \varphi\xi(d)&=(L(xR,e),Q(y ,\mathbf p(e')\mathbf p(\alpha(x)^{-1}e)^{-1}\alpha(x)^{-1}e))\\ &=(L(xR,e),Q(y ,\mathbf p(e')\mathbf p(pe')^{-1}pe'))\\ &=(L(xR,e),Q(y ,\mathbf p(e')\mathbf p(e')^{-1}p^{-1}pe')) =(L(xR,e),Q(y ,e'))=d. \end{align*} $$
This calculation proves that the restriction of
![]() $\xi $
to
$\xi $
to
![]() $(\pi _Rv_A)^{-1}(U)\times (\pi _Q\mathop {\mathbf {can}}\nolimits )^{-1}(U)\cap D$
inverts
$(\pi _Rv_A)^{-1}(U)\times (\pi _Q\mathop {\mathbf {can}}\nolimits )^{-1}(U)\cap D$
inverts
![]() $\varphi $
. As a belongs to the last subset, all such subsets cover D. Thus, the required claim follows from Lemma 3.8 and Proposition 2.1.
$\varphi $
. As a belongs to the last subset, all such subsets cover D. Thus, the required claim follows from Lemma 3.8 and Proposition 2.1.
3.5 Canonical and twisted projections for the triple product
By (3-10), we get the following commutative diagram:

Now let M be a subgroup of G and
![]() $B:Y\to G/M$
be a Q-equivariant continuous map. We consider the map
$B:Y\to G/M$
be a Q-equivariant continuous map. We consider the map
![]() $\alpha B:X\mathop {\times }\limits _R P\mathop {\times }\limits _Q Y\to G/M$
given by
$\alpha B:X\mathop {\times }\limits _R P\mathop {\times }\limits _Q Y\to G/M$
given by
It is easy to check that this map is well defined, continuous, and L-equivariant. By (3-10), we get the following commutative diagram:

3.6 The difference
In this section, we want to study the difference
To this end, we claim some additional properties of E.
Lemma 3.10. Suppose that P acts freely on E. Let U be a compact Lie group acting continuously on E on the right so that this action and the left action of G commute. Suppose that the space
![]() $R\mathop {\backslash } E$
is Hausdorff and is acted upon by U transitively. Then the projection to the first component
$R\mathop {\backslash } E$
is Hausdorff and is acted upon by U transitively. Then the projection to the first component
is a fiber bundle with fiber homeomorphic to
where
![]() $\bar e$
is an arbitrary point of
$\bar e$
is an arbitrary point of
![]() $P\mathop {\backslash } E$
.
$P\mathop {\backslash } E$
.
Proof. We denote the action of U by
![]() $\cdot $
. We make U act on
$\cdot $
. We make U act on
![]() $Y\,{}_Q\!\times \,E$
on the right by the rule
$Y\,{}_Q\!\times \,E$
on the right by the rule
![]() $Q(y,e)\cdot u=Q(y,e\cdot u)$
. Then we obviously get
$Q(y,e)\cdot u=Q(y,e\cdot u)$
. Then we obviously get
![]() $F_{\bar e}\cdot u=F_{\bar e\cdot u}$
. As U acts transitively on
$F_{\bar e}\cdot u=F_{\bar e\cdot u}$
. As U acts transitively on
![]() $R\mathop {\backslash } E$
, it also acts transitively on
$R\mathop {\backslash } E$
, it also acts transitively on
![]() $P\mathop {\backslash } E$
. Therefore, the spaces
$P\mathop {\backslash } E$
. Therefore, the spaces
![]() $F_{\bar e}$
are homeomorphic for different points
$F_{\bar e}$
are homeomorphic for different points
![]() $\bar e$
. We rely on the notation of Diagram (3-11).
$\bar e$
. We rely on the notation of Diagram (3-11).
Let
![]() $a\in (X/R)\,{}_L\!\times \,E$
be an arbitrary point. By Lemma 3.8,
$a\in (X/R)\,{}_L\!\times \,E$
be an arbitrary point. By Lemma 3.8,
We consider the map
![]() $t:U\to R\mathop {\backslash } E$
defined by
$t:U\to R\mathop {\backslash } E$
defined by
![]() $t(u)=v_A(a)u$
. Let V be the stabilizer of
$t(u)=v_A(a)u$
. Let V be the stabilizer of
![]() $v_A(a)$
in U. Note that V is a Lie group as it is closed in U. Let us consider the commutative diagram
$v_A(a)$
in U. Note that V is a Lie group as it is closed in U. Let us consider the commutative diagram

The bottom map, which is given by
![]() $Vu\mapsto v_A(a)\cdot u$
, is continuous and bijective. It is a homeomorphism, being a map from a compact space to a Hausdorff space. As the natural projection
$Vu\mapsto v_A(a)\cdot u$
, is continuous and bijective. It is a homeomorphism, being a map from a compact space to a Hausdorff space. As the natural projection
![]() $U\to V\mathop {\backslash } U$
is a principal V-bundle, it has a continuous section in an open neighborhood of any point of
$U\to V\mathop {\backslash } U$
is a principal V-bundle, it has a continuous section in an open neighborhood of any point of
![]() $V\mathop {\backslash } U$
. From the diagram above, we get the same property for the map t. In particular, there exist an open neighborhood W of
$V\mathop {\backslash } U$
. From the diagram above, we get the same property for the map t. In particular, there exist an open neighborhood W of
![]() $v_A(a)$
in
$v_A(a)$
in
![]() $R\mathop {\backslash } E$
and a continuous section
$R\mathop {\backslash } E$
and a continuous section
![]() $s:W\to U$
of t. In other words,
$s:W\to U$
of t. In other words,
for any
![]() $w\in W$
.
$w\in W$
.
Let
![]() $H=v_A^{-1}(W)$
. It is an open subset of
$H=v_A^{-1}(W)$
. It is an open subset of
![]() $(X/R)\,{}_L\!\times \,E$
containing a. We construct the map
$(X/R)\,{}_L\!\times \,E$
containing a. We construct the map
![]() $\sigma :H\times \omega ^{-1}(a)\to ((X/R)\,{}_L\!\times \,E)\times (Y\,{}_Q\!\times \,E)$
by
$\sigma :H\times \omega ^{-1}(a)\to ((X/R)\,{}_L\!\times \,E)\times (Y\,{}_Q\!\times \,E)$
by
This map is obviously well defined and continuous. Let us prove that
![]() $\mathop{\rm im}\sigma \cap \mathop{\rm im}\varphi =\varnothing $
. By Lemma 3.8, we have to prove the inequality
$\mathop{\rm im}\sigma \cap \mathop{\rm im}\varphi =\varnothing $
. By Lemma 3.8, we have to prove the inequality
As the G- and U-actions on E commute, the map
![]() $\pi _R$
is U-equivariant. Therefore, (3-17) is equivalent to
$\pi _R$
is U-equivariant. Therefore, (3-17) is equivalent to
Applying (3-16) for
![]() $w=v_A(h)$
, to compute the left-hand side, we get the equivalent inequality
$w=v_A(h)$
, to compute the left-hand side, we get the equivalent inequality
which holds by Lemma 3.8, as
![]() $(a,Q(y,e))\notin \mathop{\rm im}\varphi $
. Thus, we can consider
$(a,Q(y,e))\notin \mathop{\rm im}\varphi $
. Thus, we can consider
![]() $\sigma $
as a continuous map from
$\sigma $
as a continuous map from
![]() $H\times \omega ^{-1}(a)$
to
$H\times \omega ^{-1}(a)$
to
![]() $\omega ^{-1}(H)$
. The diagram
$\omega ^{-1}(H)$
. The diagram

is obviously commutative.
To prove that
![]() $\sigma $
is a homeomorphism, we notice that its inverse map
$\sigma $
is a homeomorphism, we notice that its inverse map
![]() ${\omega ^{-1}(H)\to H\times \omega ^{-1}(a)}$
is given by
${\omega ^{-1}(H)\to H\times \omega ^{-1}(a)}$
is given by
To establish the required cohomological properties of the compact version of
![]() $F_{\bar e}$
(Lemma 4.8), we need the following result whose proof we leave to the reader.
$F_{\bar e}$
(Lemma 4.8), we need the following result whose proof we leave to the reader.
Lemma 3.11. Suppose that the quotient map
![]() $E\to P\mathop {\backslash } E$
is a principal P-bundle. Then the composition
$E\to P\mathop {\backslash } E$
is a principal P-bundle. Then the composition
is a fiber bundle with fiber
![]() $Y\,{}_Q\times P$
.
$Y\,{}_Q\times P$
.
3.7 The tensor product
The above results can be applied to equivariant cohomologies. Let us suppose that all quotient maps
![]() $E\to L\mathop {\backslash } E$
,
$E\to L\mathop {\backslash } E$
,
![]() $E\to P\mathop {\backslash } E$
,
$E\to P\mathop {\backslash } E$
,
![]() $E\to R\mathop {\backslash } E$
,
$E\to R\mathop {\backslash } E$
,
![]() $E\to Q\mathop {\backslash } E$
,
$E\to Q\mathop {\backslash } E$
,
![]() $E\to M\mathop {\backslash } E$
are universal principal bundles for the respective groups. We denote by
$E\to M\mathop {\backslash } E$
are universal principal bundles for the respective groups. We denote by
![]() $\Theta $
the following composition:
$\Theta $
the following composition:

The map
![]() $\times $
is the cross product and is given by
$\times $
is the cross product and is given by
![]() $a\otimes b\mapsto \mathop{\rm pr}\nolimits _1^*(a)\cup \mathop{\rm pr}\nolimits _2^*(b)$
. The classical rule
$a\otimes b\mapsto \mathop{\rm pr}\nolimits _1^*(a)\cup \mathop{\rm pr}\nolimits _2^*(b)$
. The classical rule
makes
![]() $H^{\bullet }_L(X/R,\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }_Q(Y,\Bbbk )$
into an associative ring.
$H^{\bullet }_L(X/R,\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }_Q(Y,\Bbbk )$
into an associative ring.
Using the notation of Section 3.5, we can get the following result.
Theorem 3.12. The map
![]() $\Theta $
is a homomorphism of rings and of left
$\Theta $
is a homomorphism of rings and of left
![]() $H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-modules with respect to the canonical actions. For any Q-equivariant continuous map
$H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-modules with respect to the canonical actions. For any Q-equivariant continuous map
![]() $B:Y\to G/M$
, the map
$B:Y\to G/M$
, the map
![]() $\Theta $
is also a homomorphism of right
$\Theta $
is also a homomorphism of right
![]() $H^{\bullet }_M(\mathrm {pt},\Bbbk )$
-modules with respect to the actions twisted by B and
$H^{\bullet }_M(\mathrm {pt},\Bbbk )$
-modules with respect to the actions twisted by B and
![]() $\alpha B$
on the domain and the codomain of
$\alpha B$
on the domain and the codomain of
![]() $\Theta $
, respectively.
$\Theta $
, respectively.
Proof. The fact that
![]() $\Theta $
is a homomorphism of rings can be proved following the calculation before [Reference HatcherHa, Theorem 3.16].
$\Theta $
is a homomorphism of rings can be proved following the calculation before [Reference HatcherHa, Theorem 3.16].
Let
![]() $a\in H^{\bullet }_L(\mathrm {pt},\Bbbk )$
,
$a\in H^{\bullet }_L(\mathrm {pt},\Bbbk )$
,
![]() $m\in H^{\bullet }_L(X/R,\Bbbk )$
, and
$m\in H^{\bullet }_L(X/R,\Bbbk )$
, and
![]() $n\in H^{\bullet }_Q(Y,\Bbbk )$
. As diagram (3-13) is commutative,
$n\in H^{\bullet }_Q(Y,\Bbbk )$
. As diagram (3-13) is commutative,
 $$ \begin{align*} \Theta( a m\otimes n)&=\Theta((\mathop{\mathbf{can}}\nolimits^*( a)\cup m)\otimes n)=\varphi^*(\mathop{\rm pr}\nolimits_1^*(\mathop{\mathbf{can}}\nolimits^*( a)\cup m)\cup\mathop{\rm pr}\nolimits_2^*( n))\\ &=(\mathop{\mathbf{can}}\nolimits\mathop{\rm pr}\nolimits_1\varphi)^*( a)\cup\varphi^*(\mathop{\rm pr}\nolimits_1^*( m)\cup\mathop{\rm pr}\nolimits_2^*( n))\\&=\mathop{\mathbf{can}}\nolimits^*( a)\cup\Theta( m\otimes n) = a\Theta( m\otimes n). \end{align*} $$
$$ \begin{align*} \Theta( a m\otimes n)&=\Theta((\mathop{\mathbf{can}}\nolimits^*( a)\cup m)\otimes n)=\varphi^*(\mathop{\rm pr}\nolimits_1^*(\mathop{\mathbf{can}}\nolimits^*( a)\cup m)\cup\mathop{\rm pr}\nolimits_2^*( n))\\ &=(\mathop{\mathbf{can}}\nolimits\mathop{\rm pr}\nolimits_1\varphi)^*( a)\cup\varphi^*(\mathop{\rm pr}\nolimits_1^*( m)\cup\mathop{\rm pr}\nolimits_2^*( n))\\&=\mathop{\mathbf{can}}\nolimits^*( a)\cup\Theta( m\otimes n) = a\Theta( m\otimes n). \end{align*} $$
Now let
![]() $ m\in H^{\bullet }_L(X/R,\Bbbk )$
,
$ m\in H^{\bullet }_L(X/R,\Bbbk )$
,
![]() $ n\in H^{\bullet }_Q(Y,\Bbbk )$
, and
$ n\in H^{\bullet }_Q(Y,\Bbbk )$
, and
![]() $b\in H^{\bullet }_M(\mathrm {pt},\Bbbk )$
. As diagram (3-15) is commutative,
$b\in H^{\bullet }_M(\mathrm {pt},\Bbbk )$
. As diagram (3-15) is commutative,
 $$ \begin{align*} \Theta( m\otimes nb)&=\Theta( m\otimes (n\cup v_B^*(b)))=\varphi^*(\mathop{\rm pr}\nolimits_1^*( m)\cup\mathop{\rm pr}\nolimits_2^*( n\cup v_B^*(b)))\\ &=\varphi^*(\mathop{\rm pr}\nolimits_1^*( m)\cup \mathop{\rm pr}\nolimits_2^*( n))\cup (v_B\mathop{\rm pr}\nolimits_2\varphi)^*(b)\\ &=\Theta( m\otimes n)\cup v_{\alpha B}^*(b) =\Theta( m\otimes n)b.\\[-42pt] \end{align*} $$
$$ \begin{align*} \Theta( m\otimes nb)&=\Theta( m\otimes (n\cup v_B^*(b)))=\varphi^*(\mathop{\rm pr}\nolimits_1^*( m)\cup\mathop{\rm pr}\nolimits_2^*( n\cup v_B^*(b)))\\ &=\varphi^*(\mathop{\rm pr}\nolimits_1^*( m)\cup \mathop{\rm pr}\nolimits_2^*( n))\cup (v_B\mathop{\rm pr}\nolimits_2\varphi)^*(b)\\ &=\Theta( m\otimes n)\cup v_{\alpha B}^*(b) =\Theta( m\otimes n)b.\\[-42pt] \end{align*} $$
Now let us look more closely at the tensor product
![]() $H^{\bullet }_L(X/R,\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }_Q(Y,\Bbbk )$
. According to Section 3.1, the left factor
$H^{\bullet }_L(X/R,\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }_Q(Y,\Bbbk )$
. According to Section 3.1, the left factor
![]() $H^{\bullet }_L(X/R,\Bbbk )$
is a right
$H^{\bullet }_L(X/R,\Bbbk )$
is a right
![]() $H_R^{\bullet }(\mathrm {pt},\Bbbk )$
-module with the action twisted by A. However, the right factor
$H_R^{\bullet }(\mathrm {pt},\Bbbk )$
-module with the action twisted by A. However, the right factor
![]() $H^{\bullet }_Q(Y,\Bbbk )$
is a left
$H^{\bullet }_Q(Y,\Bbbk )$
is a left
![]() $H_Q^{\bullet }(\mathrm {pt},\Bbbk )$
-module with respect to the canonical action (Section 2.7). Moreover, the quotient maps
$H_Q^{\bullet }(\mathrm {pt},\Bbbk )$
-module with respect to the canonical action (Section 2.7). Moreover, the quotient maps
![]() $\pi _R$
and
$\pi _R$
and
![]() $\pi _Q$
, refer to Diagram (3-11), yield the following maps:
$\pi _Q$
, refer to Diagram (3-11), yield the following maps:
They allow us to define the structure of a right
![]() $H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
$H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
![]() $H^{\bullet }_L(X/R,\Bbbk )$
and of a left
$H^{\bullet }_L(X/R,\Bbbk )$
and of a left
![]() $H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
$H_P^{\bullet }(\mathrm {pt},\Bbbk )$
-module on
![]() $H^{\bullet }_Q(Y,\Bbbk )$
.
$H^{\bullet }_Q(Y,\Bbbk )$
.
Lemma 3.13. The map
![]() $\Theta $
factors through
$\Theta $
factors through
![]() $H^{\bullet }_L(X/R,\Bbbk )\otimes _{H_P^{\bullet }(\mathrm {pt},\Bbbk )}H^{\bullet }_Q(Y,\Bbbk )$
.
$H^{\bullet }_L(X/R,\Bbbk )\otimes _{H_P^{\bullet }(\mathrm {pt},\Bbbk )}H^{\bullet }_Q(Y,\Bbbk )$
.
Proof. Let
![]() $m\in H^{\bullet }_L(X/R,\Bbbk )$
,
$m\in H^{\bullet }_L(X/R,\Bbbk )$
,
![]() $n\in H^{\bullet }_Q(Y,\Bbbk )$
, and
$n\in H^{\bullet }_Q(Y,\Bbbk )$
, and
![]() $a\in H_P^{\bullet }(\mathrm {pt},\Bbbk )$
. By Lemma 3.7 and Diagram (3-18) defining
$a\in H_P^{\bullet }(\mathrm {pt},\Bbbk )$
. By Lemma 3.7 and Diagram (3-18) defining
![]() $\Theta $
,
$\Theta $
,
 $$ \begin{align*} \Theta(m a\otimes n)&=\Theta(m\pi_R^*( a)\otimes n)=\Theta(m\cup v_A^*\pi_R^*( a)\otimes n) =\varphi^*(\mathop{\rm pr}\nolimits_1^*(m\cup v_A^*\pi_R^*( a))\cup\mathop{\rm pr}\nolimits_2^*( n))\\ &=\varphi^*\mathop{\rm pr}\nolimits_1^*(m)\cup (\pi_R v_A \mathop{\rm pr}\nolimits_1 \varphi)^*( a)\cup\varphi^*\mathop{\rm pr}\nolimits_2^*( n)\\ &=\varphi^*\mathop{\rm pr}\nolimits_1^*(m)\cup (\pi_Q \mathop{\mathbf{can}}\nolimits \mathop{\rm pr}\nolimits_2\varphi)^*( a)\cup\varphi^*\mathop{\rm pr}\nolimits_2^*( n)\\ &=\varphi^*(\mathop{\rm pr}\nolimits_1^*(m)\cup \mathop{\rm pr}\nolimits_2^* \mathop{\mathbf{can}}\nolimits^* \pi_Q^*( a)\cup\mathop{\rm pr}\nolimits_2^*( n)) =\varphi^*(\mathop{\rm pr}\nolimits_1^*(m)\cup \mathop{\rm pr}\nolimits_2^*(\mathop{\mathbf{can}}\nolimits^* \pi_Q^*( a)\cup n))\\ &=\Theta(m\otimes \mathop{\mathbf{can}}\nolimits^*\pi_Q^*( a)\cup n)=\Theta(m\otimes \pi_Q^*( a) n)=\Theta(m\otimes a n).\\[-42pt] \end{align*} $$
$$ \begin{align*} \Theta(m a\otimes n)&=\Theta(m\pi_R^*( a)\otimes n)=\Theta(m\cup v_A^*\pi_R^*( a)\otimes n) =\varphi^*(\mathop{\rm pr}\nolimits_1^*(m\cup v_A^*\pi_R^*( a))\cup\mathop{\rm pr}\nolimits_2^*( n))\\ &=\varphi^*\mathop{\rm pr}\nolimits_1^*(m)\cup (\pi_R v_A \mathop{\rm pr}\nolimits_1 \varphi)^*( a)\cup\varphi^*\mathop{\rm pr}\nolimits_2^*( n)\\ &=\varphi^*\mathop{\rm pr}\nolimits_1^*(m)\cup (\pi_Q \mathop{\mathbf{can}}\nolimits \mathop{\rm pr}\nolimits_2\varphi)^*( a)\cup\varphi^*\mathop{\rm pr}\nolimits_2^*( n)\\ &=\varphi^*(\mathop{\rm pr}\nolimits_1^*(m)\cup \mathop{\rm pr}\nolimits_2^* \mathop{\mathbf{can}}\nolimits^* \pi_Q^*( a)\cup\mathop{\rm pr}\nolimits_2^*( n)) =\varphi^*(\mathop{\rm pr}\nolimits_1^*(m)\cup \mathop{\rm pr}\nolimits_2^*(\mathop{\mathbf{can}}\nolimits^* \pi_Q^*( a)\cup n))\\ &=\Theta(m\otimes \mathop{\mathbf{can}}\nolimits^*\pi_Q^*( a)\cup n)=\Theta(m\otimes \pi_Q^*( a) n)=\Theta(m\otimes a n).\\[-42pt] \end{align*} $$
We denote the map induced by
![]() $\Theta $
as follows:
$\Theta $
as follows:
We make the left-hand side into a ring using product (3-19).
Corollary 3.14. The map
![]() $\theta $
is a homomorphism of rings and of left
$\theta $
is a homomorphism of rings and of left
![]() $H^{\bullet }_L(\mathrm {pt},\Bbbk )$
- modules with respect to the canonical actions. For any Q-equivariant continuous map
$H^{\bullet }_L(\mathrm {pt},\Bbbk )$
- modules with respect to the canonical actions. For any Q-equivariant continuous map
![]() ${B:Y\to G/M}$
, the map
${B:Y\to G/M}$
, the map
![]() $\theta $
is also a homomorphism of right
$\theta $
is also a homomorphism of right
![]() $H^{\bullet }_M(\mathrm {pt},\Bbbk )$
-modules with respect to the actions twisted by B and
$H^{\bullet }_M(\mathrm {pt},\Bbbk )$
-modules with respect to the actions twisted by B and
![]() $\alpha B$
on the domain and the codomain of
$\alpha B$
on the domain and the codomain of
![]() $\theta $
, respectively.
$\theta $
, respectively.
Proof. The result follows from Theorem 3.12.
4 The isomorphism
We prove that the map
![]() $\theta $
introduced in Section 3 is an isomorphism under certain restrictions, which we are going to formulate. First, we assume the following two conditions:
$\theta $
introduced in Section 3 is an isomorphism under certain restrictions, which we are going to formulate. First, we assume the following two conditions:
-
(I) the ring
 $\Bbbk $
has finite global dimension
$\Bbbk $
has finite global dimension
 $\mathop {\mathrm {gld}}(\Bbbk )$
;
$\mathop {\mathrm {gld}}(\Bbbk )$
; -
(II) G is a compact Lie group and L, R, P, Q, M are closed subgroups.
These conditions are supposed to hold for the rest of the paper.
4.1 Künneth formula
Let
![]() $X_1,\ldots ,X_m$
be topological spaces such that
$X_1,\ldots ,X_m$
be topological spaces such that
![]() $H^n(X_j,\Bbbk )$
are free of finite rank for all
$H^n(X_j,\Bbbk )$
are free of finite rank for all
![]() $n\leqslant N$
and
$n\leqslant N$
and
![]() $j=2,\ldots ,m$
. Then for any
$j=2,\ldots ,m$
. Then for any
![]() $n\leqslant N-\mathop {\mathrm {gld}}(\Bbbk )$
, we have the isomorphism
$n\leqslant N-\mathop {\mathrm {gld}}(\Bbbk )$
, we have the isomorphism
 $$ \begin{align*} \bigoplus_{i_1+\cdots+i_m=n}H^{i_1}(X_1,\Bbbk)\otimes\cdots\otimes H^{i_m}(X_m,\Bbbk)\stackrel\sim\to H^n(X_1\times\cdots\times X_m,\Bbbk) \end{align*} $$
$$ \begin{align*} \bigoplus_{i_1+\cdots+i_m=n}H^{i_1}(X_1,\Bbbk)\otimes\cdots\otimes H^{i_m}(X_m,\Bbbk)\stackrel\sim\to H^n(X_1\times\cdots\times X_m,\Bbbk) \end{align*} $$
that is given by the cross product
![]() $a_1\otimes \cdots \otimes a_m\mapsto p_1^*(a_1)\cup \cdots \cup p_m^*(a_m)$
.
$a_1\otimes \cdots \otimes a_m\mapsto p_1^*(a_1)\cup \cdots \cup p_m^*(a_m)$
.
This isomorphism and Proposition 2.4 prove that
![]() $H^n((E^N)^m,\Bbbk )=0$
for any
$H^n((E^N)^m,\Bbbk )=0$
for any
![]() ${0<n\leqslant 2(N-{\mathfrak n}_{G})}$
and natural number m. Moreover, the space
${0<n\leqslant 2(N-{\mathfrak n}_{G})}$
and natural number m. Moreover, the space
![]() $E^m$
is contractible. Hence, we get the following result similar to Proposition 2.6.
$E^m$
is contractible. Hence, we get the following result similar to Proposition 2.6.
Proposition 4.1. Suppose that for each
![]() $i=1,\ldots ,m$
, there is a left
$i=1,\ldots ,m$
, there is a left
![]() $L^{(i)}$
-space
$L^{(i)}$
-space
![]() $X^{(i)}$
for a closed subgroup
$X^{(i)}$
for a closed subgroup
![]() $L^{(i)}$
of G. Then the restriction map
$L^{(i)}$
of G. Then the restriction map
 $$ \begin{align*} H^n((X^{(1)}&\,{}_{L^{(1)}}\!\times E)\times\cdots\times(X^{(m)}\,{}_{L^{(m)}}\!\times E),\Bbbk)\\&\to H^n((X^{(1)}\,{}_{L^{(1)}}\!\times E^N)\times\cdots\times(X^{(m)}\,{}_{L^{(m)}}\!\times E^N),\Bbbk) \end{align*} $$
$$ \begin{align*} H^n((X^{(1)}&\,{}_{L^{(1)}}\!\times E)\times\cdots\times(X^{(m)}\,{}_{L^{(m)}}\!\times E),\Bbbk)\\&\to H^n((X^{(1)}\,{}_{L^{(1)}}\!\times E^N)\times\cdots\times(X^{(m)}\,{}_{L^{(m)}}\!\times E^N),\Bbbk) \end{align*} $$
is an isomorphism for
![]() $n\leqslant 2(N-{\mathfrak n}_{G})$
.
$n\leqslant 2(N-{\mathfrak n}_{G})$
.
4.2 An auxiliary lemma
We are going to use the following result proved in [Reference ShchigolevS2, Lemma 18].
Proposition 4.2. Let S be a locally compact Hausdorff space,
![]() $p:S\to T$
be a fiber bundle with fiber F,
$p:S\to T$
be a fiber bundle with fiber F,
![]() $t\in T$
be a point, and
$t\in T$
be a point, and
![]() $\Bbbk $
be a commutative ring. Suppose that T is compact, Hausdorff, connected, and simply connected, all
$\Bbbk $
be a commutative ring. Suppose that T is compact, Hausdorff, connected, and simply connected, all
![]() $H_c^n(F,\Bbbk )$
are free of finite rank,
$H_c^n(F,\Bbbk )$
are free of finite rank,
![]() $H_c^n(F,\Bbbk )=0$
for odd n, and
$H_c^n(F,\Bbbk )=0$
for odd n, and
![]() $H_c^n(T,\Bbbk )=0$
for odd
$H_c^n(T,\Bbbk )=0$
for odd
![]() $n\leqslant N$
.
$n\leqslant N$
.
Then the following are true.
-
(1) The restriction map
 $H_c^n(S,\Bbbk )\to H_c^n(p^{-1}(t),\Bbbk )$
is surjective for all
$H_c^n(S,\Bbbk )\to H_c^n(p^{-1}(t),\Bbbk )$
is surjective for all
 $n<N$
.
$n<N$
. -
(2)
 $H_c^n(S-p^{-1}(t),\Bbbk )=0$
for odd
$H_c^n(S-p^{-1}(t),\Bbbk )=0$
for odd
 $n<N$
.
$n<N$
. -
(3) If
 $H_c^n(T,\Bbbk )$
are free of finite rank for
$H_c^n(T,\Bbbk )$
are free of finite rank for
 $n\leqslant N$
, then the
$n\leqslant N$
, then the
 $\Bbbk $
-modules
$\Bbbk $
-modules
 $H_c^n(S-p^{-1}(t),\Bbbk )$
are also free of finite rank for
$H_c^n(S-p^{-1}(t),\Bbbk )$
are also free of finite rank for
 $n<N$
.
$n<N$
.
4.3 Leray spectral sequence with compact support
For any topological space S and a commutative ring
![]() $\Bbbk $
, we denote by
$\Bbbk $
, we denote by ![]() the constant sheaf on S. In the formulation of the next result (refer, for example, to [Reference DimcaDi, Corollary 2.3.24]), we use the direct image functor
the constant sheaf on S. In the formulation of the next result (refer, for example, to [Reference DimcaDi, Corollary 2.3.24]), we use the direct image functor
![]() $f_!$
with compact (proper) support for a continuous map f between locally compact spaces and its qth right derived functor
$f_!$
with compact (proper) support for a continuous map f between locally compact spaces and its qth right derived functor
![]() $R^q\!f_!$
[Reference Kashiwara and SchapiraKS, II.2.5, II.2.6].
$R^q\!f_!$
[Reference Kashiwara and SchapiraKS, II.2.5, II.2.6].
Proposition 4.3. Let
![]() $f:S\to T$
be a continuous map between locally compact Hausdorff spaces. There exists a first quadrant spectral sequence with the second page
$f:S\to T$
be a continuous map between locally compact Hausdorff spaces. There exists a first quadrant spectral sequence with the second page ![]() converging to
converging to
![]() $H_c^{p+q}(S,\Bbbk )$
.
$H_c^{p+q}(S,\Bbbk )$
.
Let us consider the case where
![]() $f:S\to T$
is a fiber bundle with fiber F. In this case, the sheaf
$f:S\to T$
is a fiber bundle with fiber F. In this case, the sheaf ![]() is locally constant. Indeed, applying the proper base change [Reference Kashiwara and SchapiraKS, Proposition 2.6.7], we reduce the problem to the case where
is locally constant. Indeed, applying the proper base change [Reference Kashiwara and SchapiraKS, Proposition 2.6.7], we reduce the problem to the case where
![]() $S=F\times T$
and
$S=F\times T$
and
![]() $f=\mathop{\rm pr}\nolimits _2$
. Applying the proper base change to the Cartesian square
$f=\mathop{\rm pr}\nolimits _2$
. Applying the proper base change to the Cartesian square

we get
Taking the qth cohomology,
Corollary 4.4. Let
![]() $f:S\to T$
be a fiber bundle with fiber F, where S and T are locally compact and Hausdorff. Suppose that
$f:S\to T$
be a fiber bundle with fiber F, where S and T are locally compact and Hausdorff. Suppose that
![]() $H^q_c(F,\Bbbk )$
is free of finite rank for any q and T is simply connected. Then there exists a first quadrant spectral sequence with the second page
$H^q_c(F,\Bbbk )$
is free of finite rank for any q and T is simply connected. Then there exists a first quadrant spectral sequence with the second page
![]() $E_2^{p,q}=H_c^p(T,\Bbbk )\,{\otimes }_{\Bbbk }\, H^q_c(F,\Bbbk )$
converging to
$E_2^{p,q}=H_c^p(T,\Bbbk )\,{\otimes }_{\Bbbk }\, H^q_c(F,\Bbbk )$
converging to
![]() $H_c^{p+q}(S,\Bbbk )$
.
$H_c^{p+q}(S,\Bbbk )$
.
In particular, if
![]() $H_c^{\bullet }(T,\Bbbk )$
and
$H_c^{\bullet }(T,\Bbbk )$
and
![]() $H^{\bullet }_c(F,\Bbbk )$
are free of finite rank in each degree and vanish in odd degrees, then
$H^{\bullet }_c(F,\Bbbk )$
are free of finite rank in each degree and vanish in odd degrees, then
![]() $ H_c^{\bullet }(S,\Bbbk )\cong H_c^{\bullet }(T,\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }_c(F,\Bbbk ) $
.
$ H_c^{\bullet }(S,\Bbbk )\cong H_c^{\bullet }(T,\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }_c(F,\Bbbk ) $
.
Proof. By the preceding arguments, we know that the sheaf ![]() is locally constant. It follows from [Reference IversenI, Proposition IV.4.20] that
is locally constant. It follows from [Reference IversenI, Proposition IV.4.20] that ![]() is constant. Thus, by (4-1), it is isomorphic to
is constant. Thus, by (4-1), it is isomorphic to ![]() , where n is the rank of
, where n is the rank of
![]() $H^q_c(F,\Bbbk )$
. To prove the first result, it suffices to substitute this sheaf into the spectral sequence of Proposition 4.3.
$H^q_c(F,\Bbbk )$
. To prove the first result, it suffices to substitute this sheaf into the spectral sequence of Proposition 4.3.
The second result follows from the fact that this spectral sequence collapses at the second page and
![]() $\mathop {\rm Ext}^1(\Bbbk ,M)=0$
for any
$\mathop {\rm Ext}^1(\Bbbk ,M)=0$
for any
![]() $\Bbbk $
-module M.
$\Bbbk $
-module M.
Corollary 4.5. Let L be a connected compact Lie group such that
![]() $H^{\bullet }_L(\mathrm {pt},\Bbbk )$
is free of finite rank in each degree and vanishes in odd degrees. For any compact Hausdorff L-space X such that
$H^{\bullet }_L(\mathrm {pt},\Bbbk )$
is free of finite rank in each degree and vanishes in odd degrees. For any compact Hausdorff L-space X such that
![]() $H^{\bullet }(X,\Bbbk )$
is free of finite rank in each degree and vanishes in odd degrees, there is an isomorphism
$H^{\bullet }(X,\Bbbk )$
is free of finite rank in each degree and vanishes in odd degrees, there is an isomorphism
![]() $ H^{\bullet }_L(X,\Bbbk )\cong H^{\bullet }_L(\mathrm {pt},\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }(X,\Bbbk ) $
.
$ H^{\bullet }_L(X,\Bbbk )\cong H^{\bullet }_L(\mathrm {pt},\Bbbk )\,{\otimes }_{\Bbbk }\, H^{\bullet }(X,\Bbbk ) $
.
Proof. We assume that
![]() $G=L$
and the spaces
$G=L$
and the spaces
![]() $E^N$
are chosen as in Section 2.8. Let n be a nonnegative integer. We choose any N such that
$E^N$
are chosen as in Section 2.8. Let n be a nonnegative integer. We choose any N such that
![]() $n<2(N-{\mathfrak n}_{G})$
. Applying Corollary 4.4 together with Corollary 2.5 to the canonical projection
$n<2(N-{\mathfrak n}_{G})$
. Applying Corollary 4.4 together with Corollary 2.5 to the canonical projection
![]() ${X\,{}_L\!\times \,E^N\to L\mathop {\backslash } E^N}$
, we get the spectral sequence with the following second page:
${X\,{}_L\!\times \,E^N\to L\mathop {\backslash } E^N}$
, we get the spectral sequence with the following second page:
![]() $ E_2^{p,q}=H^p(L\mathop {\backslash } E^N,\Bbbk )\,{\otimes }_{\Bbbk }\, H^q(X,\Bbbk ) $
. By Proposition 2.6, we get that
$ E_2^{p,q}=H^p(L\mathop {\backslash } E^N,\Bbbk )\,{\otimes }_{\Bbbk }\, H^q(X,\Bbbk ) $
. By Proposition 2.6, we get that
![]() $ {E_2^{p,q}=H_L^p(\mathrm {pt},\Bbbk )\,{\otimes }_{\Bbbk }\, H^q(X,\Bbbk ) }$
for
$ {E_2^{p,q}=H_L^p(\mathrm {pt},\Bbbk )\,{\otimes }_{\Bbbk }\, H^q(X,\Bbbk ) }$
for
![]() $p\leqslant 2(N-{\mathfrak n}_{G})$
. The differentials coming to and from each
$p\leqslant 2(N-{\mathfrak n}_{G})$
. The differentials coming to and from each
![]() $E_r^{p,q}$
for
$E_r^{p,q}$
for
![]() $p+q<2(N-{\mathfrak n}_{G})$
are zero. Therefore,
$p+q<2(N-{\mathfrak n}_{G})$
are zero. Therefore,
![]() $E_\infty ^{p,q}=E_2^{p,q}$
. As no extension problem emerges in this case,
$E_\infty ^{p,q}=E_2^{p,q}$
. As no extension problem emerges in this case,
 $$ \begin{align*} H^n(X\,{}_L\!\times\,E^N)=\bigoplus_{p+q=n}H_L^p(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^q(X,\Bbbk) \end{align*} $$
$$ \begin{align*} H^n(X\,{}_L\!\times\,E^N)=\bigoplus_{p+q=n}H_L^p(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^q(X,\Bbbk) \end{align*} $$
for
![]() $n<2(N-{\mathfrak n}_{G})$
. Proposition 2.6 applied to the left-hand side finishes the proof.
$n<2(N-{\mathfrak n}_{G})$
. Proposition 2.6 applied to the left-hand side finishes the proof.
4.4 Restrictions on groups and spaces
We consider the following additional conditions:
-
(III) P, Q, and L are connected;
-
(IV)
 $H^n_P(\mathrm {pt},\Bbbk )$
,
$H^n_P(\mathrm {pt},\Bbbk )$
,
 $H^n_Q(\mathrm {pt},\Bbbk )$
, and
$H^n_Q(\mathrm {pt},\Bbbk )$
, and
 $H^n_L(\mathrm {pt},\Bbbk )$
are free of finite rank and vanish for odd n;
$H^n_L(\mathrm {pt},\Bbbk )$
are free of finite rank and vanish for odd n; -
(V) X and Y are compact and Hausdorff;
-
(VI) the cohomologies of
 $X/R$
,
$X/R$
,
 $P/Q$
, and Y with coefficients in
$P/Q$
, and Y with coefficients in
 $\Bbbk $
are free of finite rank in each degree and vanish in odd degrees;
$\Bbbk $
are free of finite rank in each degree and vanish in odd degrees; -
(VII)
 $X/R$
and
$X/R$
and
 $P/Q$
are simply connected;
$P/Q$
are simply connected; -
(VIII) the quotient map
 $X\to X/R$
is a principal R-bundle.
$X\to X/R$
is a principal R-bundle.
By Proposition 2.2 and Condition (VIII), these conditions imply that the natural projections
are fiber bundles with fibers Y and
![]() $P\mathop {\times }\limits _Q Y$
, respectively. These projections are given by
$P\mathop {\times }\limits _Q Y$
, respectively. These projections are given by
![]() $[p:y]\mapsto pQ$
and
$[p:y]\mapsto pQ$
and
![]() $[x:p:y]\mapsto xR$
. To apply Proposition 2.2, we can consider the isomorphisms
$[x:p:y]\mapsto xR$
. To apply Proposition 2.2, we can consider the isomorphisms
![]() $P\mathop {\times }\limits _Q Y\cong Y\,{}_Q\!\times P$
and
$P\mathop {\times }\limits _Q Y\cong Y\,{}_Q\!\times P$
and
![]() $X\mathop {\times }\limits _R P\mathop {\times }\limits _Q Y\cong (P\mathop {\times }\limits _Q Y)\,{}_R\!\times X$
given by
$X\mathop {\times }\limits _R P\mathop {\times }\limits _Q Y\cong (P\mathop {\times }\limits _Q Y)\,{}_R\!\times X$
given by
![]() $[p:y]\mapsto Q(y,p^{-1})$
and
$[p:y]\mapsto Q(y,p^{-1})$
and
![]() $[x:p:y]\mapsto R([p:y],x)$
, respectively. Here we consider X as a left R-space under the following action:
$[x:p:y]\mapsto R([p:y],x)$
, respectively. Here we consider X as a left R-space under the following action:
![]() $rx=xr^{-1}$
. We also have the isomorphisms
$rx=xr^{-1}$
. We also have the isomorphisms
![]() $R\mathop {\backslash } X\cong X/R$
and
$R\mathop {\backslash } X\cong X/R$
and
![]() $Q\mathop {\backslash } P\cong P/Q$
given by
$Q\mathop {\backslash } P\cong P/Q$
given by
![]() $Rx\mapsto xR$
and
$Rx\mapsto xR$
and
![]() ${Qp\mapsto p^{-1}Q}$
, respectively. Our projections become canonical in the sense of Section 2.4 if we apply the identifications described just above. By Conditions (II) and (V), we get that all four spaces in the left- and right-hand sides of the above formulas are compact and Hausdorff. Applying Corollary 4.4 to the first sequence and using Conditions (VI) and (VII),
${Qp\mapsto p^{-1}Q}$
, respectively. Our projections become canonical in the sense of Section 2.4 if we apply the identifications described just above. By Conditions (II) and (V), we get that all four spaces in the left- and right-hand sides of the above formulas are compact and Hausdorff. Applying Corollary 4.4 to the first sequence and using Conditions (VI) and (VII),
Hence, we also get that the cohomology in the left-hand side vanishes in odd degrees and is free of finite rank in each degree. Now applying Corollary 4.4 to the second sequence and using Conditions (VI) and (VII) again,
 $$ \begin{align}H^{\bullet}(X\mathop{\times}\limits_R P\mathop{\times}\limits_Q Y,\Bbbk)&\cong H^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P\mathop{\times}\limits_Q Y,\Bbbk)\nonumber\\&\cong H^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk). \end{align} $$
$$ \begin{align}H^{\bullet}(X\mathop{\times}\limits_R P\mathop{\times}\limits_Q Y,\Bbbk)&\cong H^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P\mathop{\times}\limits_Q Y,\Bbbk)\nonumber\\&\cong H^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk). \end{align} $$
This cohomology also vanishes in odd degrees and is free of finite rank in each degree.
Lemma 4.6. The space
![]() $(X/R)\,{}_L\!\times \,E^N$
is simply connected.
$(X/R)\,{}_L\!\times \,E^N$
is simply connected.
Proof. By Proposition 2.2,
![]() $(X/R)\,{}_L\!\times \,E^N\to L\mathop {\backslash } E^N$
is a fiber bundle with fiber
$(X/R)\,{}_L\!\times \,E^N\to L\mathop {\backslash } E^N$
is a fiber bundle with fiber
![]() $X/R$
. Therefore, the result follows from Condition (VII) and the long exact sequence of homotopy groups
$X/R$
. Therefore, the result follows from Condition (VII) and the long exact sequence of homotopy groups
The last equality follows from Corollary 2.5.
4.5 Surjectivity
We use here the abbreviations (refer to Remark 2.8)
Lemma 4.7.
![]() $H^n((X/R)\;{}_L\!\times E^N,\Bbbk )=H^n(Z\;{}_L\!\times E^N,\Bbbk )=0$
for odd
$H^n((X/R)\;{}_L\!\times E^N,\Bbbk )=H^n(Z\;{}_L\!\times E^N,\Bbbk )=0$
for odd
![]() $n\leqslant 2(N-{\mathfrak n}_{G})$
.
$n\leqslant 2(N-{\mathfrak n}_{G})$
.
Proof. The result follows from Proposition 2.6, Conditions (III), (IV), (VI), (4-2), and Corollary 4.5.
We are going to study the cohomologies with compact support of the spaces
![]() $C^N$
. First, we study the cohomology of the fiber
$C^N$
. First, we study the cohomology of the fiber
![]() $F^N_{\bar e}$
; refer to Lemma 3.10 and Remark 2.8.
$F^N_{\bar e}$
; refer to Lemma 3.10 and Remark 2.8.
Lemma 4.8. For any
![]() $n<2(N-{\mathfrak n}_{G})$
and
$n<2(N-{\mathfrak n}_{G})$
and
![]() $\bar e\in P\mathop {\backslash } E^N$
, the cohomology
$\bar e\in P\mathop {\backslash } E^N$
, the cohomology
![]() $H_c^n(F^N_{\bar e},\Bbbk )$
is free of finite rank and vanishes if n is odd.
$H_c^n(F^N_{\bar e},\Bbbk )$
is free of finite rank and vanishes if n is odd.
Proof. It suffices to apply Parts (2) and (3) of Proposition 4.2 to the fiber bundle
![]() $\pi _Q\mathop {\mathbf {can}}\nolimits :Y\,{}_Q\!\times \,E^N\to P\mathop {\backslash } E^N$
with fiber
$\pi _Q\mathop {\mathbf {can}}\nolimits :Y\,{}_Q\!\times \,E^N\to P\mathop {\backslash } E^N$
with fiber
![]() $Y\,{}_Q\times P\cong P\mathop {\times }\limits _Q Y$
; refer to Lemma 3.11.
$Y\,{}_Q\times P\cong P\mathop {\times }\limits _Q Y$
; refer to Lemma 3.11.
Lemma 4.9.
![]() $H_c^n(C^N,\Bbbk )=0$
for odd
$H_c^n(C^N,\Bbbk )=0$
for odd
![]() $n<2(N-{\mathfrak n}_{G})$
.
$n<2(N-{\mathfrak n}_{G})$
.
Proof. In view of Lemmas 4.6 and 4.8, we can apply Corollary 4.4 to the projection
![]() $\omega ^N$
as in Lemma 3.10 (refer to Remark 2.8 about the notation). The second page of this spectral sequence is equal to
$\omega ^N$
as in Lemma 3.10 (refer to Remark 2.8 about the notation). The second page of this spectral sequence is equal to
if
![]() $q<2(N-{\mathfrak n}_{G})$
. It follows from Lemmas 4.7 and 4.8 that
$q<2(N-{\mathfrak n}_{G})$
. It follows from Lemmas 4.7 and 4.8 that
![]() $E_r^{p,q}=0$
for any finite
$E_r^{p,q}=0$
for any finite
![]() $r\geqslant 2$
if
$r\geqslant 2$
if
![]() $p+q<2(N-{\mathfrak n}_{G})$
and either of p or q is odd. Thus, this fact is also true for
$p+q<2(N-{\mathfrak n}_{G})$
and either of p or q is odd. Thus, this fact is also true for
![]() $r=\infty $
.
$r=\infty $
.
Corollary 4.10. For any
![]() $n<2(N-{\mathfrak n}_{G})-1$
, the map
$n<2(N-{\mathfrak n}_{G})-1$
, the map
is surjective.
Corollary 4.11. The map
![]() $\theta $
is surjective.
$\theta $
is surjective.
Proof. Under our assumption (VI), the left map (cross product) in (3-18) is an isomorphism by the Künneth formula. Therefore, it suffices to prove that
![]() $\varphi ^*$
is surjective. In each degree, this fact follows from Corollary 4.10 and Proposition 4.1 for N big enough.
$\varphi ^*$
is surjective. In each degree, this fact follows from Corollary 4.10 and Proposition 4.1 for N big enough.
Lemma 4.12. In each degree, the homomorphism
![]() $\theta $
induces a map between free
$\theta $
induces a map between free
![]() $\Bbbk $
-modules of the same finite rank.
$\Bbbk $
-modules of the same finite rank.
Proof. As is noted in [Reference JantzenJ, 1.5(3)], we have
![]() $H_P^{\bullet }(P/Q,\Bbbk )\cong H_Q(\mathrm {pt},\Bbbk )$
. Then the result follows from the following computation based on (4-2) and Corollary 4.5:
$H_P^{\bullet }(P/Q,\Bbbk )\cong H_Q(\mathrm {pt},\Bbbk )$
. Then the result follows from the following computation based on (4-2) and Corollary 4.5:
 $$ \begin{align*} &H^{\bullet}_L(X/R,\Bbbk)\otimes_{H_P^{\bullet}(\mathrm{pt},\Bbbk)}H^{\bullet}_Q(Y,\Bbbk)\\&\quad\cong H_L^{\bullet}(X/R,\Bbbk)\otimes_{H_P^{\bullet}(\mathrm{pt},\Bbbk)}H_Q^{\bullet}(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(X/R,\Bbbk)\otimes_{H_P^{\bullet}(\mathrm{pt},\Bbbk)}H^{\bullet}_P(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(X\mathop{\times}\limits_R P\mathop{\times}\limits_Q Y,\Bbbk)\cong H_L^{\bullet}(X\mathop{\times}\limits_R P\mathop{\times}\limits_Q Y,\Bbbk).\\[-43pt] \end{align*} $$
$$ \begin{align*} &H^{\bullet}_L(X/R,\Bbbk)\otimes_{H_P^{\bullet}(\mathrm{pt},\Bbbk)}H^{\bullet}_Q(Y,\Bbbk)\\&\quad\cong H_L^{\bullet}(X/R,\Bbbk)\otimes_{H_P^{\bullet}(\mathrm{pt},\Bbbk)}H_Q^{\bullet}(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(X/R,\Bbbk)\otimes_{H_P^{\bullet}(\mathrm{pt},\Bbbk)}H^{\bullet}_P(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(X/R,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(P/Q,\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(Y,\Bbbk)\\ &\quad\cong H_L^{\bullet}(\mathrm{pt},\Bbbk)\,{\otimes}_{\Bbbk}\, H^{\bullet}(X\mathop{\times}\limits_R P\mathop{\times}\limits_Q Y,\Bbbk)\cong H_L^{\bullet}(X\mathop{\times}\limits_R P\mathop{\times}\limits_Q Y,\Bbbk).\\[-43pt] \end{align*} $$
To prove the main result of this section, let us remember the following proposition.
Proposition 4.13 [Reference RotmanR, Theorem 3.6].
Let R be a commutative ring and let M be a finitely generated R-module. If
![]() $\beta :M\to M$
is an epic R-module homomorphism, then
$\beta :M\to M$
is an epic R-module homomorphism, then
![]() $\beta $
is an isomorphism.
$\beta $
is an isomorphism.
Theorem 4.14. Suppose that Conditions (I)–(VIII) are satisfied. Then
![]() $\theta $
is an isomorphism of rings and left
$\theta $
is an isomorphism of rings and left
![]() $H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-modules. If M is another closed subgroup of G and
$H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-modules. If M is another closed subgroup of G and
![]() $B:Y\to G/M$
is a Q-equivariant continuous map, then
$B:Y\to G/M$
is a Q-equivariant continuous map, then
![]() $\theta $
is an isomorphism of rings and
$\theta $
is an isomorphism of rings and
![]() $H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-
$H^{\bullet }_L(\mathrm {pt},\Bbbk )$
-
![]() $H^{\bullet }_M(\mathrm {pt},\Bbbk )$
-bimodules.
$H^{\bullet }_M(\mathrm {pt},\Bbbk )$
-bimodules.
Proof. Working in one degree at a time and composing
![]() $\theta $
with the isomorphism of Lemma 4.12, we get a surjective homomorphism from a finitely generated
$\theta $
with the isomorphism of Lemma 4.12, we get a surjective homomorphism from a finitely generated
![]() $\Bbbk $
-module to itself. Now it suffices to apply Proposition 4.13 to prove that
$\Bbbk $
-module to itself. Now it suffices to apply Proposition 4.13 to prove that
![]() $\theta $
is bijective. The remaining claims follow from Corollary 3.14.
$\theta $
is bijective. The remaining claims follow from Corollary 3.14.
We consider below two basic examples of how this theorem can be applied.
4.6 Equivariant cohomology of the flag variety
Let G be a semisimple compact Lie group and K be a maximal torus in G (as in Section 2.14).
Suppose that the order
![]() $|W|$
of the Weyl group is invertible in
$|W|$
of the Weyl group is invertible in
![]() $\Bbbk $
. The map
$\Bbbk $
. The map
![]() ${\pi _K:K\mathop {\backslash } E\to G\mathop {\backslash } E}$
given by
${\pi _K:K\mathop {\backslash } E\to G\mathop {\backslash } E}$
given by
![]() $\pi _K(Ke)=Ge$
induces the isomorphism
$\pi _K(Ke)=Ge$
induces the isomorphism
where the right-hand side is the subring of W-invariants (refer to the proof of [Reference Brion, Broer, Daigneault and SabidussiBr, Proposition 1]).
We are going to apply the results of the previous sections to the following data
![]() $X=G$
,
$X=G$
,
![]() $Y=G/K$
,
$Y=G/K$
,
![]() $P=R=Q=G$
,
$P=R=Q=G$
,
![]() $L=M=K$
, letting
$L=M=K$
, letting
![]() $\alpha :X\to G$
and
$\alpha :X\to G$
and
![]() $B:Y\to G/M$
be the identity maps on G and
$B:Y\to G/M$
be the identity maps on G and
![]() $G/K$
, respectively. The induced map
$G/K$
, respectively. The induced map
![]() $A:X/R\to G/R$
is then the identity map on
$A:X/R\to G/R$
is then the identity map on
![]() $G/G$
. By Theorem 4.14, Condition (IV) being guaranteed by (4-3), and the calculation of
$G/G$
. By Theorem 4.14, Condition (IV) being guaranteed by (4-3), and the calculation of
![]() $H_K^{\bullet }(\mathrm {pt},\Bbbk )$
, the homomorphism
$H_K^{\bullet }(\mathrm {pt},\Bbbk )$
, the homomorphism
![]() $\theta $
is an isomorphism and
$\theta $
is an isomorphism and
As
![]() $G/G\cong \mathrm {pt}$
and the map
$G/G\cong \mathrm {pt}$
and the map
![]() $v_B:(G/K)\,{}_G\!\times \,E\to K\mathop {\backslash } E$
is a homeomorphism [Reference JantzenJ, 1.5], we get by (4-3) the well-known isomorphism of rings and bimodules
$v_B:(G/K)\,{}_G\!\times \,E\to K\mathop {\backslash } E$
is a homeomorphism [Reference JantzenJ, 1.5], we get by (4-3) the well-known isomorphism of rings and bimodules
with the canonical action of
![]() $H_K^{\bullet }(\mathrm {pt},\Bbbk )$
on the left and on the right.
$H_K^{\bullet }(\mathrm {pt},\Bbbk )$
on the left and on the right.
4.7 Standard bimodules
We continue to work here with the same groups G and K as in the previous section. Let us denote
This is the
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodule with respect to the cup product. For any
${\mathcal R}$
-bimodule with respect to the cup product. For any
![]() $w\in W$
, we denote by
$w\in W$
, we denote by
![]() ${\mathcal R}_w$
the
${\mathcal R}_w$
the
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodule equal to
${\mathcal R}$
-bimodule equal to
![]() ${\mathcal R}$
as an abelian group whose left action
${\mathcal R}$
as an abelian group whose left action
![]() $\cdot $
is untwisted and right action
$\cdot $
is untwisted and right action
![]() $\cdot _w$
is twisted by w:
$\cdot _w$
is twisted by w:
The construction of this bimodule naturally arises as a special case of the twisted action described in Section 3.1. Indeed, let
![]() $A_w:\mathrm {pt}\to G/K$
be the map with the value
$A_w:\mathrm {pt}\to G/K$
be the map with the value
![]() $wK$
. Then we get the map
$wK$
. Then we get the map
![]() $v_{A_w}:K\mathop {\backslash } E\cong \mathrm {pt}\,{}_K\!\times \,E\to K\mathop {\backslash } E$
, which coincides with the map
$v_{A_w}:K\mathop {\backslash } E\cong \mathrm {pt}\,{}_K\!\times \,E\to K\mathop {\backslash } E$
, which coincides with the map
![]() $K\mathop {\backslash } \rho _{w^{-1}}$
described in Section 2.14. By (2-8), we get that the right action on
$K\mathop {\backslash } \rho _{w^{-1}}$
described in Section 2.14. By (2-8), we get that the right action on
![]() $H_K^{\bullet }(\mathrm {pt},\Bbbk )$
on itself twisted by
$H_K^{\bullet }(\mathrm {pt},\Bbbk )$
on itself twisted by
![]() $A_w$
coincides with
$A_w$
coincides with
![]() $\cdot _w$
.
$\cdot _w$
.
Now let us compute the tensor product of these bimodules with the help of the isomorphism
![]() $\theta $
. Let
$\theta $
. Let
![]() $X=K$
,
$X=K$
,
![]() $Y=\mathrm {pt}$
,
$Y=\mathrm {pt}$
,
![]() $L=R=P=Q=N=M=K$
, and
$L=R=P=Q=N=M=K$
, and
![]() $\alpha :X\to G$
be the map defined by
$\alpha :X\to G$
be the map defined by
![]() $\alpha (k)=\dot {w}k$
. The last map becomes K-K-equivariant if we consider the right action of K on X by multiplication and define the left action by
$\alpha (k)=\dot {w}k$
. The last map becomes K-K-equivariant if we consider the right action of K on X by multiplication and define the left action by
![]() $k'\ast k=\dot {w}^{-1}k'\dot {w}k$
. Using this map
$k'\ast k=\dot {w}^{-1}k'\dot {w}k$
. Using this map
![]() $\alpha $
, we define the map
$\alpha $
, we define the map
![]() $A:\mathrm {pt}\cong K/K\to G/K$
by (3-9). Obviously,
$A:\mathrm {pt}\cong K/K\to G/K$
by (3-9). Obviously,
![]() $A=A_w$
.
$A=A_w$
.
Let us choose any
![]() $w'\in W$
and set
$w'\in W$
and set
![]() $B=A_{w'}$
. By (3-14),
$B=A_{w'}$
. By (3-14),
Hence,
![]() $\alpha B=A_{ww'}$
under the identification
$\alpha B=A_{ww'}$
under the identification
![]() $K\mathop {\times }\limits _KK\mathop {\times }\limits _K\mathrm {pt}\cong \mathrm {pt}$
.
$K\mathop {\times }\limits _KK\mathop {\times }\limits _K\mathrm {pt}\cong \mathrm {pt}$
.
As P, Q, L are connected,
![]() $X/R$
,
$X/R$
,
![]() $P/Q$
, Y are singletons, and
$P/Q$
, Y are singletons, and
![]() $H_K^{\bullet }(\mathrm {pt},\Bbbk )$
is the polynomial ring in finitely many variables of the second degree, Conditions (I)–(VIII) are satisfied. By Theorem 4.14, the homeomorphism
$H_K^{\bullet }(\mathrm {pt},\Bbbk )$
is the polynomial ring in finitely many variables of the second degree, Conditions (I)–(VIII) are satisfied. By Theorem 4.14, the homeomorphism
![]() $\theta $
is an isomorphism and it reads as the classical
$\theta $
is an isomorphism and it reads as the classical
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodule isomorphism
${\mathcal R}$
-bimodule isomorphism
5 Bott–Samelson varieties
5.1 Computation of the equivariant cohomology
Let G be a semisimple compact Lie group. We use Abbreviation (4-4). Additionally, we assume that
![]() $2$
is invertible in
$2$
is invertible in
![]() $\Bbbk $
. For any reflection t, we have two rings
$\Bbbk $
. For any reflection t, we have two rings
where
![]() $G_t$
is the group defined in the last paragraph of Section 2.14.
$G_t$
is the group defined in the last paragraph of Section 2.14.
Lemma 5.1. For any reflection t, the quotient map
![]() $\pi _t:K\mathop {\backslash } E\to G_t\mathop {\backslash } E$
induces the isomorphism
$\pi _t:K\mathop {\backslash } E\to G_t\mathop {\backslash } E$
induces the isomorphism
![]() $\pi _t^*:{\mathcal R}(t)\stackrel \sim \to {\mathcal R}^t$
.
$\pi _t^*:{\mathcal R}(t)\stackrel \sim \to {\mathcal R}^t$
.
Proof. The result follows from the proof of [Reference Brion, Broer, Daigneault and SabidussiBr, Proposition 1] applied to the group
![]() $G_t$
, as its Weyl group consists of
$G_t$
, as its Weyl group consists of
![]() $2$
elements and
$2$
elements and
![]() $2$
is invertible in
$2$
is invertible in
![]() $\Bbbk $
.
$\Bbbk $
.
For a sequence of reflections
![]() $\underline {t}=(t_1,\ldots ,t_n)$
, we define the following space:
$\underline {t}=(t_1,\ldots ,t_n)$
, we define the following space:
called the Bott–Samelson variety for sequence
![]() $\underline {t}$
. The sequence
$\underline {t}$
. The sequence
![]() $\underline {t}$
can be empty, in which case
$\underline {t}$
can be empty, in which case
![]() $\mathrm {BS}(\underline {t})$
is just the singleton. In what follows, we use the natural identification
$\mathrm {BS}(\underline {t})$
is just the singleton. In what follows, we use the natural identification
![]() $\mathrm {pt}\,{}_K\!\times E\cong K\mathop {\backslash } E$
given by
$\mathrm {pt}\,{}_K\!\times E\cong K\mathop {\backslash } E$
given by
![]() $K(pt,e)=Ke$
.
$K(pt,e)=Ke$
.
The torus K acts on the left on
![]() $\mathrm {BS}(\underline {t})$
(via the first factor for nonempty
$\mathrm {BS}(\underline {t})$
(via the first factor for nonempty
![]() $\underline {t}$
). Therefore, we can consider the equivariant cohomology denoted as follows:
$\underline {t}$
). Therefore, we can consider the equivariant cohomology denoted as follows:
It is a left
![]() ${\mathcal R}$
-module with respect to the canonical action. However, there is the K-equivariant map
${\mathcal R}$
-module with respect to the canonical action. However, there is the K-equivariant map
given by ![]() . Therefore,
. Therefore,
![]() $H(\underline {t})$
is also a right
$H(\underline {t})$
is also a right
![]() ${\mathcal R}$
-module with the action twisted by
${\mathcal R}$
-module with the action twisted by
![]() $A_{\underline {t}}$
according to Section 3.1.
$A_{\underline {t}}$
according to Section 3.1.
We represent
![]() $H(\underline {t})$
graphically by
$H(\underline {t})$
graphically by

If we need to indicate the elements of
![]() $\underline {t}$
directly, then we use the following notation:
$\underline {t}$
directly, then we use the following notation:
We consider the following map
![]() $\varphi _{\underline {t}}:\mathrm {BS}(\underline {t})\,{}_K\!\times E\to (K\mathop {\backslash } E)^{n+1}$
:
$\varphi _{\underline {t}}:\mathrm {BS}(\underline {t})\,{}_K\!\times E\to (K\mathop {\backslash } E)^{n+1}$
:
This map can be constructed inductively with the help of the embedding
![]() $\varphi $
constructed in Section 3.4 as follows. If
$\varphi $
constructed in Section 3.4 as follows. If
![]() $\underline {t}=\underline {\varnothing }$
, then
$\underline {t}=\underline {\varnothing }$
, then
![]() $\varphi _{\underline {t}}$
is the identity map.
$\varphi _{\underline {t}}$
is the identity map.
Now suppose that
![]() $\underline {t}$
is not empty. Then we consider the following commutative diagram:
$\underline {t}$
is not empty. Then we consider the following commutative diagram:

Here
![]() $\underline {t}'=(t_1,\ldots ,t_{n-1})$
is the truncated sequence (Section 2.2) and the map
$\underline {t}'=(t_1,\ldots ,t_{n-1})$
is the truncated sequence (Section 2.2) and the map
![]() $\varphi $
is the map defined in Section 3.4 for the following set of data:
$\varphi $
is the map defined in Section 3.4 for the following set of data:
and
![]() $\alpha :X\to G$
given by
$\alpha :X\to G$
given by
![]() $[g_1:\cdots :g_{n-1}]\mapsto g_1\cdots g_{n-1}$
.
$[g_1:\cdots :g_{n-1}]\mapsto g_1\cdots g_{n-1}$
.
Lemma 5.2. The map
![]() $\varphi _{\underline {t}}$
is a topological embedding.
$\varphi _{\underline {t}}$
is a topological embedding.
Using this embedding, we can define the map
![]() $\Theta _{\underline {t}}$
as the following composition:
$\Theta _{\underline {t}}$
as the following composition:
where the first map is the cross product (Section 3.7). We consider the following commutative diagram:

where
![]() $\Theta $
is Map (3-18) corresponding to
$\Theta $
is Map (3-18) corresponding to
![]() $\varphi $
. If we additionally set
$\varphi $
. If we additionally set
![]() $M=K$
and define
$M=K$
and define
![]() $B:Y\to G/M$
by
$B:Y\to G/M$
by
![]() $B(pt)=1M$
, then we get by Corollary 3.14 that
$B(pt)=1M$
, then we get by Corollary 3.14 that
![]() $\Theta $
is a homomorphism of rings and
$\Theta $
is a homomorphism of rings and
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-modules. Note that (5-3) is commutative as (5-2) is so. Indeed,
${\mathcal R}$
-modules. Note that (5-3) is commutative as (5-2) is so. Indeed,
Hence, we need to prove that
Both equalities follow if we append
![]() $\mathop{\rm pr}\nolimits _i$
to the left at both sides of the commutativity condition
$\mathop{\rm pr}\nolimits _i$
to the left at both sides of the commutativity condition
![]() $(\varphi _{\underline {t}'}\times \mathrm {id})\varphi =\varphi _{\underline {t}}$
of (5-2). As
$(\varphi _{\underline {t}'}\times \mathrm {id})\varphi =\varphi _{\underline {t}}$
of (5-2). As
![]() $\Theta _{\underline {\varnothing }}=\mathrm {id}$
, we can use Diagram (5-3) to compute
$\Theta _{\underline {\varnothing }}=\mathrm {id}$
, we can use Diagram (5-3) to compute
![]() $\Theta _{\underline {t}}$
inductively. This computation and Corollary 3.14 prove that
$\Theta _{\underline {t}}$
inductively. This computation and Corollary 3.14 prove that
![]() $\Theta _{\underline {t}}$
is a homomorphism of rings and
$\Theta _{\underline {t}}$
is a homomorphism of rings and
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules.
${\mathcal R}$
-bimodules.
Let us consider now the tensor product:
where each
![]() ${\mathcal R}(t_i)$
acts on the left and on the right on
${\mathcal R}(t_i)$
acts on the left and on the right on
![]() ${\mathcal R}$
through
${\mathcal R}$
through
![]() $\pi _{t_i}$
, that is,
$\pi _{t_i}$
, that is,
![]() $bm\hspace{-1pt}=\hspace{-1pt}\pi _{t_i}^*(b)\hspace{-1pt}\cup\hspace{-1pt} m$
and
$bm\hspace{-1pt}=\hspace{-1pt}\pi _{t_i}^*(b)\hspace{-1pt}\cup\hspace{-1pt} m$
and
![]() $mb=m\cup \pi _{t_i}^*(b)$
for any
$mb=m\cup \pi _{t_i}^*(b)$
for any
![]() $m\in {\mathcal R}$
and
$m\in {\mathcal R}$
and
![]() $b\in {\mathcal R}(t_i)$
. In view of Lemma 5.1,
$b\in {\mathcal R}(t_i)$
. In view of Lemma 5.1,
We use the first equality when we apply the (iso)morphisms
![]() $\theta $
introduced in Section 3.7 and the second equality to perform algebraic computations.
$\theta $
introduced in Section 3.7 and the second equality to perform algebraic computations.
Theorem 5.3. The map
![]() $\Theta _{\underline {t}}$
factors through the natural projection
$\Theta _{\underline {t}}$
factors through the natural projection
![]() ${\mathcal R}^{\,{\otimes }_{\Bbbk }\, n+1}\to {\mathcal R}^{\otimes \underline {t}}$
to an isomorphism of rings and
${\mathcal R}^{\,{\otimes }_{\Bbbk }\, n+1}\to {\mathcal R}^{\otimes \underline {t}}$
to an isomorphism of rings and
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules
${\mathcal R}$
-bimodules
![]() $\theta _{\underline {t}}:{\mathcal R}^{\otimes \underline {t}}\stackrel \sim \to H(\underline {t})$
.
$\theta _{\underline {t}}:{\mathcal R}^{\otimes \underline {t}}\stackrel \sim \to H(\underline {t})$
.
Proof. Let us apply induction on the length of
![]() $\underline {t}$
. There is nothing to prove if
$\underline {t}$
. There is nothing to prove if
![]() $\underline {t}=\underline {\varnothing }$
, as
$\underline {t}=\underline {\varnothing }$
, as
![]() $\Theta _{\underline {\varnothing }}=\mathrm {id}$
. Now suppose that
$\Theta _{\underline {\varnothing }}=\mathrm {id}$
. Now suppose that
![]() $\underline {t}$
is not empty. We consider the following diagram:
$\underline {t}$
is not empty. We consider the following diagram:

where
![]() $\theta $
is the map corresponding to
$\theta $
is the map corresponding to
![]() $\Theta $
from (5-3), as described in Section 3.7, and the dashed arrow is defined so that the trapezoid containing it is commutative. We need to prove that the triangle containing this arrow is also commutative. This is however so, as all other triangles, both trapezoids as well as the outer perimeter are commutative. The last claim follows from the commutativity of (5-3) and the inductive definition of
$\Theta $
from (5-3), as described in Section 3.7, and the dashed arrow is defined so that the trapezoid containing it is commutative. We need to prove that the triangle containing this arrow is also commutative. This is however so, as all other triangles, both trapezoids as well as the outer perimeter are commutative. The last claim follows from the commutativity of (5-3) and the inductive definition of
![]() $\theta _{\underline {t}'}$
. Note that
$\theta _{\underline {t}'}$
. Note that
![]() $\theta $
is an isomorphism by Theorem 4.14, the validity of Condition (VIII) being guaranteed inductively by Lemma 2.3. Thus,
$\theta $
is an isomorphism by Theorem 4.14, the validity of Condition (VIII) being guaranteed inductively by Lemma 2.3. Thus,
![]() $\theta _{\underline {t}}$
is a composition of isomorphisms and therefore is an isomorphism itself.
$\theta _{\underline {t}}$
is a composition of isomorphisms and therefore is an isomorphism itself.
5.2 Concatenation
Our next aim is to prove that the isomorphisms
![]() $\theta _{\underline {t}}$
of Theorem 5.3 behave well under the concatenation of sequences. Let
$\theta _{\underline {t}}$
of Theorem 5.3 behave well under the concatenation of sequences. Let
![]() $\underline {t}=(t_1,\ldots ,t_n)$
and
$\underline {t}=(t_1,\ldots ,t_n)$
and
![]() $\underline {r}=(r_1,\ldots ,r_m)$
be sequences of reflections. As in Section 3.4, we consider the embedding
$\underline {r}=(r_1,\ldots ,r_m)$
be sequences of reflections. As in Section 3.4, we consider the embedding
for the following set of data:
and
![]() $\alpha :X\to G$
defined by
$\alpha :X\to G$
defined by
![]() $[g_1:\cdots :g_n]\mapsto g_1\cdots g_n$
. Here we use the representation
$[g_1:\cdots :g_n]\mapsto g_1\cdots g_n$
. Here we use the representation
thus assuming
Setting additionally
![]() $M=K$
and defining
$M=K$
and defining
![]() $B:Y\to G/M$
by
$B:Y\to G/M$
by ![]() , we get by Theorem 4.14 the following isomorphisms of rings and
, we get by Theorem 4.14 the following isomorphisms of rings and
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules:
${\mathcal R}$
-bimodules:
where
![]() $\theta _{\underline {t},\underline {r}}$
is induced by
$\theta _{\underline {t},\underline {r}}$
is induced by
![]() $\varphi _{\underline {t},\underline {r}}$
as in Section 3.7.
$\varphi _{\underline {t},\underline {r}}$
as in Section 3.7.
Theorem 5.4. There is the following commutative diagram:

where the isomorphism of the bottom arrow is given by
Proof. Following the upper path,
Following the lower path,
Comparing the right-hand sides, we see that we need to prove the formulas
which follow from elementary calculations.
The maps
![]() $\varphi _{\underline {t},\underline {r}}$
were introduced for the universal principal bundle E. In what follows, we also use their compact versions
$\varphi _{\underline {t},\underline {r}}$
were introduced for the universal principal bundle E. In what follows, we also use their compact versions
![]() $\varphi _{\underline {t},\underline {r}}^N$
(Remark 2.8).
$\varphi _{\underline {t},\underline {r}}^N$
(Remark 2.8).
5.3 Bott towers
We remember the main constructions of [Reference Grossberg and KarshonGK]. Let n be a nonnegative integer and
![]() $\underline {c}=\{c_{i,j}\}_{1\leqslant i<j\leqslant n}$
be a collection of integers. The Bott tower [Reference Grossberg and KarshonGK, Section 2.2] corresponding to
$\underline {c}=\{c_{i,j}\}_{1\leqslant i<j\leqslant n}$
be a collection of integers. The Bott tower [Reference Grossberg and KarshonGK, Section 2.2] corresponding to
![]() $\underline {c}$
is the quotient
$\underline {c}$
is the quotient
with respect to the following action of
![]() $({\mathbb C}^\times )^n$
:
$({\mathbb C}^\times )^n$
:
 $$ \begin{align} &(z_1,w_1,\ldots,z_n,w_n)(1,\ldots,1,a_i,1,\ldots,1)\nonumber\\ &\quad=(z_1,w_1,\ldots,z_{i-1},w_{i-1}, z_i a_i, w_i a_i,\ldots,z_j,w_j a_i^{c_{i,j}},\ldots), \end{align} $$
$$ \begin{align} &(z_1,w_1,\ldots,z_n,w_n)(1,\ldots,1,a_i,1,\ldots,1)\nonumber\\ &\quad=(z_1,w_1,\ldots,z_{i-1},w_{i-1}, z_i a_i, w_i a_i,\ldots,z_j,w_j a_i^{c_{i,j}},\ldots), \end{align} $$
where
![]() $a_i$
is at the ith place. Note that
$a_i$
is at the ith place. Note that
![]() $\mathrm {BT}(\underline {c})$
is a smooth complex manifold and thus is canonically oriented. We denote the orbit containing the sequence
$\mathrm {BT}(\underline {c})$
is a smooth complex manifold and thus is canonically oriented. We denote the orbit containing the sequence
![]() $(z_1,w_1,\ldots ,z_n,w_n)$
by
$(z_1,w_1,\ldots ,z_n,w_n)$
by
![]() $[z_1,w_1,\ldots ,z_n,w_n]$
. This notation agrees with the notation of points of Bott towers in [Reference Grossberg and KarshonGK] and with our notation of points of
$[z_1,w_1,\ldots ,z_n,w_n]$
. This notation agrees with the notation of points of Bott towers in [Reference Grossberg and KarshonGK] and with our notation of points of
![]() $P^1(\mathbb C)$
introduced in Section 2.4. In the following lemma, we denote by
$P^1(\mathbb C)$
introduced in Section 2.4. In the following lemma, we denote by
![]() $\langle \alpha _t,\alpha _r\rangle $
the integer such that
$\langle \alpha _t,\alpha _r\rangle $
the integer such that
![]() $t(\alpha _r)=\alpha _r-\langle \alpha _r,\alpha _t\rangle \alpha _t$
.
$t(\alpha _r)=\alpha _r-\langle \alpha _r,\alpha _t\rangle \alpha _t$
.
Lemma 5.5. Let
![]() $\underline {t}=(t_1,\ldots ,t_n)$
be a sequence of reflections. Let us define
$\underline {t}=(t_1,\ldots ,t_n)$
be a sequence of reflections. Let us define
![]() $c_{i,j}=\langle \alpha _{t_j},\alpha _{t_i}\rangle $
and
$c_{i,j}=\langle \alpha _{t_j},\alpha _{t_i}\rangle $
and
![]() $\underline {c}=\{c_{i,j}\}_{1\leqslant i<j\leqslant n}$
. Then the manifolds
$\underline {c}=\{c_{i,j}\}_{1\leqslant i<j\leqslant n}$
. Then the manifolds
![]() $\mathrm {BT}(\underline {c})$
and
$\mathrm {BT}(\underline {c})$
and
![]() $\mathrm {BS}(\underline {t})$
are homeomorphic.
$\mathrm {BS}(\underline {t})$
are homeomorphic.
Proof. Let us consider the three-dimensional sphere
![]() $S^3\hspace{-1pt}=\hspace{-1pt}\{(z,w)\hspace{-1pt}\in\hspace{-1pt} {\mathbb C}^2\mathbin{\rm |} |z|^2\hspace{-1pt}+\hspace{-1pt}|w|^2\hspace{-1pt}=\hspace{-1pt}1\}$
. We can construct the quotient
$S^3\hspace{-1pt}=\hspace{-1pt}\{(z,w)\hspace{-1pt}\in\hspace{-1pt} {\mathbb C}^2\mathbin{\rm |} |z|^2\hspace{-1pt}+\hspace{-1pt}|w|^2\hspace{-1pt}=\hspace{-1pt}1\}$
. We can construct the quotient
![]() $(S^3)^n/(S^1)^n$
with respect to the group action given by (5-5). Using the same notation for the orbits of this space as for the orbits of
$(S^3)^n/(S^1)^n$
with respect to the group action given by (5-5). Using the same notation for the orbits of this space as for the orbits of
![]() $\mathrm {BT}(\underline {c})$
, we define the homeomorphism
$\mathrm {BT}(\underline {c})$
, we define the homeomorphism
![]() $(S^3)^n/(S^1)^n\stackrel \sim \to \mathrm {BS}(\underline {t})$
as follows:
$(S^3)^n/(S^1)^n\stackrel \sim \to \mathrm {BS}(\underline {t})$
as follows:
 $$ \begin{align*} [z_1,w_1,\ldots,z_n,w_n]\mapsto\left.\left[\varphi_{\alpha_{t_1}}\!\!\left(\!\!\begin{array}{cc}z_1& -\bar w_1\\w_1&\bar z_1\end{array}\!\!\right):\cdots:\varphi_{\alpha_{t_n}}\!\!\left(\!\!\begin{array}{cc}z_n& -\bar w_n\\w_n&\bar z_n\end{array}\!\!\right)\right]\!\!\right]. \end{align*} $$
$$ \begin{align*} [z_1,w_1,\ldots,z_n,w_n]\mapsto\left.\left[\varphi_{\alpha_{t_1}}\!\!\left(\!\!\begin{array}{cc}z_1& -\bar w_1\\w_1&\bar z_1\end{array}\!\!\right):\cdots:\varphi_{\alpha_{t_n}}\!\!\left(\!\!\begin{array}{cc}z_n& -\bar w_n\\w_n&\bar z_n\end{array}\!\!\right)\right]\!\!\right]. \end{align*} $$
Here
![]() $\varphi _{\alpha _{t_i}}$
are the root homomorphisms as in Section 2.14. As the natural embedding
$\varphi _{\alpha _{t_i}}$
are the root homomorphisms as in Section 2.14. As the natural embedding
![]() $(S^3)^n\hookrightarrow ({\mathbb C}^2-\{(0,0)\})^n$
induces the homeomorphism
$(S^3)^n\hookrightarrow ({\mathbb C}^2-\{(0,0)\})^n$
induces the homeomorphism
![]() $(S^3)^n/(S^1)^n\stackrel \sim \to \mathrm {BT}(\underline {c})$
, refer to [Reference Grossberg and KarshonGK, Section 2.2], the required result follows.
$(S^3)^n/(S^1)^n\stackrel \sim \to \mathrm {BT}(\underline {c})$
, refer to [Reference Grossberg and KarshonGK, Section 2.2], the required result follows.
The homomorphism described above defines two things: an orientation on
![]() $\mathrm {BS}(\underline {t})$
and a left K-action on
$\mathrm {BS}(\underline {t})$
and a left K-action on
![]() $\mathrm {BT}(\underline {c})$
. This action is given by
$\mathrm {BT}(\underline {c})$
. This action is given by
Note that the coefficients
![]() $c_{i,j}$
are given by the same formula in [Reference Grossberg and KarshonGK, Section 3.7] in the case where all reflections are simple.
$c_{i,j}$
are given by the same formula in [Reference Grossberg and KarshonGK, Section 3.7] in the case where all reflections are simple.
In the special case of a sequence of length 1, we have
![]() $\mathrm {BS}(t)\cong \mathrm {BT}(\underline {\varnothing })=P^1(\mathbb C)$
. This isomorphism proves that
$\mathrm {BS}(t)\cong \mathrm {BT}(\underline {\varnothing })=P^1(\mathbb C)$
. This isomorphism proves that
where
![]() $\dot t$
is a lifting of t (Section 2.14). We assume here and in the following that
$\dot t$
is a lifting of t (Section 2.14). We assume here and in the following that
![]() $\dot t$
is chosen within
$\dot t$
is chosen within
![]() $G_t$
for any reflection t. Moreover, we assume that the neutral element of W is lifted to the neutral element of G. By Lemma 5.5,
$G_t$
for any reflection t. Moreover, we assume that the neutral element of W is lifted to the neutral element of G. By Lemma 5.5,
Thus, in accordance with the identification of Section 2.14, we compute the equivariant Euler class (that is, the first Chern class), following the guidelines of [Reference Anderson and FultonAF, Ch. 2, Section 3], as follows:
for N big enough.
5.4 Fixed points
Let
![]() $\underline {t}=(t_1,\ldots ,t_n)$
be a sequence of reflections. We denote by
$\underline {t}=(t_1,\ldots ,t_n)$
be a sequence of reflections. We denote by
![]() $\Gamma _{\underline {t}}$
the set of generalized combinatorial galleries whose elements are sequences
$\Gamma _{\underline {t}}$
the set of generalized combinatorial galleries whose elements are sequences
![]() $\underline {\gamma }=(\gamma _1,\ldots ,\gamma _n)$
, where
$\underline {\gamma }=(\gamma _1,\ldots ,\gamma _n)$
, where
![]() $\gamma _i=t_i$
or
$\gamma _i=t_i$
or
![]() $\gamma _i=1$
for each i.
$\gamma _i=1$
for each i.
Lemma 5.6. The set
![]() ${}^{K\!}\mathrm {BS}(\underline {t})$
consists of the points
${}^{K\!}\mathrm {BS}(\underline {t})$
consists of the points ![]() , where
, where
![]() $n=|\underline {t}|$
and
$n=|\underline {t}|$
and
![]() $\underline {\gamma }\in \Gamma _{\underline {t}}$
.
$\underline {\gamma }\in \Gamma _{\underline {t}}$
.
Proof. As K is normalized by W, the points ![]() are fixed by K. To prove the converse claim, let us apply induction on n. The case
are fixed by K. To prove the converse claim, let us apply induction on n. The case
![]() $n=0$
is obvious. Suppose that
$n=0$
is obvious. Suppose that
![]() $n>0$
. Then
$n>0$
. Then
![]() $t_n$
is the last element of
$t_n$
is the last element of
![]() $\underline {t}$
. We consider the truncated sequence
$\underline {t}$
. We consider the truncated sequence
![]() $\underline {t}'$
and two K-equivariant maps
$\underline {t}'$
and two K-equivariant maps
![]() $\mathop{\rm tr}\nolimits :\mathrm {BS}(\underline {t})\to \mathrm {BS}(\underline {t}')$
and
$\mathop{\rm tr}\nolimits :\mathrm {BS}(\underline {t})\to \mathrm {BS}(\underline {t}')$
and
![]() $\zeta :\mathrm {BS}(\underline {t})\to G/K$
given by
$\zeta :\mathrm {BS}(\underline {t})\to G/K$
given by
respectively. Let
![]() $a\in \!{}^{K\!}\mathrm {BS}(\underline {t})$
. Then
$a\in \!{}^{K\!}\mathrm {BS}(\underline {t})$
. Then
![]() $\mathop{\rm tr}\nolimits (a)\in \!{}^{K\!}\mathrm {BS}(\underline {t}')$
. By induction, we have a representation
$\mathop{\rm tr}\nolimits (a)\in \!{}^{K\!}\mathrm {BS}(\underline {t}')$
. By induction, we have a representation ![]() for some
for some
![]() $(\gamma _1,\ldots \gamma _{n-1})\in \Gamma _{\underline {t}'}$
and
$(\gamma _1,\ldots \gamma _{n-1})\in \Gamma _{\underline {t}'}$
and
![]() $x_n\in G_{t_n}$
. However,
$x_n\in G_{t_n}$
. However,
![]() $\zeta (a)\in \!{}^{K\!}(G/K)$
. Thus,
$\zeta (a)\in \!{}^{K\!}(G/K)$
. Thus,
for any
![]() $k\in K$
. Hence,
$k\in K$
. Hence,
![]() $k'x_nK=x_nK$
, where
$k'x_nK=x_nK$
, where
![]() $k'=(\dot \gamma _1\cdots \dot \gamma _{n-1})^{-1}k\dot \gamma _1\cdots \dot \gamma _{n-1}$
. As
$k'=(\dot \gamma _1\cdots \dot \gamma _{n-1})^{-1}k\dot \gamma _1\cdots \dot \gamma _{n-1}$
. As
![]() $k'$
is an arbitrary element of K, we get by (5-6) that
$k'$
is an arbitrary element of K, we get by (5-6) that
![]() $x_nK\in \!{}^{K\!}\mathrm {BS}(t_n)=\{1K,\dot t_nK\}$
. The result follows.
$x_nK\in \!{}^{K\!}\mathrm {BS}(t_n)=\{1K,\dot t_nK\}$
. The result follows.
We identify
![]() $\underline {\gamma }$
with the point
$\underline {\gamma }$
with the point ![]() of
of
![]() $\mathrm {BS}(\underline {t})$
. For each
$\mathrm {BS}(\underline {t})$
. For each
![]() $x\in W$
, we denote by
$x\in W$
, we denote by
![]() $\Gamma _{\underline {t},x}$
the subset of
$\Gamma _{\underline {t},x}$
the subset of
![]() $\Gamma _{\underline {t}}$
consisting of sequences
$\Gamma _{\underline {t}}$
consisting of sequences
![]() $\underline {\gamma }$
such that
$\underline {\gamma }$
such that
![]() $\gamma _1\cdots \gamma _n=x$
. According to Lemma 3.5, the embedding
$\gamma _1\cdots \gamma _n=x$
. According to Lemma 3.5, the embedding
![]() $i_{\underline {\gamma }}:\mathrm {pt}\hookrightarrow \mathrm {BS}(\underline {t})$
taking value
$i_{\underline {\gamma }}:\mathrm {pt}\hookrightarrow \mathrm {BS}(\underline {t})$
taking value
![]() $\underline {\gamma }\in \Gamma _{\underline {t},x}$
induces the homomorphism of
$\underline {\gamma }\in \Gamma _{\underline {t},x}$
induces the homomorphism of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules
${\mathcal R}$
-bimodules
Note that in this formula, the left actions are canonical, and the right actions on the domain and codomain are twisted by
![]() $A_{\underline {t}}$
and
$A_{\underline {t}}$
and
![]() $A_{\underline {t}}i_{\underline {\gamma }}$
, respectively (Lemma 3.5). The last map takes only the value
$A_{\underline {t}}i_{\underline {\gamma }}$
, respectively (Lemma 3.5). The last map takes only the value
![]() $xK$
. We can compute
$xK$
. We can compute
![]() $i_{\underline {\gamma }}^{\star }$
in coordinates.
$i_{\underline {\gamma }}^{\star }$
in coordinates.
Theorem 5.7. For each
![]() $\underline {\gamma }\in \Gamma _{\underline {t},x}$
, the composition
$\underline {\gamma }\in \Gamma _{\underline {t},x}$
, the composition
is given by
![]() $ a_1\otimes \cdots \otimes a_{n+1}\mapsto a_1\cup \gamma _1(a_2)\cup \cdots \cup \gamma _1\cdots \gamma _n(a_{n+1}) $
.
$ a_1\otimes \cdots \otimes a_{n+1}\mapsto a_1\cup \gamma _1(a_2)\cup \cdots \cup \gamma _1\cdots \gamma _n(a_{n+1}) $
.
Proof. Replacing
![]() $\theta _{\underline {t}}$
by
$\theta _{\underline {t}}$
by
![]() $\Theta _{\underline {t}}$
, it remains to compute the following composition:
$\Theta _{\underline {t}}$
, it remains to compute the following composition:
Following these maps,

It is easy to note that
![]() $\mathop{\rm pr}\nolimits _j\varphi _{\underline {t}}(i_{\underline {\gamma }}\times \mathrm {id})=K\mathop {\backslash }\rho _{(\gamma _1\cdots \gamma _{j-1})^{-1}}$
, where the last map is defined in Section 2.14. Therefore, by (2-8),
$\mathop{\rm pr}\nolimits _j\varphi _{\underline {t}}(i_{\underline {\gamma }}\times \mathrm {id})=K\mathop {\backslash }\rho _{(\gamma _1\cdots \gamma _{j-1})^{-1}}$
, where the last map is defined in Section 2.14. Therefore, by (2-8),
The result follows from this formula and (5-8).
Using the Mayer–Vietoris isomorphism, we obtain that the restriction to
![]() ${}^{K\!}\mathrm {BS}(\underline {t})$
is the following homomorphism of
${}^{K\!}\mathrm {BS}(\underline {t})$
is the following homomorphism of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules:
${\mathcal R}$
-bimodules:
 $$ \begin{align*} H^{\bullet}_K(\mathrm{BS}(\underline{t}),\Bbbk)\to H^{\bullet}_K({}^K\mathrm{BS}(\underline{t}),\Bbbk)\stackrel\sim\to\bigoplus_{x\in W}\bigoplus_{\gamma\in\Gamma_{\underline{t},x}}{\mathcal R}_x. \end{align*} $$
$$ \begin{align*} H^{\bullet}_K(\mathrm{BS}(\underline{t}),\Bbbk)\to H^{\bullet}_K({}^K\mathrm{BS}(\underline{t}),\Bbbk)\stackrel\sim\to\bigoplus_{x\in W}\bigoplus_{\gamma\in\Gamma_{\underline{t},x}}{\mathcal R}_x. \end{align*} $$
We do not claim that this homomorphism is a monomorphism. However, this is true under some restrictions on
![]() $\Bbbk $
(for example, if
$\Bbbk $
(for example, if
![]() $\Bbbk $
has no zero divisors, refer to [Reference ShchigolevS1, Corollary 2.5]), in which case we say that the localization theorem holds.
$\Bbbk $
has no zero divisors, refer to [Reference ShchigolevS1, Corollary 2.5]), in which case we say that the localization theorem holds.
6 Morphisms
6.1 The setup
In the notation of the rest of the paper, we replace any sequence
![]() $\underline {t}$
of length 1 by its unique element t. For example,
$\underline {t}$
of length 1 by its unique element t. For example,
![]() $\varphi _{\underline {r},t}=\varphi _{\underline {r},\underline {t}}$
,
$\varphi _{\underline {r},t}=\varphi _{\underline {r},\underline {t}}$
,
![]() $\theta _t=\theta _{\underline {t}}$
,
$\theta _t=\theta _{\underline {t}}$
,
![]() $\theta _{\underline {r},t}=\theta _{\underline {r},\underline {t}}$
,
$\theta _{\underline {r},t}=\theta _{\underline {r},\underline {t}}$
,
![]() ${\mathcal R}^{\otimes t}={\mathcal R}^{\otimes \underline {t}}$
, and so forth. We also no longer write the symbol of the cup product between elements of
${\mathcal R}^{\otimes t}={\mathcal R}^{\otimes \underline {t}}$
, and so forth. We also no longer write the symbol of the cup product between elements of
![]() ${\mathcal R}$
.
${\mathcal R}$
.
We draw morphisms between bimodules
![]() $H(\underline {t})$
as usual from bottom to top. The easiest case is represented by trivial morphisms, which are drawn as vertical lines in the colors corresponding to the colors of the reflections:
$H(\underline {t})$
as usual from bottom to top. The easiest case is represented by trivial morphisms, which are drawn as vertical lines in the colors corresponding to the colors of the reflections:

Recall that for any reflection, we denote by
![]() $\alpha _t$
the positive root such that
$\alpha _t$
the positive root such that
![]() $t=\omega _{\alpha _t}$
(Section 2.14) and set
$t=\omega _{\alpha _t}$
(Section 2.14) and set
The operator
![]() $\partial _t$
in the last formula is called the Demazure operator. We get
$\partial _t$
in the last formula is called the Demazure operator. We get
![]() $P_t(f)\in {\mathcal R}^t$
,
$P_t(f)\in {\mathcal R}^t$
,
![]() $\partial _t(f)\in {\mathcal R}^t$
, and
$\partial _t(f)\in {\mathcal R}^t$
, and
An element m of an
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodule M is called central if
${\mathcal R}$
-bimodule M is called central if
![]() $rm=mr$
for every
$rm=mr$
for every
![]() $r\in {\mathcal R}$
. For example, if we consider
$r\in {\mathcal R}$
. For example, if we consider
![]() ${\mathcal R}$
itself as
${\mathcal R}$
itself as
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodule, then all elements of
${\mathcal R}$
-bimodule, then all elements of
![]() ${\mathcal R}$
are central. However, for any reflection t, only the zero element of the standard bimodule
${\mathcal R}$
are central. However, for any reflection t, only the zero element of the standard bimodule
![]() ${\mathcal R}_t$
(Section 4.7) is central. Indeed,
${\mathcal R}_t$
(Section 4.7) is central. Indeed,
![]() $m\in {\mathcal R}_t$
is central if and only if
$m\in {\mathcal R}_t$
is central if and only if
![]() $rm=t(r)m$
for every
$rm=t(r)m$
for every
![]() $r\in {\mathcal R}$
. Substituting
$r\in {\mathcal R}$
. Substituting
![]() $r=\alpha _t$
, we get
$r=\alpha _t$
, we get
![]() $2\alpha _tm=0$
. As
$2\alpha _tm=0$
. As
![]() $2$
is invertible, we get
$2$
is invertible, we get
![]() $m=0$
. Obviously, central elements are mapped to central ones by bimodule homomorphisms.
$m=0$
. Obviously, central elements are mapped to central ones by bimodule homomorphisms.
We use the shift of grading (Section 2.3) so that all homomorphisms of bimodules we consider are grading preserving. For example, we get the following exact sequence [Reference Elias and WilliamsonEW, (3.4)]:
where
![]() $\lambda _t(r)=r({\alpha _t}/2)\otimes 1+r\otimes ({\alpha _t}/2)$
and
$\lambda _t(r)=r({\alpha _t}/2)\otimes 1+r\otimes ({\alpha _t}/2)$
and
![]() $\sigma _t(f\otimes g)=ft(g)$
. Therefore, any central element of
$\sigma _t(f\otimes g)=ft(g)$
. Therefore, any central element of
![]() ${\mathcal R}\otimes _{{\mathcal R}^t}{\mathcal R}$
is in the kernel of
${\mathcal R}\otimes _{{\mathcal R}^t}{\mathcal R}$
is in the kernel of
![]() $\sigma _t$
and thus in the image of
$\sigma _t$
and thus in the image of
![]() $\lambda _t$
. Note that in [Reference Elias and WilliamsonEW], t is supposed to be simple, but the above sequence is actually exact for any reflection t.
$\lambda _t$
. Note that in [Reference Elias and WilliamsonEW], t is supposed to be simple, but the above sequence is actually exact for any reflection t.
6.2 One-color morphisms
Let us fix a reflection t. Consider the natural embedding
![]() $\iota _t:\mathrm {pt}\to \mathrm {BS}(t)$
, which maps pt to
$\iota _t:\mathrm {pt}\to \mathrm {BS}(t)$
, which maps pt to ![]() . We get
. We get
![]() $\iota _t=i_{(1)}$
in the notation of Section 5.4. We get the equivariant pull-back
$\iota _t=i_{(1)}$
in the notation of Section 5.4. We get the equivariant pull-back
which we represent as the diagram

This map is a homomorphism of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules by Lemma 3.5. By Theorem 5.7, we get that the composition map
${\mathcal R}$
-bimodules by Lemma 3.5. By Theorem 5.7, we get that the composition map
is given by
Now we consider the negated equivariant push-forward
Recall that a number in round brackets means the degree shift (Section 2.3). We represent the above morphism as follows:

By Lemma 3.6, this map is a homomorphism of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules. We want to compute the composition
${\mathcal R}$
-bimodules. We want to compute the composition
It suffices to compute the image of
![]() $1$
. As the element
$1$
. As the element
![]() $-\theta _t^{-1}i_{t\star }(1)$
is central and of degree
$-\theta _t^{-1}i_{t\star }(1)$
is central and of degree
![]() $2$
, the short exact sequence (6-2) and the argument following it show that
$2$
, the short exact sequence (6-2) and the argument following it show that
for some
![]() $c\in \Bbbk $
. To compute this constant, first apply
$c\in \Bbbk $
. To compute this constant, first apply
![]() $-\theta _t$
and then
$-\theta _t$
and then
![]() $\iota _t^{\star }$
to the formula above. By (5-7), Theorem 5.7, and (6-3),
$\iota _t^{\star }$
to the formula above. By (5-7), Theorem 5.7, and (6-3),
Hence,
![]() $c=1/2$
. As all maps in (6-4) are homomorphisms of bimodules,
$c=1/2$
. As all maps in (6-4) are homomorphisms of bimodules,
Let
![]() $\mu _t:\mathrm {BS}(t,t)\to \mathrm {BS}(t)$
be the map given by
$\mu _t:\mathrm {BS}(t,t)\to \mathrm {BS}(t)$
be the map given by ![]() . Taking the pull-back, we get the map
. Taking the pull-back, we get the map
We draw this diagram as follows:

This map is a homomorphism of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules by Lemma 3.5. We are going to compute the composition
${\mathcal R}$
-bimodules by Lemma 3.5. We are going to compute the composition
Computing in degree zero,
where the central unit is the constant unit function on
![]() $\mathrm {BS}(t,t){}_K\times E$
and all other units are the constant unit function on
$\mathrm {BS}(t,t){}_K\times E$
and all other units are the constant unit function on
![]() $K\mathop {\backslash } E$
. These functions are considered as cohomology classes and pull-back to constant unit functions, which explains the computation above.
$K\mathop {\backslash } E$
. These functions are considered as cohomology classes and pull-back to constant unit functions, which explains the computation above.
As all maps in (6-5) are homomorphisms of bimodules,
As expected, the merge diagram

is defined to be the negated push-forward map
![]() $-\mu _{t\star }:H(t,t)\to H(t)(-2)$
. We postpone the computation of its coordinate form until we manage to extend diagram (−ι
t⋆
) horizontally.
$-\mu _{t\star }:H(t,t)\to H(t)(-2)$
. We postpone the computation of its coordinate form until we manage to extend diagram (−ι
t⋆
) horizontally.
6.3 Horizontal extensions
We apply the concatenation properties proved in Section 5.2. First, let us consider the planar diagram

for a reflection t and a sequence of reflections
![]() $\underline {r}=(r_1,\ldots ,r_n)$
(the black solid strings can be of any color). Let
$\underline {r}=(r_1,\ldots ,r_n)$
(the black solid strings can be of any color). Let
![]() $\underline {r}t=(r_1,\ldots ,r_n,t)$
be the concatenated sequence and
$\underline {r}t=(r_1,\ldots ,r_n,t)$
be the concatenated sequence and
![]() $\iota _{\underline {r},t}:\mathrm {BS}(\underline {r})\hookrightarrow \mathrm {BS}(\underline {r}t)$
be the embedding given by
$\iota _{\underline {r},t}:\mathrm {BS}(\underline {r})\hookrightarrow \mathrm {BS}(\underline {r}t)$
be the embedding given by ![]() . We claim that the diagram
. We claim that the diagram

is commutative. Indeed, for any
![]() $a\in H(\underline {r})$
and
$a\in H(\underline {r})$
and
![]() $b\in H(t)$
,
$b\in H(t)$
,
Comparing the results, we see that it suffices to prove the equalities
They follow directly from the identification rule in (5-4) for concatenation operators and the definition in (3-10).
Now let us extend ( ι ⋆ ṟ,t ) to the right

for another sequence of reflections
![]() $\underline {v}=(v_1,\ldots ,v_m)$
. Let
$\underline {v}=(v_1,\ldots ,v_m)$
. Let
![]() $\underline {r}t\underline {v}=(r_1,\ldots ,r_n,t,v_1,\ldots ,v_m)$
be the concatenated sequence and
$\underline {r}t\underline {v}=(r_1,\ldots ,r_n,t,v_1,\ldots ,v_m)$
be the concatenated sequence and
![]() $\iota _{\underline {r},t,\underline {v}}:\mathrm {BS}(\underline {rv})\hookrightarrow \mathrm {BS}(\underline {r}t\underline {v})$
be the map defined by
$\iota _{\underline {r},t,\underline {v}}:\mathrm {BS}(\underline {rv})\hookrightarrow \mathrm {BS}(\underline {r}t\underline {v})$
be the map defined by ![]() . We get the commutative diagram
. We get the commutative diagram

Inserting (6-3) to (6-6) and (6-8), we get the formula in coordinates
 $$ \begin{align*} &\theta_{\underline{rv}}^{-1}\iota_{\underline{r},t,\underline{v}}^{\star}\theta_{\underline{r}t\underline{v}}(a_1\otimes\cdots\otimes a_{n+1}\otimes b_1\otimes\cdots\otimes b_{m+1})\\&\quad=a_1\otimes\cdots\otimes a_n\otimes a_{n+1}b_1\otimes b_2\otimes\cdots\otimes b_{m+1}. \end{align*} $$
$$ \begin{align*} &\theta_{\underline{rv}}^{-1}\iota_{\underline{r},t,\underline{v}}^{\star}\theta_{\underline{r}t\underline{v}}(a_1\otimes\cdots\otimes a_{n+1}\otimes b_1\otimes\cdots\otimes b_{m+1})\\&\quad=a_1\otimes\cdots\otimes a_n\otimes a_{n+1}b_1\otimes b_2\otimes\cdots\otimes b_{m+1}. \end{align*} $$
Let us extend (−ι t⋆ ) horizontally. To this end, we first prove that the diagram

is commutative. It is more difficult, as it involves push-forward operators. For any
![]() $a\in H(\underline {r})$
and
$a\in H(\underline {r})$
and
![]() $b\in {\mathcal R}$
, we get by the first equality of (6-7) and the projection formula (Proposition 2.9),
$b\in {\mathcal R}$
, we get by the first equality of (6-7) and the projection formula (Proposition 2.9),
 $$ \begin{align} \iota_{\underline{r},t\star}\theta_{\underline{r},\underline{\varnothing}}(a\otimes b)&=\iota_{\underline{r},t\star}\varphi_{\underline{r},\underline{\varnothing}}^*(\mathop{\rm pr}\nolimits_1^*(a)\cup\mathop{\rm pr}\nolimits_2^*(b)) =\iota_{\underline{r},t\star}((\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},\underline{\varnothing}})^*(a)\cup (\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},\underline{\varnothing}})^*(b))\nonumber\\ &=\iota_{\underline{r},t\star}(\iota_{\underline{r},t}^{\star}(\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},t})^*(a)\cup (\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},\underline{\varnothing}})^*(b))\nonumber\\ &=(\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},t})^*(a)\cup\iota_{\underline{r},t\star}(\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},\underline{\varnothing}})^*(b). \end{align} $$
$$ \begin{align} \iota_{\underline{r},t\star}\theta_{\underline{r},\underline{\varnothing}}(a\otimes b)&=\iota_{\underline{r},t\star}\varphi_{\underline{r},\underline{\varnothing}}^*(\mathop{\rm pr}\nolimits_1^*(a)\cup\mathop{\rm pr}\nolimits_2^*(b)) =\iota_{\underline{r},t\star}((\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},\underline{\varnothing}})^*(a)\cup (\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},\underline{\varnothing}})^*(b))\nonumber\\ &=\iota_{\underline{r},t\star}(\iota_{\underline{r},t}^{\star}(\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},t})^*(a)\cup (\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},\underline{\varnothing}})^*(b))\nonumber\\ &=(\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},t})^*(a)\cup\iota_{\underline{r},t\star}(\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},\underline{\varnothing}})^*(b). \end{align} $$
Let us consider the compatibly oriented Cartesian square

By (2-4), we get
![]() $(\iota _{\underline {r},t}\,{\mathop{{}_{K}\times}}\, \mathrm {id})_*(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},\underline {\varnothing }}^N)^*=(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},t}^N)^*(\iota _t\,{\mathop{{}_{K}\times}}\, \mathrm {id})_*$
. Taking the limit
$(\iota _{\underline {r},t}\,{\mathop{{}_{K}\times}}\, \mathrm {id})_*(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},\underline {\varnothing }}^N)^*=(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},t}^N)^*(\iota _t\,{\mathop{{}_{K}\times}}\, \mathrm {id})_*$
. Taking the limit
![]() $N\to \infty $
, we get
$N\to \infty $
, we get
![]() $\iota _{\underline {r},t\star }(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},\underline {\varnothing }})^*=(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},t})^*\iota _{t\star }$
. Applying this substitution to the right-hand side of (6-10),
$\iota _{\underline {r},t\star }(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},\underline {\varnothing }})^*=(\mathop{\rm pr}\nolimits _2\varphi _{\underline {r},t})^*\iota _{t\star }$
. Applying this substitution to the right-hand side of (6-10),
 $$ \begin{align*} \iota_{\underline{r},t\star}\theta_{\underline{r},\underline{\varnothing}}(a\otimes b)&=(\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},t})^*(a)\cup(\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},t})^*\iota_{t\star}(b)\\ &=\varphi_{\underline{r},t}^*(\mathop{\rm pr}\nolimits_1^*(a)\cup\mathop{\rm pr}\nolimits_2^*\iota_{t\star}(b))=\theta_{\underline{r},t}(a\otimes \iota_{t\star}(b))=\theta_{\underline{r},t}(\mathrm{id}\otimes\iota_{t\star})(a\otimes b). \end{align*} $$
$$ \begin{align*} \iota_{\underline{r},t\star}\theta_{\underline{r},\underline{\varnothing}}(a\otimes b)&=(\mathop{\rm pr}\nolimits_1\varphi_{\underline{r},t})^*(a)\cup(\mathop{\rm pr}\nolimits_2\varphi_{\underline{r},t})^*\iota_{t\star}(b)\\ &=\varphi_{\underline{r},t}^*(\mathop{\rm pr}\nolimits_1^*(a)\cup\mathop{\rm pr}\nolimits_2^*\iota_{t\star}(b))=\theta_{\underline{r},t}(a\otimes \iota_{t\star}(b))=\theta_{\underline{r},t}(\mathrm{id}\otimes\iota_{t\star})(a\otimes b). \end{align*} $$
This equality proves the commutativity of (6-9). We prove similarly that the diagram

is commutative. Computing in coordinates,
 $$ \begin{align} &\theta_{\underline{r}t\underline{v}}^{-1}(-\iota_{\underline{r},t,\underline{v}\star})\theta_{\underline{rv}}(a_1\otimes\cdots\otimes a_n\otimes d\otimes b_2\otimes\cdots\otimes b_{m+1})\nonumber\\ &\quad=a_1\otimes\cdots\otimes a_n\otimes\bigg( d\frac{\alpha_t}2\otimes 1+d\otimes\frac{\alpha_t}2\bigg)\otimes b_2\otimes\cdots\otimes b_{m+1}. \end{align} $$
$$ \begin{align} &\theta_{\underline{r}t\underline{v}}^{-1}(-\iota_{\underline{r},t,\underline{v}\star})\theta_{\underline{rv}}(a_1\otimes\cdots\otimes a_n\otimes d\otimes b_2\otimes\cdots\otimes b_{m+1})\nonumber\\ &\quad=a_1\otimes\cdots\otimes a_n\otimes\bigg( d\frac{\alpha_t}2\otimes 1+d\otimes\frac{\alpha_t}2\bigg)\otimes b_2\otimes\cdots\otimes b_{m+1}. \end{align} $$
This morphism is represented by the diagram

The split map
![]() $\mu _t^{\star }$
can be extended horizontally in the same way. Consider the diagram
$\mu _t^{\star }$
can be extended horizontally in the same way. Consider the diagram

for sequences of reflections
![]() $\underline {r}=(r_1,\ldots ,r_n)$
and
$\underline {r}=(r_1,\ldots ,r_n)$
and
![]() $\underline {v}=(v_1,\ldots ,v_m)$
. Let
$\underline {v}=(v_1,\ldots ,v_m)$
. Let
![]() $\mu _{\underline {r},t,\underline {v}}:\mathrm {BS}(\underline {r}tt\underline {v})\to \mathrm {BS}(\underline {r}t\underline {v})$
be the map given by
$\mu _{\underline {r},t,\underline {v}}:\mathrm {BS}(\underline {r}tt\underline {v})\to \mathrm {BS}(\underline {r}t\underline {v})$
be the map given by
where
![]() $\underline {r}tt\underline {v}=(r_1,\ldots ,r_n,t,t,v_1,\ldots ,v_m)$
. Arguing as for
$\underline {r}tt\underline {v}=(r_1,\ldots ,r_n,t,t,v_1,\ldots ,v_m)$
. Arguing as for
![]() $\iota _{\underline {r},t,\underline {v}}^{\star }$
,
$\iota _{\underline {r},t,\underline {v}}^{\star }$
,
At this point, we can compute the coordinate form of the merge diagram (−μ
t⋆
). We clearly have
![]() $\mu _t\iota _{t,t,\underline {\varnothing }}=\mathrm {id}$
. Taking the push-forwards, we get
$\mu _t\iota _{t,t,\underline {\varnothing }}=\mathrm {id}$
. Taking the push-forwards, we get
![]() $(-\mu _{t\star })(-\iota _{t,t,\underline {\varnothing }\star })=\mathrm {id}$
. Expressed diagrammatically, we get our first relation:
$(-\mu _{t\star })(-\iota _{t,t,\underline {\varnothing }\star })=\mathrm {id}$
. Expressed diagrammatically, we get our first relation:

Note that we have proved this relation without resorting to coordinatization. By (6-11),
 $$ \begin{align*} 1\otimes1=\theta_t^{-1}\mu_{t\star}\iota_{t,t,\varnothing\star}\theta_t(1\otimes 1)&=\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}\bigg(1\otimes\frac{\alpha_t}2\otimes 1+1\otimes 1\otimes\frac{\alpha_t}2\bigg)\\ &=\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}\bigg(1\otimes\frac{\alpha_t}2\otimes 1\bigg)-\theta_t^{-1}\mu_{t\star}\theta_{(t,t)}(1\otimes 1\otimes 1)\frac{\alpha_t}2. \end{align*} $$
$$ \begin{align*} 1\otimes1=\theta_t^{-1}\mu_{t\star}\iota_{t,t,\varnothing\star}\theta_t(1\otimes 1)&=\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}\bigg(1\otimes\frac{\alpha_t}2\otimes 1+1\otimes 1\otimes\frac{\alpha_t}2\bigg)\\ &=\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}\bigg(1\otimes\frac{\alpha_t}2\otimes 1\bigg)-\theta_t^{-1}\mu_{t\star}\theta_{(t,t)}(1\otimes 1\otimes 1)\frac{\alpha_t}2. \end{align*} $$
As
![]() $\theta _t^{-1}\mu _{t\star }\theta _{(t,t)}(1\otimes 1\otimes 1)=0$
for the degree reason,
$\theta _t^{-1}\mu _{t\star }\theta _{(t,t)}(1\otimes 1\otimes 1)=0$
for the degree reason,
Let us take any
![]() $b\in H(t)$
. Applying (6-1) and the above formula,
$b\in H(t)$
. Applying (6-1) and the above formula,
 $$ \begin{align*} &\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}(1\otimes b\otimes 1)\\ &\quad=-P_t(b)\,\theta_t^{-1}\mu_{t\star}\theta_{(t,t)}(1\otimes 1\otimes 1) +\partial_t(b)\,\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}\bigg(1\otimes\frac{\alpha_t}2\otimes 1\bigg) =\partial_t(b)\otimes 1. \end{align*} $$
$$ \begin{align*} &\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}(1\otimes b\otimes 1)\\ &\quad=-P_t(b)\,\theta_t^{-1}\mu_{t\star}\theta_{(t,t)}(1\otimes 1\otimes 1) +\partial_t(b)\,\theta_t^{-1}(-\mu_{t\star})\theta_{(t,t)}\bigg(1\otimes\frac{\alpha_t}2\otimes 1\bigg) =\partial_t(b)\otimes 1. \end{align*} $$
As this map is a homomorphism of bimodules,
The arguments described in this section allow us to extend
![]() $-\mu _{t\star }$
to the morphism
$-\mu _{t\star }$
to the morphism
![]() $-\mu _{\underline {r},t,\underline {v}\star }$
represented by the diagram
$-\mu _{\underline {r},t,\underline {v}\star }$
represented by the diagram

and prove the coordinate formula
 $$ \begin{align*} &\theta_{\underline{r}t\underline{v}}^{-1}(-\mu_{\underline{r},t,\underline{v}\star})\theta_{\underline{r}tt\underline{v}}(a_1\otimes\cdots\otimes a_n\otimes a\otimes b\otimes c\otimes b_2\otimes\cdots\otimes b_{m+1})\\ &\quad=a_1\otimes\cdots\otimes a_n\otimes a\partial_t(b)\otimes c\otimes b_2\otimes\cdots\otimes b_{m+1}. \end{align*} $$
$$ \begin{align*} &\theta_{\underline{r}t\underline{v}}^{-1}(-\mu_{\underline{r},t,\underline{v}\star})\theta_{\underline{r}tt\underline{v}}(a_1\otimes\cdots\otimes a_n\otimes a\otimes b\otimes c\otimes b_2\otimes\cdots\otimes b_{m+1})\\ &\quad=a_1\otimes\cdots\otimes a_n\otimes a\partial_t(b)\otimes c\otimes b_2\otimes\cdots\otimes b_{m+1}. \end{align*} $$
6.4 Generalized Bott–Samelson varieties
For any nonempty set S of simple reflections, let
![]() $G_S$
be the subgroup of G generated by all groups
$G_S$
be the subgroup of G generated by all groups
![]() $G_s$
, where
$G_s$
, where
![]() $s\in S$
. Let
$s\in S$
. Let
![]() $\underline {S}=(S_1,\ldots ,S_n)$
be a sequence of nonempty sets of simple reflections. Then we define
$\underline {S}=(S_1,\ldots ,S_n)$
be a sequence of nonempty sets of simple reflections. Then we define
Clearly, if each
![]() $S_i$
is a singleton, then
$S_i$
is a singleton, then
![]() $\mathrm {BS}(\underline {S})=\mathrm {BS}(\underline {s})$
, where
$\mathrm {BS}(\underline {S})=\mathrm {BS}(\underline {s})$
, where
![]() $S_i=\{s_i\}$
and
$S_i=\{s_i\}$
and
![]() $\underline {s}=(s_1,\ldots ,s_n)$
. Bearing in mind this fact, we omit brackets for singletons. For example, we abbreviate
$\underline {s}=(s_1,\ldots ,s_n)$
. Bearing in mind this fact, we omit brackets for singletons. For example, we abbreviate
![]() $\mathrm {BS}(\{s\},\{s',\tilde s\},\{\mathring s\},\{\ddot s\})$
to
$\mathrm {BS}(\{s\},\{s',\tilde s\},\{\mathring s\},\{\ddot s\})$
to
![]() $\mathrm {BS}(s,\{s',\tilde s\},{\mathring s},\ddot s)$
. We denote the equivariant cohomology by
$\mathrm {BS}(s,\{s',\tilde s\},{\mathring s},\ddot s)$
. We denote the equivariant cohomology by
![]() $H(\underline {S})=H_K^{\bullet }(\mathrm {BS}(\underline {S}),\Bbbk )$
, use the similar abbreviation, and draw
$H(\underline {S})=H_K^{\bullet }(\mathrm {BS}(\underline {S}),\Bbbk )$
, use the similar abbreviation, and draw
![]() $H(\underline {S})$
and the identical morphisms between them similarly to the cohomologies and morphisms for the usual Bott–Samelson varieties. For example, the identity map
$H(\underline {S})$
and the identical morphisms between them similarly to the cohomologies and morphisms for the usual Bott–Samelson varieties. For example, the identity map
![]() $H(s,\{s',\tilde s\},{\mathring s},\ddot s)\to H(s,\{s',\tilde s\},{\mathring s},\ddot s)$
is depicted as
$H(s,\{s',\tilde s\},{\mathring s},\ddot s)\to H(s,\{s',\tilde s\},{\mathring s},\ddot s)$
is depicted as

The variety
![]() $\mathrm {BS}(\underline {S})$
has a complex structure coming from the following algebraic realization:
$\mathrm {BS}(\underline {S})$
has a complex structure coming from the following algebraic realization:
where B and
![]() $P_{S_i}$
are the Borel subgroup and the minimal parabolic subgroup containing
$P_{S_i}$
are the Borel subgroup and the minimal parabolic subgroup containing
![]() $S_i$
of the complexification
$S_i$
of the complexification
![]() $G^c$
of G, respectively. In this way,
$G^c$
of G, respectively. In this way,
![]() $\mathrm {BS}(\underline {S})$
receives a complex structure and thus an orientation. We also get
$\mathrm {BS}(\underline {S})$
receives a complex structure and thus an orientation. We also get
![]() $G/K\cong G^c/B$
and thus the quotient
$G/K\cong G^c/B$
and thus the quotient
![]() $G/K$
also receives a complex structure. Also note that
$G/K$
also receives a complex structure. Also note that
![]() $H_B^{\bullet }(\mathrm {pt},\Bbbk )\cong H_T^{\bullet }(\mathrm {pt},\Bbbk )\cong H_K^{\bullet }(\mathrm {pt},\Bbbk )$
, where T is the maximal complex torus in
$H_B^{\bullet }(\mathrm {pt},\Bbbk )\cong H_T^{\bullet }(\mathrm {pt},\Bbbk )\cong H_K^{\bullet }(\mathrm {pt},\Bbbk )$
, where T is the maximal complex torus in
![]() $G^c$
containing K.
$G^c$
containing K.
In what follows, we consider only the case where each set
![]() $S_i$
is a singleton with at most one exception where this set consists of two distinct simple reflections (as in the example above).
$S_i$
is a singleton with at most one exception where this set consists of two distinct simple reflections (as in the example above).
6.5 Two-color morphisms
In the rest of the paper, we consider only sequences of simple reflections. For the simplicity of exposition, we omit the coordinatization maps
![]() $\theta _s$
, and thus think of them as identity maps. We denote
$\theta _s$
, and thus think of them as identity maps. We denote
for any simple reflection s.
If we consider
![]() $H(\underline {s})$
, where
$H(\underline {s})$
, where
![]() $\underline {s}=(s_1,\ldots ,s_n),$
as a left
$\underline {s}=(s_1,\ldots ,s_n),$
as a left
![]() ${\mathcal R}$
-module, then it has the following
${\mathcal R}$
-module, then it has the following
![]() ${\mathcal R}$
-basis:
${\mathcal R}$
-basis:
This
![]() ${\mathcal R}$
-basis consists of
${\mathcal R}$
-basis consists of
![]() $2^n$
elements. We denote by
$2^n$
elements. We denote by
![]() $H(\underline {s})^{<}$
the left
$H(\underline {s})^{<}$
the left
![]() ${\mathcal R}$
-submodule of
${\mathcal R}$
-submodule of
![]() $H(\underline {s})$
generated by all above basis elements such that
$H(\underline {s})$
generated by all above basis elements such that
![]() $\varepsilon _i=0$
for some i. The remaining basis element
$\varepsilon _i=0$
for some i. The remaining basis element
![]() $1\otimes x_{s_1}\otimes \cdots \otimes x_{s_n}$
is called the normal element of
$1\otimes x_{s_1}\otimes \cdots \otimes x_{s_n}$
is called the normal element of
![]() $H(\underline {s})$
; refer to [Reference LibedinskyLi, Définition 4.6].
$H(\underline {s})$
; refer to [Reference LibedinskyLi, Définition 4.6].
We leave the proof of the following result to the reader.
Proposition 6.1.
![]() $c_{s_1}\cdots c_{s_n}=1\otimes x_{s_1}\otimes \cdots \otimes x_{s_n}+h$
for some
$c_{s_1}\cdots c_{s_n}=1\otimes x_{s_1}\otimes \cdots \otimes x_{s_n}+h$
for some
![]() $h\in H(\underline {s})^{<}$
.
$h\in H(\underline {s})^{<}$
.
For any distinct simple reflections s and
![]() $\tilde s$
, we denote by
$\tilde s$
, we denote by
![]() $m_{s,\tilde s}$
the order of the product
$m_{s,\tilde s}$
the order of the product
![]() $s\tilde{s}$
. We have
$s\tilde{s}$
. We have
![]() $m_{s,\tilde s}=m_{\tilde s,s}\in \{2,3,4,6\}$
. We define the following sequence:
$m_{s,\tilde s}=m_{\tilde s,s}\in \{2,3,4,6\}$
. We define the following sequence:
 $$ \begin{align*} [s,\tilde s]=\left\{\!\! \begin{array}{ll} (s,\tilde s)&\text{ if }m_{s,\tilde s}=2,\\ (s,\tilde s,s)&\text{ if }m_{s,\tilde s}=3,\\ (s,\tilde s,s,\tilde s)&\text{ if }m_{s,\tilde s}=4,\\ (s,\tilde s,s,\tilde s,s,\tilde s)&\text{ if }m_{s,\tilde s}=6. \end{array} \right. \end{align*} $$
$$ \begin{align*} [s,\tilde s]=\left\{\!\! \begin{array}{ll} (s,\tilde s)&\text{ if }m_{s,\tilde s}=2,\\ (s,\tilde s,s)&\text{ if }m_{s,\tilde s}=3,\\ (s,\tilde s,s,\tilde s)&\text{ if }m_{s,\tilde s}=4,\\ (s,\tilde s,s,\tilde s,s,\tilde s)&\text{ if }m_{s,\tilde s}=6. \end{array} \right. \end{align*} $$
The product of the reflections of
![]() $[s,\tilde s]$
is denoted by
$[s,\tilde s]$
is denoted by
![]() $w_{s,\tilde s}$
. The braid relation for this pair reads as
$w_{s,\tilde s}$
. The braid relation for this pair reads as
![]() $w_{s,\tilde s}=w_{\tilde s,s}$
. We use the notation
$w_{s,\tilde s}=w_{\tilde s,s}$
. We use the notation
![]() $\mathrm {BS}(s,\tilde s,\ldots )=\mathrm {BS}([s,\tilde{s}])$
and
$\mathrm {BS}(s,\tilde s,\ldots )=\mathrm {BS}([s,\tilde{s}])$
and
![]() $H(s,\tilde{s},\ldots )= H([s,\tilde s])$
, and denote by
$H(s,\tilde{s},\ldots )= H([s,\tilde s])$
, and denote by
![]() $1\otimes x_s\otimes x_{\tilde{s}}\otimes \cdots $
the normal element of
$1\otimes x_s\otimes x_{\tilde{s}}\otimes \cdots $
the normal element of
![]() $H(s,\tilde s,\ldots )$
. Similarly,
$H(s,\tilde s,\ldots )$
. Similarly,
![]() $c_sc_{\tilde s}\cdots $
denotes one of the products
$c_sc_{\tilde s}\cdots $
denotes one of the products
![]() $c_sc_{\tilde s}$
,
$c_sc_{\tilde s}$
,
![]() $c_sc_{\tilde s}c_s$
,
$c_sc_{\tilde s}c_s$
,
![]() $c_sc_{\tilde s}c_sc_{\tilde s}$
,
$c_sc_{\tilde s}c_sc_{\tilde s}$
,
![]() $c_sc_{\tilde s}c_sc_{\tilde s}c_sc_{\tilde s}$
, depending on
$c_sc_{\tilde s}c_sc_{\tilde s}c_sc_{\tilde s}$
, depending on
![]() $m_{s,\tilde s}$
.
$m_{s,\tilde s}$
.
The image of the twisting map
![]() $A_{[s,\tilde s]}$
, refer to (5-1), is actually contained in the Schubert variety
$A_{[s,\tilde s]}$
, refer to (5-1), is actually contained in the Schubert variety
![]() $X_{s,\tilde s}=\overline {K_{w_{s,\tilde s}}/K}=\mathrm {BS}(\{s,\tilde s\})$
. Thus,
$X_{s,\tilde s}=\overline {K_{w_{s,\tilde s}}/K}=\mathrm {BS}(\{s,\tilde s\})$
. Thus,
![]() $A_{[s,\tilde s]}$
can be regarded as the map
$A_{[s,\tilde s]}$
can be regarded as the map
![]() $\eta _{s,\tilde s}:\mathrm {BS}(s,\tilde s,\ldots )\to X_{s,\tilde s}$
. The twisting maps for both sides are
$\eta _{s,\tilde s}:\mathrm {BS}(s,\tilde s,\ldots )\to X_{s,\tilde s}$
. The twisting maps for both sides are
![]() $A_{[s,\tilde s]}$
and the natural inclusion to
$A_{[s,\tilde s]}$
and the natural inclusion to
![]() $G/K$
, respectively. Note also that both these spaces are smooth manifolds having real dimension
$G/K$
, respectively. Note also that both these spaces are smooth manifolds having real dimension
![]() $2m_{s,\tilde s}$
. Therefore, we get the following two morphisms of
$2m_{s,\tilde s}$
. Therefore, we get the following two morphisms of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules:
${\mathcal R}$
-bimodules:
presented by the diagrams

Here the dash-dotted line can be either dotted or solid, depending on the parity of
![]() $m_{s,\tilde s}$
.
$m_{s,\tilde s}$
.
First, we calculate the following composition.
Lemma 6.2.
![]() $\eta _{s,\tilde s\star }\eta _{s,\tilde s}^{\star }=\mathrm {id}$
.
$\eta _{s,\tilde s\star }\eta _{s,\tilde s}^{\star }=\mathrm {id}$
.
Proof. Applying the projection formula (Proposition 2.9),
for any
![]() $h\in H_K^{\bullet }(X_{s,\tilde s},\Bbbk )$
. Therefore, it suffices to prove that
$h\in H_K^{\bullet }(X_{s,\tilde s},\Bbbk )$
. Therefore, it suffices to prove that
![]() $\eta _{s,\tilde s\star }(1)=1$
. There is an open subset
$\eta _{s,\tilde s\star }(1)=1$
. There is an open subset
![]() $V\subset X_{s,\tilde s}$
such that
$V\subset X_{s,\tilde s}$
such that
![]() $\eta _{s,\tilde s}$
induces the orientation-preserving diffeomorphism
$\eta _{s,\tilde s}$
induces the orientation-preserving diffeomorphism
![]() $\eta ^{\prime }_{s,\tilde s}:U\stackrel \sim \to V$
, where
$\eta ^{\prime }_{s,\tilde s}:U\stackrel \sim \to V$
, where
![]() $U=\eta _{s,\tilde s}^{-1}(V)$
. Consider the following Cartesian square:
$U=\eta _{s,\tilde s}^{-1}(V)$
. Consider the following Cartesian square:

By (the equivariant version of) (2-4),
As
![]() $X_{s,\tilde s}$
is connected, the above calculation proves that
$X_{s,\tilde s}$
is connected, the above calculation proves that
![]() $\eta _{s,\tilde s\star }(1)=1$
.
$\eta _{s,\tilde s\star }(1)=1$
.
Diagramatically expressed, this lemma looks as follows:

6.6 The
 $2m_{s,\tilde s}$
-valent vertex
$2m_{s,\tilde s}$
-valent vertex
Here, we are going to consider the composition
![]() $\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }:H(s,\tilde s,\ldots )\to H(\tilde s,s,\ldots )$
, which is a morphism of
$\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }:H(s,\tilde s,\ldots )\to H(\tilde s,s,\ldots )$
, which is a morphism of
![]() ${\mathcal R}$
-
${\mathcal R}$
-
![]() ${\mathcal R}$
-bimodules. It is depicted as follows:
${\mathcal R}$
-bimodules. It is depicted as follows:

Note that all such morphisms are proportional by [Reference LibedinskyLi, Proposition 4.3]. In this paper,
![]() $2$
was assumed to be invertible in
$2$
was assumed to be invertible in
![]() $\Bbbk $
.
$\Bbbk $
.
Lemma 6.3.
![]() $\ker \mu _{s,\tilde s\star }\subset H(s,\tilde s,\ldots )^{<}$
.
$\ker \mu _{s,\tilde s\star }\subset H(s,\tilde s,\ldots )^{<}$
.
Proof. Let
![]() $h\in \ker \mu _{s,\tilde s\star }$
. We have
$h\in \ker \mu _{s,\tilde s\star }$
. We have
![]() $h=\alpha \otimes x_s\otimes x_{\tilde s}\otimes \cdots +h'$
for some
$h=\alpha \otimes x_s\otimes x_{\tilde s}\otimes \cdots +h'$
for some
![]() $h'\in H(s,\tilde s,\ldots )^{<}$
. For reasons of degree, we get
$h'\in H(s,\tilde s,\ldots )^{<}$
. For reasons of degree, we get
![]() $\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }(h')\in H(\tilde s,s,\ldots )^{<}$
. Therefore, it follows from
$\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }(h')\in H(\tilde s,s,\ldots )^{<}$
. Therefore, it follows from
![]() $\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }(h)=0$
that
$\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }(h)=0$
that
By [Reference LibedinskyLi, Proposition 4.3 and Lemme 4.7], this is only possible if
![]() $\alpha =0$
.
$\alpha =0$
.
Our next aim is to prove the following normalization condition.
Lemma 6.4.
![]() $\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }(1\otimes x_s\otimes x_{\tilde s}\otimes \cdots )\hspace{-1.5pt}=\hspace{-1.5pt}1\otimes x_{\tilde s}\otimes x_s\otimes \cdots\hspace{-1pt} +\hspace{-1pt}h$
for some
$\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }(1\otimes x_s\otimes x_{\tilde s}\otimes \cdots )\hspace{-1.5pt}=\hspace{-1.5pt}1\otimes x_{\tilde s}\otimes x_s\otimes \cdots\hspace{-1pt} +\hspace{-1pt}h$
for some
![]() $h\hspace{-1pt}\in\hspace{-1pt} H (\tilde s,s,\ldots )^{<}$
.
$h\hspace{-1pt}\in\hspace{-1pt} H (\tilde s,s,\ldots )^{<}$
.
Proof. Let us consider the following chain of inclusions:
where
![]() $s'=s$
or
$s'=s$
or
![]() $s'=\tilde s$
depending on the parity of
$s'=\tilde s$
depending on the parity of
![]() $m_{s,\tilde s}$
. We denote the resulting composition by
$m_{s,\tilde s}$
. We denote the resulting composition by
![]() $\iota $
. Its image is
$\iota $
. Its image is ![]() . Taking the equivariant push-forward
. Taking the equivariant push-forward

we get by (6-11) that the image of
![]() $(-1)^{m_{s,\tilde s}}$
is equal to
$(-1)^{m_{s,\tilde s}}$
is equal to
![]() $c_sc_{\tilde s}\cdots $
. Hence,
$c_sc_{\tilde s}\cdots $
. Hence,
![]() $\eta _{s,\tilde s\star }(c_sc_{\tilde s}\cdots )=(\eta _{s,\tilde s}\iota )_\star ((-1)^{m_{s,\tilde s}})$
. Arguing similarly, we get
$\eta _{s,\tilde s\star }(c_sc_{\tilde s}\cdots )=(\eta _{s,\tilde s}\iota )_\star ((-1)^{m_{s,\tilde s}})$
. Arguing similarly, we get
![]() $\eta _{\tilde s,s\star }(c_{\tilde s}c_s\cdots )=(\eta _{\tilde s,s}\iota )_\star ((-1)^{m_{s,\tilde s}})$
. As
$\eta _{\tilde s,s\star }(c_{\tilde s}c_s\cdots )=(\eta _{\tilde s,s}\iota )_\star ((-1)^{m_{s,\tilde s}})$
. As
![]() $\eta _{s,\tilde s}\iota =\eta _{\tilde s,s}\iota $
, we get by Lemmas 6.2 and 6.3 that
$\eta _{s,\tilde s}\iota =\eta _{\tilde s,s}\iota $
, we get by Lemmas 6.2 and 6.3 that
To conclude the proof, it suffices to apply Proposition 6.1 and the fact that
![]() $\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }$
, being a degree-preserving homomorphism
$\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }$
, being a degree-preserving homomorphism
![]() $H(s,\tilde s,\ldots )\to H(\tilde s,s,\ldots )$
of left
$H(s,\tilde s,\ldots )\to H(\tilde s,s,\ldots )$
of left
![]() ${\mathcal R}$
-modules, maps
${\mathcal R}$
-modules, maps
![]() $H(s,\tilde s,\ldots )^{<}$
to
$H(s,\tilde s,\ldots )^{<}$
to
![]() $H(\tilde s,s,\ldots )^{<}$
.
$H(\tilde s,s,\ldots )^{<}$
.
We have just proved that the composition
![]() $\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }$
is just the map
$\eta _{\tilde s,s}^{\star }\eta _{s,\tilde s\star }$
is just the map
![]() $f_{s,\tilde s}$
from [Reference LibedinskyLi, Lemme 4.7].
$f_{s,\tilde s}$
from [Reference LibedinskyLi, Lemme 4.7].
6.7 The Jones–Wenzl projector
This map is given by the composition
![]() $\eta _{s,\tilde s}^{\star }\eta _{s,\tilde s\star }$
and is represented by the diagram:
$\eta _{s,\tilde s}^{\star }\eta _{s,\tilde s\star }$
and is represented by the diagram:

From Lemma 6.2, we immediately get the relations:


6.8 Two-color dot contraction
As an example for
![]() $m_{s,\tilde s}=3$
, let us prove the following relation:
$m_{s,\tilde s}=3$
, let us prove the following relation:

Indeed, the left-hand side and the right-hand side are equal to
respectively. Hence, it suffices to prove that
![]() $\eta _{\tilde s,s}\iota _{(\tilde s,s),\tilde s,\underline {\varnothing }}=\eta _{s,\tilde s}\iota _{\underline {\varnothing },s,(\tilde s,s)}$
, which is obvious.
$\eta _{\tilde s,s}\iota _{(\tilde s,s),\tilde s,\underline {\varnothing }}=\eta _{s,\tilde s}\iota _{\underline {\varnothing },s,(\tilde s,s)}$
, which is obvious.
6.9 Horizontal extensions of two-color morphisms
Finally, it remains to extend the morphisms
![]() $\eta _{s,\tilde s}^{\star }$
and
$\eta _{s,\tilde s}^{\star }$
and
![]() $\eta _{s,\tilde s\star }$
horizontally. It can be done similarly to the method used in Section 6.3. First, let
$\eta _{s,\tilde s\star }$
horizontally. It can be done similarly to the method used in Section 6.3. First, let
![]() $\underline {\mathring {s}}=(\mathring {s}_1,\ldots ,\mathring {s}_n)$
be a sequence of simple reflections. Then we define the map
$\underline {\mathring {s}}=(\mathring {s}_1,\ldots ,\mathring {s}_n)$
be a sequence of simple reflections. Then we define the map
by ![]() . We have the following commutative diagram:
. We have the following commutative diagram:

where
![]() $\theta _{\underline {\mathring {s}},s,\tilde s}$
corresponds to
$\theta _{\underline {\mathring {s}},s,\tilde s}$
corresponds to
as in Section 3.4 for the following set of data:
and
![]() $\alpha :X\to G$
defined by
$\alpha :X\to G$
defined by
![]() $[g_1:\cdots :g_n]\mapsto g_1\cdots g_n$
.
$[g_1:\cdots :g_n]\mapsto g_1\cdots g_n$
.
Now let
![]() $\underline {\ddot s}=(\ddot s_1,\ldots ,\ddot s_m)$
be another sequence of simple reflections. We define the map
$\underline {\ddot s}=(\ddot s_1,\ldots ,\ddot s_m)$
be another sequence of simple reflections. We define the map
by
We have the following commutative diagram:

where
![]() $\theta _{\underline {\mathring s},s,\tilde s,\underline {\ddot s}}$
is defined similarly to
$\theta _{\underline {\mathring s},s,\tilde s,\underline {\ddot s}}$
is defined similarly to
![]() $\theta _{\underline {\mathring s},s,\tilde s}$
. We leave it to the reader to write down similar diagrams for push-forwards, to draw the corresponding diagrams and to compose them (refer to the examples of diagrams in Section 1).
$\theta _{\underline {\mathring s},s,\tilde s}$
. We leave it to the reader to write down similar diagrams for push-forwards, to draw the corresponding diagrams and to compose them (refer to the examples of diagrams in Section 1).