No CrossRef data available.
Published online by Cambridge University Press: 06 April 2022
The ring
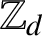 $\mathbb Z_{d}$
of d-adic integers has a natural interpretation as the boundary of a rooted d-ary tree
$\mathbb Z_{d}$
of d-adic integers has a natural interpretation as the boundary of a rooted d-ary tree
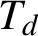 $T_{d}$
. Endomorphisms of this tree (that is, solenoidal maps) are in one-to-one correspondence with 1-Lipschitz mappings from
$T_{d}$
. Endomorphisms of this tree (that is, solenoidal maps) are in one-to-one correspondence with 1-Lipschitz mappings from
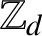 $\mathbb Z_{d}$
to itself. In the case when
$\mathbb Z_{d}$
to itself. In the case when
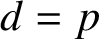 $d=p$
is prime, Anashin [‘Automata finiteness criterion in terms of van der Put series of automata functions’,p-Adic Numbers Ultrametric Anal. Appl. 4(2) (2012), 151–160] showed that
$d=p$
is prime, Anashin [‘Automata finiteness criterion in terms of van der Put series of automata functions’,p-Adic Numbers Ultrametric Anal. Appl. 4(2) (2012), 151–160] showed that
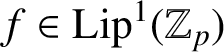 $f\in \mathrm {Lip}^{1}(\mathbb Z_{p})$
is defined by a finite Mealy automaton if and only if the reduced coefficients of its van der Put series constitute a p-automatic sequence over a finite subset of
$f\in \mathrm {Lip}^{1}(\mathbb Z_{p})$
is defined by a finite Mealy automaton if and only if the reduced coefficients of its van der Put series constitute a p-automatic sequence over a finite subset of
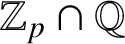 $\mathbb Z_{p}\cap \mathbb Q$
. We generalize this result to arbitrary integers
$\mathbb Z_{p}\cap \mathbb Q$
. We generalize this result to arbitrary integers
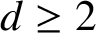 $d\geq 2$
and describe the explicit connection between the Moore automaton producing such a sequence and the Mealy automaton inducing the corresponding endomorphism of a rooted tree. We also produce two algorithms converting one automaton to the other and vice versa. As a demonstration, we apply our algorithms to the Thue–Morse sequence and to one of the generators of the lamplighter group acting on the binary rooted tree.
$d\geq 2$
and describe the explicit connection between the Moore automaton producing such a sequence and the Mealy automaton inducing the corresponding endomorphism of a rooted tree. We also produce two algorithms converting one automaton to the other and vice versa. As a demonstration, we apply our algorithms to the Thue–Morse sequence and to one of the generators of the lamplighter group acting on the binary rooted tree.
Communicated by Ben Martin
The first author graciously acknowledges support from the Simons Foundation through Collaboration Grant No. 527814 and is also supported by the mega-grant of the Russian Federation Government (N14.W03.31.0030). He also gratefully acknowledges support of the Swiss National Science Foundation.
The second author greatly appreciates the support of the Simons Foundation through Collaboration Grant No. 317198.