Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-24T05:35:19.151Z Has data issue: false hasContentIssue false
  • English
  • Français

Separation axioms and subcategories of top

Published online by Cambridge University Press:  09 April 2009

Ryosuke Nakagawa
Ryosuke Nakagawa
Affiliation:
Department of Mathematics, University of Tsukuba Ibaraki, Japan.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

(Point, closed subset)-separation axioms and closed subsets separation axioms for topological spaces will be uniformly defined. Then it is shown that a subcategory 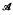of TOP is bireflective in TOP if and only if Ob 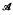consists of all separated spaces for some (point, closed subset)-separation axiom. A characterization theorem on subcategories of all separated spaces for closed subsets separation axioms is also given by using the category SEP of all separation spaces and the embedding functor G: TOP → SEP. As an application we have that a T1-space is normal if and only if it is embedded in a product space of the unit intervals in SEP.

Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 22 , Issue 4 , December 1976 , pp. 476 - 490
Copyright
Copyright © Australian Mathematical Society 1976

References

Császár, A. (1960), Fondements de la topologie general, (Budapest, Paris, 1960).Google Scholar
Davis, A. S. (1961), ‘Indexed systems of neighborhoods for general topological spaces’, Amer. Math. Monthly 68, 886–893.CrossRefGoogle Scholar
Hammer, P. C. (1963), ‘Extended topology: Connected sets and Wallace separations’, Portugaliae Math. 22, 167–187.Google Scholar
Herrlich, H. (1974), ‘Topological functors’, General Topology and Appl. 4, 125–142.Google Scholar
Herrlich, H. (1974a), ‘A concept of nearness’, General Topology and Appl. 4, 191–212.CrossRefGoogle Scholar
Herrlich, H. and Strecker, G. E. (1973), Category theory, (Allyn and Bacon, Boston, 1973).Google Scholar
Kannan, V. (1972), ‘Reflexive cum coreflexive subcategories’, Math. Ann. 195, 168–174.CrossRefGoogle Scholar
Pervin, W. J. (1963), ‘Quasi-proximities for topological spaces’, Math. Ann. 150, 325–326.CrossRefGoogle Scholar
Sharpe, R., Beattie, M. and Marsden, J. (1966), ‘A universal factorization theorem in topology’, Canad. Math. Bull. 9, 201–207.CrossRefGoogle Scholar
Terada, T. (1975), ‘Note on z-, C*-, and C-embedding’, Sci. Rep. Tokyo Kyoiku Daigaku 13, 129–132.Google Scholar
Thomas, J. P. (1968), ‘Associated regular spaces’, Canad. J. Math. 20, 1087–1092.CrossRefGoogle Scholar
Wallace, A. D. (1941), ‘Separation spaces’, Ann. Math. 42, 687–697.CrossRefGoogle Scholar
Wyler, O. (1973), ‘Quotient maps’, General Topology and Appl. 3, 149–160.CrossRefGoogle Scholar