Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Argabright, L. N.
1966.
A note on invariant integrals on locally compact semigroups.
Proceedings of the American Mathematical Society,
Vol. 17,
Issue. 2,
p.
377.
Tewari, U. B.
1968.
A note on right invariant integrals on locally compact semigroups.
Journal of the Australian Mathematical Society,
Vol. 8,
Issue. 3,
p.
512.
Tserpes, N. A.
and
Kartsatos, A. G.
1969.
On the equivalence of invariant integrals and minimal ideals in semigroups.
Bulletin of the Australian Mathematical Society,
Vol. 1,
Issue. 2,
p.
269.
Mukherjea, A.
1969.
Idempotent probabilities on semigroups.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 11,
Issue. 2,
p.
142.
Sun, Tze-Chien
and
Tserpes, Nicolas A.
1970.
Idempotent measures on locally compact semigroups.
Zeitschrift f�r Wahrscheinlichkeitstheorie und Verwandte Gebiete,
Vol. 15,
Issue. 4,
p.
273.
Tewari, U.B
1973.
Subinvariant integrals and means on topological semigroups.
Journal of Mathematical Analysis and Applications,
Vol. 41,
Issue. 2,
p.
328.
Cooper, T.J.
and
Michael, J.H.
1974.
Two fixed point theorems and invariant integrals.
Bulletin of the Australian Mathematical Society,
Vol. 11,
Issue. 1,
p.
15.
Chew, James
1975.
Left invariant measure in topological semigroups.
Journal of the Australian Mathematical Society,
Vol. 20,
Issue. 2,
p.
142.
Högnäs, Göran
and
Akademi, Åbo
1989.
Probability Measures on Groups IX.
Vol. 1379,
Issue. ,
p.
135.


 of continuous real-valued functions on ς with compact supports. If ς has the property: (A) for each pair of compact sets
of continuous real-valued functions on ς with compact supports. If ς has the property: (A) for each pair of compact sets 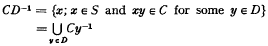
 and a ∈ S, the function
and a ∈ S, the function 

