Published online by Cambridge University Press: 03 May 2019
A classical result due to Paley and Wiener characterizes the existence of a nonzero function in 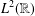 $L^{2}(\mathbb{R})$, supported on a half-line, in terms of the decay of its Fourier transform. In this paper, we prove an analogue of this result for Damek–Ricci spaces.
$L^{2}(\mathbb{R})$, supported on a half-line, in terms of the decay of its Fourier transform. In this paper, we prove an analogue of this result for Damek–Ricci spaces.
The author was supported by Research Fellowship from Indian Statistical Institute, India.
Current address: Department of Mathematics, Indian Institute of Technology, Bombay, Powai, Mumbai-400076, India email [email protected], [email protected]