Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-16T17:09:41.453Z Has data issue: false hasContentIssue false
  • English
  • Français

Representations of minimally almost periodic groups

Part of: Linear algebraic groups and related topics Selfadjoint operator algebras Locally compact groups and their algebras

Published online by Cambridge University Press:  09 April 2009

Alain Valette
Alain Valette
Affiliation:
Department of Mathematics CP 216, Université Libre de Bruxelles, Boulevard du Triomphe, B-1050 Brussels, Belgium
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For any group G, we introduce the subset S(G) of elements g which are conjugate to 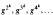for some positive integer k. We show that, for any bounded representation π of G any g in S(G), either π(g) = 1 or the spectrum of π(g) is the full unit circle in C. As a corollary, S(G) is in the kernel of any homomorphism from G to the unitary group of a post-liminal C*-algebra with finite composition series.

Next, for a topological group G, we consider the subset of elements approximately conjugate to 1, and we prove that it is contained in the kernel of any uniformly continuous bounded representation of G, and of any strongly continuous unitary representation in a finite von Neumann algebra.

We apply these results to prove triviality for a number of representations of isotropic simple algebraic groups defined over various fields.

MSC classification

Secondary: 22D12: Other representations of locally compact groups 20G15: Linear algebraic groups over arbitrary fields 46L05: General theory of $C^*$-algebras
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 41 , Issue 3 , December 1986 , pp. 366 - 375
Copyright
Copyright © Australian Mathematical Society 1986

References

[1]Borel, A. et Tits, J., ‘Homomorphismes “abstraits” de groupes algébriques simples’, Ann. of Math. 98 (1973), 499–573.CrossRefGoogle Scholar
[2]Dieudonné, J., La géométrie des groupes classiques, 2nde édition (Springer-Verlag, Berlin and New York, 1963).CrossRefGoogle Scholar
[3]Gurarie, D., ‘Banach uniformly continuous representations of Lie groups and algebras’, J. Funct. Anal. 36 (1980), 401–407.CrossRefGoogle Scholar
[4]Gurarie, D., ‘Banach uniformly continuous representations of locally compact groups’, preprint, 1979.Google Scholar
[5]Howe, R. E. and Moore, C. C., ‘Asymptotic properties of unitary representations’, J. Funct. Anal. 32 (1979), 72–96.CrossRefGoogle Scholar
[6]Kallman, R. V., ‘A characterization of uniformly continuous unitary representations of connected locally compact groups’, Michigan Math. J. 16 (1969), 257–263.CrossRefGoogle Scholar
[7]Kuipers, L. and Niederreiter, H., Uniform distribution of sequences (Texts & Monographs in Pure & Applied Math., J. Wiley, New York, 1974).Google Scholar
[8]Mackey, G. W., The theory of unitary group representations (Chicago Lecture Notes in Math., Univ. of Chicago Press, 1976).Google Scholar
[9]Moore, C. C., ‘Restrictions of unitary representations to subgroups, and ergodic theory’ (Lecture Notes in Physics, 6, Springer-Verlag, Berlin and New York, 1970, pp. 1–36).Google Scholar
[10]von Neumann, J. and Segal, I. E., ‘A theorem on unitary representations of semi-simple Lie groups’, Ann. of Math. 52 (1950), 509–517.Google Scholar
[11]von Neumann, J. and Wigner, E. P., ‘Minimally almost periodic groups’, Ann. of Math. 41 (1940), 746–750.CrossRefGoogle Scholar
[12]Pedersen, G. K., C*-algebras and their automorphism groups (London Math. Soc. Monographs, 14, Academic Press, New York, 1979).Google Scholar
[13]Rothman, S., ‘The von Neumann kernel and minimally almost periodic groups’, Trans. Amer. Math. Soc. 259 (1980), 401–421.CrossRefGoogle Scholar
[14]Rothman, S. and Strassberg, H., ‘The von Neumann kernel of a locally compact group’, J. Austral. Math. Soc. Ser. A 36 (1984), 279–286.CrossRefGoogle Scholar
[15]Sherman, T., ‘Representations of Lie algebras by normal operators’, Proc. Amer. Math. Soc. 16 (1965), 1125–1129.CrossRefGoogle Scholar
[16]Singer, I. M., ‘Uniformly continuous representations of Lie groups’, Ann. of Math. 56 (1952), 242–247.CrossRefGoogle Scholar
[17]Tits, J., ‘Algebraic and abstract simple groups’, Ann. of Math. 80 (1964), 313–329.CrossRefGoogle Scholar
[18]van der Waerden, B. L., ‘Stetigkeitssätze für halbeinfache Liesche Gruppen’, Math. Z. 36 (1932), 780–786.CrossRefGoogle Scholar