Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-23T23:40:06.297Z Has data issue: false hasContentIssue false
  • English
  • Français

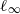REMARKS ON ℓ1 AND -MAXIMAL REGULARITY FOR POWER-BOUNDED OPERATORS

Part of: Difference equations General theory of linear operators Groups and semigroups of linear operators, their generalizations and applications Difference and functional equations

Published online by Cambridge University Press:  01 June 2008

N. J. KALTON  and
P. PORTAL
N. J. KALTON
Affiliation:
Department of Mathematics, University of Missouri–Columbia, Columbia, MO 65211, USA (email: [email protected])
P. PORTAL*
Affiliation:
Centre for Mathematics and its Applications, Australian National University, Canberra, ACT 0200, Australia (email: [email protected])
*
✉For correspondence; e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

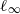We discuss ℓp-maximal regularity of power-bounded operators and relate the discrete to the continuous time problem for analytic semigroups. We give a complete characterization of operators with ℓ1 and -maximal regularity. We also introduce an unconditional form of Ritt’s condition for power-bounded operators, which plays the role of the existence of an -calculus, and give a complete characterization of this condition in the case of Banach spaces which are L1-spaces, C(K)-spaces or Hilbert spaces.

Keywords

power-bounded operatorsRitt’s conditionmaximal regularityH inftysquare function

MSC classification

Secondary: 47A30: Norms (inequalities, more than one norm, etc.) 47A60: Functional calculus 47D06: One-parameter semigroups and linear evolution equations 39A12: Discrete version of topics in analysis
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 84 , Issue 3 , June 2008 , pp. 345 - 365
Copyright
Copyright © 2008 Australian Mathematical Society

Footnotes

The first author acknowledges support from NSF grant DMS-0244515.

References

[1]Auscher, P., McIntosh, A. and Nahmod, A., ‘Holomorphic functional calculi of operators, quadratic estimates and interpolation’, Indiana Univ. Math. J. 46(2) (1997), 375–403.Google Scholar
[2]Baillon, J.-B., ‘Caractère borné de certains générateurs de semi-groupes linéaires dans les espaces de Banach’, C. R. Acad. Sci. Paris Sér. A 290(16) (1980), 757–760.Google Scholar
[3]Bessaga, C. and Pełczyński, A., ‘On bases and unconditional convergence of series in Banach spaces’, Studia Math. 17 (1958), 151–164.Google Scholar
[4]Blunck, S., ‘Maximal regularity of discrete and continuous time evolution equations’, Studia Math. 146(2) (2001), 157–176.Google Scholar
[5]Blunck, S., ‘Analyticity and discrete maximal regularity on L p-spaces’, J. Funct. Anal. 183(1) (2001), 211–230.Google Scholar
[6]Cowling, M., Doust, I., McIntosh, A. and Yagi, A., ‘Banach space operators with a bounded functional calculus’, J. Austral. Math. Soc. (Series A) 60(1) (1996), 51–89.CrossRefGoogle Scholar
[7]Da Prato, G. and Grisvard, P., ‘Equations d’évolution abstraites non linéaires de type parabolique’, Ann. Mat. Pura Appl. (4) 120 (1979), 329–396.Google Scholar
[8]Dore, G., ‘Maximal regularity in L p spaces for an abstract Cauchy problem’, Adv. Differential Equations 5(1–3) (2000), 293–322.CrossRefGoogle Scholar
[9]Guerre-Delabrière, S., ‘L p-regularity of the Cauchy problem and the geometry of Banach spaces’, Illinois J. Math. 39(4) (1995), 556–566.Google Scholar
[10]Kalton, N. J., ‘A remark on sectorial operators with an -calculus’, in: Trends in Banach Spaces and Operator Theory (Memphis, TN, 2001), Contemporary Mathematics, 321 (American Mathematical Society, Providence, RI, 2003), pp. 91–99.Google Scholar
[11]Kalton, N. J. and Weis, L., ‘The -calculus and sums of closed operators’, Math. Ann. 321(2) (2001), 319–345.Google Scholar
[12]Lunardi, A., Analytic Semigroups and Optimal Regularity in Parabolic Problems, Progress in Nonlinear Differential Equations and Their Applications, 16 (Birkhäuser, Basel, 1995).Google Scholar
[13]Lyubich, Yu., ‘Spectral localization, power boundedness and invariant subspaces under Ritt’s type condition’, Studia Math. 134(2) (1999), 153–167.CrossRefGoogle Scholar
[14]McIntosh, A., ‘Operators which have an functional calculus’, in: Miniconference on Operator Theory and Partial Differential Equations (North Ryde, 1986), Proceedings of the Centre for Mathematical Analysis of the Australian National University, 14 (Australian National University, Canberra, 1986), pp. 210–231.Google Scholar
[15]Nagy, B. and Zemánek, J., ‘A resolvent condition implying power boundedness’, Studia Math. 134(2) (1999), 143–151.CrossRefGoogle Scholar
[16]Pisier, G., Factorization of Linear Operators and Geometry of Banach Spaces, CBMS Regional Conference Series in Mathematics, 60 (Conference Board of the Mathematical Sciences, Washington, DC, 1986).Google Scholar
[17]Portal, P., ‘Discrete time analytic semigroups and the geometry of Banach spaces’, Semigroup Forum 67(1) (2003), 125–144.CrossRefGoogle Scholar
[18]Ritt, R. K., ‘A condition that ’, Proc. Amer. Math. Soc. 4 (1953), 898–899.Google Scholar