Published online by Cambridge University Press: 09 March 2020
We consider the fractional critical problem  $A_{s}u=K(x)u^{(n+2s)/(n-2s)},u>0$ in
$A_{s}u=K(x)u^{(n+2s)/(n-2s)},u>0$ in 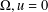 $\unicode[STIX]{x1D6FA},u=0$ on
$\unicode[STIX]{x1D6FA},u=0$ on 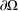 $\unicode[STIX]{x2202}\unicode[STIX]{x1D6FA}$, where
$\unicode[STIX]{x2202}\unicode[STIX]{x1D6FA}$, where  $A_{s},s\in (0,1)$, is the fractional Laplace operator and
$A_{s},s\in (0,1)$, is the fractional Laplace operator and 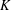 $K$ is a given function on a bounded domain
$K$ is a given function on a bounded domain  $\unicode[STIX]{x1D6FA}$ of
$\unicode[STIX]{x1D6FA}$ of 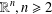 $\mathbb{R}^{n},n\geq 2$. This is based on A. Bahri’s theory of critical points at infinity in Bahri [Critical Points at Infinity in Some Variational Problems, Pitman Research Notes in Mathematics Series, 182 (Longman Scientific & Technical, Harlow, 1989)]. We prove Bahri’s estimates in the fractional setting and we provide existence theorems for the problem when
$\mathbb{R}^{n},n\geq 2$. This is based on A. Bahri’s theory of critical points at infinity in Bahri [Critical Points at Infinity in Some Variational Problems, Pitman Research Notes in Mathematics Series, 182 (Longman Scientific & Technical, Harlow, 1989)]. We prove Bahri’s estimates in the fractional setting and we provide existence theorems for the problem when 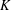 $K$ is close to 1.
$K$ is close to 1.
Communicated by F. Cirstea
This project was funded by the Deanship of Scientific Research (DSR), King Abdulaziz University, Jeddah, Saudi Arabia under Grant No. (KEP-PhD-41-130-38). The authors, therefore, acknowledge with thanks DSR technical and financial support.