Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-09T09:26:13.253Z Has data issue: false hasContentIssue false
  • English
  • Français

Perspective simplexes †

Published online by Cambridge University Press:  09 April 2009

Sahib Ram Mandan
Sahib Ram Mandan
Affiliation:
Indian Institute of TechnologyKharagpur, India
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The main purpose of this paper is to prove the proposition: “A set of r mutually perspective (m.p.) (s—1)-simplexes have the same [s−2] (say x) of perspectivity, if and only if their 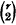centres of perspectivity (c.p.) lie in an [r−2] (say y); there then arises another such set of s m.p. (r—1)-simplexes, having the same rs vertices, which have y as their common [r−2] of perspectivity such that their 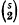c.p. lie in x.” The proposition is true in any [k] for k = s−1, s,…, r+s−2 (r ≦ s). The configuration of the proposition in [r+s−2] arises from the incidences of any r+s arbitrary primes therein and is therefore invariant under the symmetric group of permutations of r+s objects, and that in [r+s−3] is self-dual and therefore self- polar for a quadric therein. Some special cases of some interest for r = s are deduced. The treatment is an illustration of the elegance of the Möbius Barycentric Calculus ([15], pp. 136–143; [1], p. 71).

Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 6 , Issue 1 , February 1966 , pp. 11 - 17
Copyright
Copyright © Australian Mathematical Society 1966

References

[1],[2],[3],[4]Baker, H. F., Principles of Geometry, vols. 1, 2, 3, 4 (Cambridge, 1929, 1930, 1934, 1940).Google Scholar
[5]Baker, H. F., Introduction to plane geometry (Cambridge, 1943).Google Scholar
[6]Court, N. A., Desargues and his strange theorem, Scripta Math. 20 (1954), 1–20.Google Scholar
[7]Coxeter, H. S. M., Twelve points in PG (5,3) with 95040 selftransformations, Proc. Roy. Soc. A 247 (1958), 279–293.Google Scholar
[8]Mandan, S. R., Commutative law in four dimensional space S 4. East Panjab. Uni. Res. Bull. 14 (1951), 31–32.Google Scholar
[9]Mandan, S. R., Projective tetrahedra in a 4-space, J. Sci. & Engg. Res. 3 (1959), 169–174.Google Scholar
[10]Mandan, S. R., Desargues' theorem in n-space, J. Australian Math. Soc. 1 (1960), 311–318.CrossRefGoogle Scholar
[11]Room, T. G., Some configurations based on five general planes in space of ten dimensions (and the double-ten of planes and lines in space of four dimensions), Proc. Lond. Math. Soc. (2) 28 (1927), 312–346.Google Scholar
[12]Room, T. G., An extension of the theorem of the fifth associated line, Proc. Lond. Math. Soc., 31 (1929), 455–486.Google Scholar
[13]Room, T. G., Cards and Cubes (A study in incidence geometry), Sydney Uni. Sci. Jour. 17 (1938) 41–46.Google Scholar
[14]Room, T. G., The orthocentre, perspective triangles, and the double-six, Australian Math. Teacher 3 (1947), 42–47.Google Scholar
[15]Whitehead, A. N., A treatise on universal algebra 1 (Cambridge, 1898).Google Scholar