Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-25T06:08:49.914Z Has data issue: false hasContentIssue false
  • English
  • Français

Path properties of the primitives of a Brownian motion

Part of: Stochastic processes Markov processes Limit theorems

Published online by Cambridge University Press:  09 April 2009

Zhengyan Lin
Zhengyan Lin
Affiliation:
Department of Mathematics Zhejiang University, Xixi Campus Hangzhou Zhejiang 310028 P. R.China e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

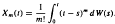Let {W(t), t ≥ 0} be a standard Brownian motion. For a positive integer m, define a Gaussian process Watanabe and Lachal gave some asymptotic properties of the process Xm(·), m ≥ 1. In this paper, we study the bounds of its moduli of continuity and large increments by establishing large deviation results.

Keywords

moduli of continuitylarge incrementsBrownian otionprimitive

MSC classification

Secondary: 60F15: Strong theorems 60J65: Brownian motion 60G17: Sample path properties
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 70 , Issue 1 , February 2001 , pp. 119 - 133
Copyright
Copyright © Australian Mathematical Society 2001

References

[1]Csörgő, M. and Révész, P., Strong approximations in probability and statistics (Academic Press, New York, 1981).Google Scholar
[2]Lachal, A., ‘Local asymptotic classes for the successive primitives of Brownian motion’, Ann. Probab. 25 (1997), 1712–1734.CrossRefGoogle Scholar
[3]Lachal, A., ‘Regular points for the successive primitives of Brownian motion’, J. Math. Kyoto Univ. 37 (1997), 99–119.Google Scholar
[4]Shepp, L. A., ‘Radon-Nikodym derivatives of Gaussian measures’, Ann. Math. Statist. 37 (1966), 321–354.CrossRefGoogle Scholar
[5]Wahba, G., ‘Improper priors, spline smoothing and the problem of guarding against model error in regression’, J. Roy. Statist. Soc. Ser. B 40 (1978), 364–372.Google Scholar
[6]Wahba, G., ‘Bayesian ‘confidence intervals' for the cross-validated smoothing spline’, J. Roy. Statist. Soc. Ser B 45 (1983), 133–150.Google Scholar
[7]Watanabe, H., ‘An asymptotic property of Gaussian processes I’, Trans. Amer. Math. Soc. 148 (1970), 233–248.CrossRefGoogle Scholar