Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-23T15:32:51.151Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Lebesgue function for lagrange interpolation with equidistant nodes

Part of: Approximations and expansions Numerical approximation and computational geometry

Published online by Cambridge University Press:  09 April 2009

T. M. Mills  and
Simon J. Smith
T. M. Mills
Affiliation:
LaTrobe University College of Northern VictoriaP.O. Box 199 Bendigo, Victoria 3550, Australia
Simon J. Smith
Affiliation:
LaTrobe University College of Northern VictoriaP.O. Box 199 Bendigo, Victoria 3550, Australia
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Properties of the Lebesgue function associated with interpolation at the equidistant nodes 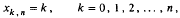, are investigated. In particular, it is proved that the relative maxima of the Lebesgue function are strictly decreasing from the outside towards the middle of the interval [0, n], and upper and lower bounds, and an asymptotic expansion, are obtained for the smallest maximum when n is odd.

Keywords

Lagrange interpolationpolynomialsequidistant nodesLebesgue functionasymptotic expansion

MSC classification

Secondary: 41A05: Interpolation 41A10: Approximation by polynomials 41A44: Best constants 41A60: Asymptotic approximations, asymptotic expansions (steepest descent, etc.) 65D05: Interpolation
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 52 , Issue 1 , February 1992 , pp. 111 - 118
Copyright
Copyright © Australian Mathematical Society 1992

References

[1]Bernstein, Serge, ‘Quelques remarques sur l'interpolation’, Math. Ann. 79 (1918), 1–12.CrossRefGoogle Scholar
[2]Braess, Dietrich, Nonlinear approximation theory (Springer-Verlag, Berlin, 1986).CrossRefGoogle Scholar
[3]Brutman, L., ‘On the Lebesgue function for polynomial interpolation’, SIAM J. Numer. Anal. 15 (1978), 694–704.CrossRefGoogle Scholar
[4]Gradshteyn, I. S. and Ryzhik, I. M., Table of integrals, series, and products Corrected and enlarged edition (Academic Press, New York, 1980).Google Scholar
[5]Luke, Yudell L., The special functions and their approximations, Volume 1 (Academic Press, New York, 1969).Google Scholar
[6]Runck, Paul Otto, ‘Über konvergenzfragen bei polynominterpolation mit äquidistanten knoten I’, J. Reine Angew. Math. 208 (1961), 51–69.CrossRefGoogle Scholar
[7]Runck, Paul Otto, ‘Über konvergenzfragen bei polynominterpolation mit äquidistanten knoten II’, J. Reine Angew. Math. 210 (1962), 175–204.Google Scholar
[8]Runge, C., ‘Über empirische funktionen und die interpolation zwische äquidistanten ordinaten’, Zeitschrift für Mathematik und Physik 46 (1901), 224–243.Google Scholar
[9]Schönhage, A., ‘Fehlerfortpflanzung bei interpolation’, Numerische Mathematik 3 (1961), 62–71.CrossRefGoogle Scholar
[10]Szegö, Gabor, Orthogonal polynomials, 4th edition (Colloq. Pub. 23, Amer. Math. Soc., Providence, 1975).Google Scholar
[11]Trefethen, L. N. and Weideman, J. A. C., ‘Two results on polynomial interpolation in equally spaced points’, J. Approx. Th., to appear.Google Scholar