Hostname: page-component-669899f699-g7b4s Total loading time: 0 Render date: 2025-04-26T01:37:12.890Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE GEOMETRY OF SPACELIKE MEAN CURVATURE FLOW SOLITONS IMMERSED IN A GRW SPACETIME

Part of: Global differential geometry

Published online by Cambridge University Press:  15 September 2023

HENRIQUE F. DE LIMA Open the ORCID record for HENRIQUE F. DE LIMA [Opens in a new window] ,
WALLACE F. GOMES ,
MÁRCIO S. SANTOS  and
MARCO ANTONIO L. VELÁSQUEZ Open the ORCID record for MARCO ANTONIO L. VELÁSQUEZ [Opens in a new window]
HENRIQUE F. DE LIMA*
Affiliation:
Departamento de MatemÁtica, Universidade Federal de Campina Grande, 58.429-970 Campina Grande, Paraíba, Brazil e-mail: [email protected], [email protected]
WALLACE F. GOMES
Affiliation:
Departamento de MatemÁtica, Universidade Federal de Campina Grande, 58.429-970 Campina Grande, Paraíba, Brazil e-mail: [email protected], [email protected]
MÁRCIO S. SANTOS
Affiliation:
Departamento de MatemÁtica, Universidade Federal da Paraíba, 58.051-900 João Pessoa, Paraíba, Brazil e-mail: [email protected]
MARCO ANTONIO L. VELÁSQUEZ
Affiliation:
Departamento de MatemÁtica, Universidade Federal de Campina Grande, 58.429-970 Campina Grande, Paraíba, Brazil e-mail: [email protected], [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate geometric aspects of complete spacelike mean curvature flow solitons of codimension one in a generalized Robertson–Walker (GRW) spacetime 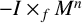$-I\times _{f}M^n$, with base $I\subset \mathbb R$, Riemannian fiber $M^n$ and warping function 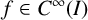$f\in C^\infty (I)$. For this, we apply suitable maximum principles to guarantee that such a mean curvature flow soliton is a slice of the ambient space and to obtain nonexistence results concerning these solitons. In particular, we deal with entire graphs constructed over the Riemannian fiber 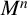$M^n$, which are spacelike mean curvature flow solitons, and we also explore the geometry of a conformal vector field to establish topological and further rigidity results for compact (without boundary) mean curvature flow solitons in a GRW spacetime. Moreover, we study the stability of spacelike mean curvature flow solitons with respect to an appropriate stability operator. Standard examples of spacelike mean curvature flow solitons in GRW spacetimes are exhibited, and applications related to these examples are given.

Keywords

GRW spacetimesspacelike mean curvature flow solitonsspacelike mean curvature flow soliton equationstable spacelike mean curvature flow solitons

MSC classification

Primary: 53C42: Immersions (minimal, prescribed curvature, tight, etc.) 53C50: Lorentz manifolds, manifolds with indefinite metrics
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 116 , Issue 2 , April 2024 , pp. 221 - 256
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

The first, third and fourth authors are partially supported by CNPq, Brazil, grants 301970/2019-0, 306524/2022-8 and 304891/2021-5, respectively. The second author is partially supported by CAPES, Brazil. The third author is also partially supported by FAPESQ-PB, Brazil, grant 3025/2021.

Communicated by J. McCoy

References

Albujer, A. L., ‘New examples of entire maximal graphs in ${\mathbb{H}}^2\times {\mathbb{R}}_1$ ’, Differential Geom. Appl. 26 (2008), 456–462.CrossRefGoogle Scholar
Albujer, A. L. and Alías, L. J., ‘Spacelike hypersurfaces with constant mean curvature in the steady state space’, Proc. Amer. Math. Soc. 137 (2009), 711–721.CrossRefGoogle Scholar
Albujer, A. L., de Lima, H. F., Oliveira, A. M. and VelÁsquez, M. A. L., ‘Rigidity of complete spacelike hypersurfaces in spatially weighted generalized Robertson–Walker spacetimes’, Differential Geom. Appl. 50 (2017), 140–154.CrossRefGoogle Scholar
Albujer, A. L., de Lima, H. F., Oliveira, A. M. and VelÁsquez, M. A. L., ‘ $\psi$ -Parabolicity and the uniqueness of spacelike hypersurfaces immersed in a spatially weighted GRW spacetime’, Mediterr. J. Math. 15 (2018), Article no. 84.CrossRefGoogle Scholar
Aledo, J. A., Alías, L. J. and Romero, A., ‘Integral formulas for compact space-like hypersurfaces in de Sitter space: applications to the case of constant higher order mean curvature’, J. Geom. Phys. 31 (1999), 195–208.CrossRefGoogle Scholar
Alías, L. J., Brasil, A. and Colares, A. G., ‘Integral formulae for spacelike hypersurfaces in conformally stationary spacetimes and applications’, Proc. Edinb. Math. Soc. (2) 46 (2003), 465–488.CrossRefGoogle Scholar
Alías, L. J. and Colares, A. G., ‘Uniqueness of spacelike hypersurfaces with constant higher order mean curvature in generalized Robertson–Walker spacetimes’, Math. Proc. Cambridge Philos. Soc. 143 (2007), 703–729.CrossRefGoogle Scholar
Alías, L. J., Colares, A. G. and de Lima, H. F., ‘On the rigidity of complete spacelike hypersurfaces immersed in a generalized Robertson–Walker spacetime’, Bull. Braz. Math. Soc. (N.S.) 44 (2013), 195–217.CrossRefGoogle Scholar
Alías, L. J., de Lira, J. H. and Rigoli, M., ‘Mean curvature flow solitons in the presence of conformal vector fields’, J. Geom. Anal. 30 (2020), 1466–1529.CrossRefGoogle Scholar
Alías, L. J., de Lira, J. H. and Rigoli, M., ‘Stability of mean curvature flow solitons in warped product spaces’, Rev. Mat. Complut. 35 (2022), 287–309.CrossRefGoogle Scholar
Alías, L. J., Romero, A. and SÁnchez, M., ‘Uniqueness of complete spacelike hypersurfaces of constant mean curvature in generalized Robertson–Walker spacetimes’, Gen. Relativity Gravitation 27 (1995), 71–84.CrossRefGoogle Scholar
Bakry, D. and Émery, M., ‘Diffusions hypercontractives’, in: Séminaire de probabilités, XIX, 1983/84, Lecture Notes in Mathematics, 1123 (eds. Azéma, J. and Yor, M.) (Springer, Berlin, 1985), 177–206.CrossRefGoogle Scholar
Barbosa, J. L. M. and do Carmo, M., ‘Stability of hypersurfaces with constant mean curvature’, Math. Z. 185 (1984), 339–353.CrossRefGoogle Scholar
Barros, A., Brasil, A. and Caminha, A., ‘Stability of spacelike hypersurfaces in foliated spacetimes’, Differential Geom. Appl. 26 (2008), 357–365.CrossRefGoogle Scholar
Batista, M. and de Lima, H. F., ‘Spacelike translating solitons in Lorentzian product spaces: nonexistence, Calabi–Bernstein type results and examples’, Commun. Contemp. Math. 24 (2022), 2150034 (20 pages).CrossRefGoogle Scholar
Bochner, S., ‘Vector fields and Ricci curvature’, Bull. Amer. Math. Soc. (N.S.) 52 (1946), 776–797.CrossRefGoogle Scholar
Bondi, H. and Gold, T., ‘On the generation of magnetism by fluid motion’, Monthly Not. Roy. Astr. Soc. 108 (1948), 252–270.CrossRefGoogle Scholar
Caminha, A., ‘The geometry of closed conformal vector fields on Riemannian spaces’, Bull. Braz. Math. Soc. (N.S.) 42 (2011), 277–300.CrossRefGoogle Scholar
Caminha, A. and de Lima, H. F., ‘Complete vertical graphs with constant mean curvature in semi-Riemannian warped products’, Bull. Belg. Math. Soc. Simon Stevin 16 (2009), 91–105.CrossRefGoogle Scholar
Case, J., Shu, Y. J. and Wei, G., ‘Rigidity of quasi-Einstein metrics’, Differential Geom. Appl. 29 (2011), 93–100.CrossRefGoogle Scholar
Castro, K. and Rosales, C., ‘Free boundary stable hypersurfaces in manifolds with density and rigidity results’, J. Geom. Phys. 79 (2014), 14–28.CrossRefGoogle Scholar
Chen, Q. and Qiu, H., ‘Rigidity of self-shrinkers and translating solitons of mean curvature flows’, Adv. Math. 294 (2016), 517–531.CrossRefGoogle Scholar
Choquet-Bruhat, Y. and York, J., ‘ The Cauchy problem ’, in: General Relativity and Gravitation (ed. Held, A.) (Plenum Press, New York, 1980).Google Scholar
Colombo, G., Mari, L. and Rigoli, M., ‘Remarks on mean curvature flow solitons in warped products’, Discrete Contin. Dyn. Syst. 13 (2020), 1957–1991.Google Scholar
de Lima, E. L., de Lima, H. F. and dos Santos, F. R., ‘On the stability and parabolicity of complete $f$ -maximal spacelike hypersurfaces in weighted generalized Robertson–Walker spacetimes’, Bull. Pol. Acad. Sci. Math. 64 (2016), 199–208.CrossRefGoogle Scholar
de Lima, H. F. and Lima, E. A. Jr, ‘Generalized maximum principles and the unicity of complete spacelike hypersurfaces immersed in a Lorentzian product space’, Beitr. Algebra Geom. 55 (2013), 59–75.CrossRefGoogle Scholar
de Lira, J. H. and Martín, F., ‘Translating solitons in Riemannian products’, J. Differential Equations 266 (2019), 7780–7812.CrossRefGoogle Scholar
Gao, S., Li, G. and Wu, C., ‘Translating spacelike graphs by mean curvature flow with prescribed contact angle’, Arch. Math. 103 (2014), 499–508.CrossRefGoogle Scholar
Hawking, S. W. and Ellis, G. F. R., The Large Scale Structure of Space-Time, Cambridge Monographs on Mathematical Physics, 1 (Cambridge University Press, London, 1973).CrossRefGoogle Scholar
Hoyle, F., ‘A new model for the expanding universe’, Monthly Not. Roy. Astr. Soc. 108 (1948), 372–382.CrossRefGoogle Scholar
Lambert, B., ‘The perpendicular Neumann problem for mean curvature flow with a timelike cone boundary condition’, Trans. Amer. Math. Soc. 366 (2014), 3373–3388.CrossRefGoogle Scholar
Lambert, B. and Lotay, J. D., ‘Spacelike mean curvature flow’, J. Geom. Anal. 31 (2021), 1291–1359.CrossRefGoogle Scholar
Lichnerowicz, A., ‘L’intégration des équations de la gravitation relativiste e le problème des n corps’, J. Math. Pures Appl. (9) 23 (1944), 37–63.Google Scholar
Montiel, S., ‘Uniqueness of spacelike hypersurfaces of constant mean curvature in foliated spacetimes’, Math. Ann. 314 (1999), 529–553.CrossRefGoogle Scholar
O’Neill, B., Semi-Riemannian Geometry with Applications to Relativity (Academic Press, London, 1983).Google Scholar
Pan, T. K., ‘Conformal vector fields in compact Riemannian manifolds’, Proc. Amer. Math. Soc. 14 (1963), 653–657.CrossRefGoogle Scholar
Romero, A., Rubio, R. M. and Salamanca, J. J., ‘Uniqueness of complete maximal hypersurfaces in spatially parabolic generalized Robertson–Walker spacetimes’, Classical Quantum Gravity 30 (2013), 1–13.CrossRefGoogle Scholar
Romero, A., Rubio, R. M. and Salamanca, J. J., ‘Parabolicity of spacelike hypersurfaces in generalized Robertson–Walker spacetimes. Applications to uniqueness results’, Int. J. Geom. Methods Mod. Phys. 10 (2013), 1360014.CrossRefGoogle Scholar
Schoen, R. and Yau, S. T., ‘Proof of the positive mass theorem. I’, Comm. Math. Phys. 65 (1979), 45–76.CrossRefGoogle Scholar
Schoen, R. and Yau, S. T., ‘Proof of the positive mass theorem. II’, Comm. Math. Phys. 79 (1981), 231–260.CrossRefGoogle Scholar
Spruck, J. and Xiao, L., ‘Entire downward translating solitons of the mean curvature flow in Minkowski space’, Proc. Amer. Math. Soc. 144 (2016), 3517–3526.CrossRefGoogle Scholar
Suyama, Y. and Tsukamoto, Y., ‘Riemannian manifolds admitting a certain conformal transformation group’, J. Differential Geom. 5 (1971), 415–426.CrossRefGoogle Scholar
Tadano, H., ‘Remark on a lower diameter bound for compact shrinking Ricci solitons’, Differential Geom. Appl. 66 (2019), 231–241.CrossRefGoogle Scholar
Xu, R. and Liu, T., ‘Rigidity of complete spacelike translating solitons in pseudo-Euclidean space’, J. Math. Anal. Appl. 477 (2019), 692–707.CrossRefGoogle Scholar
Yau, S. T., ‘Some function-theoretic properties of complete Riemannian manifolds and their applications to geometry’, Indiana Univ. Math. J. 25 (1976), 659–670.CrossRefGoogle Scholar