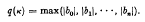Published online by Cambridge University Press: 09 April 2009
In his Topics in Number Theory, vol. 2, chapter 2 (Reading, Mass., 1956) W. J. LeVeque proved an important generalisation of Roth's theorem (K. F. Roth, Mathematika 2,1955, 1—20).
Let ξ be a fixed algebraic number, σ a positive constant, and K an algebraic number field of degree n. For κ∈K denote by κ(1), …, κ(n) the conjugates of κ relative to K, by h(κ) the smallest positive integer such that the polynomial has rational integral coefficients, and by q(κ) the quantity
has rational integral coefficients, and by q(κ) the quantity