Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-24T19:18:28.524Z Has data issue: false hasContentIssue false
  • English
  • Français

On a class of inequalities

Published online by Cambridge University Press:  09 April 2009

B. C. Rennie
B. C. Rennie
Affiliation:
R.A.A.F. Academy, Point Cook, Victor.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

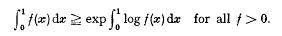First consider some familiar results, the inequality of the arithmetic and geometric mean is: Kantorovich's inequality (reference [1]) asserts that if 0 < A ≦f(x) ≦ B then: The Cauchy-Schwarz inequality is: This paper discusses a certain class of inequalities which includes the three above. Three theorems are proved which apply to any inequality of this class; then follow some examples. They are mainly to show how the general theory helps in the finding of inequalities, but the result of Example 1 seems worth reporting for its own sake.

Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 3 , Issue 4 , November 1963 , pp. 442 - 448
Copyright
Copyright © Australian Mathematical Society 1963

References

[1]Henrici, Peter, Two remarks on the Kantorovich inequality, Amer. Math. Monthly, 68 (1961), 904–906.CrossRefGoogle Scholar
[2]Bonnesen, T. and Fenchel, W., Theorie der Konvexen Körper, Ergebnisse der Mathematik und ihrer Grenzgebiete, III, 1, Berlin (1934).Google Scholar
[3]Cargo, G. T. and Shisha, O., Bounds on Ratios of Means, J. of Research, National Bureau of Standards, 66B (1962), 169–170.Google Scholar
[4]Hardy, G. H., Littlewood, J. E. and Polya, G., Inequalities, Cambridge Univ. Press (1952).Google Scholar