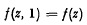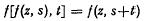Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ran, A.
1971.
Embedding theorems for two-parameter groups of formal power series and related problems.
Israel Journal of Mathematics,
Vol. 9,
Issue. 1,
p.
73.
Bogatyi, Semeon
1997.
On the nonexistence of iterative roots.
Topology and its Applications,
Vol. 76,
Issue. 2,
p.
97.