Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
BHARUCHA-REID, A.T.
and
SAMBANDHAM, M.
1986.
Random Polynomials.
p.
49.
Li, Hung C.
1988.
The exact proability that the roots of quadratic, cubic, and quartic equations are all real if the equation coefficients are random.
Communications in Statistics - Theory and Methods,
Vol. 17,
Issue. 2,
p.
395.
Pratihari, D.
Panda, R. K.
and
Pattanaik, B. P.
1993.
On the number of real roots of a random algebraic equation.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 54,
Issue. 1,
p.
86.
2001.
Mathematics of Chance.
p.
223.


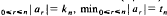 and ε
and ε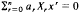 then for
then for 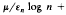
 provided lim
provided lim