Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ponnusamy, Saminathan
Sharma, Navneet Lal
and
Wirths, Karl-Joachim
2018.
Logarithmic Coefficients of the Inverse of Univalent Functions.
Results in Mathematics,
Vol. 73,
Issue. 4,
Maleki, Zahra
Shams, Saeid
Ebadian, Ali
and
Analouei Adegani, Ebrahim
2020.
On Some Problems of Strongly Ozaki Close-to-Convex Functions.
Journal of Function Spaces,
Vol. 2020,
Issue. ,
p.
1.
Alimohammadi, Davood
Cho, Nak Eun
Adegani, Ebrahim Analouei
and
Motamednezhad, Ahmad
2020.
Argument and Coefficient Estimates for Certain Analytic Functions.
Mathematics,
Vol. 8,
Issue. 1,
p.
88.
Alimohammadi, Davood
Analouei Adegani, Ebrahim
Bulboacă, Teodor
Cho, Nak Eun
and
Akeroyd, John R.
2021.
Logarithmic Coefficient Bounds and Coefficient Conjectures for Classes Associated with Convex Functions.
Journal of Function Spaces,
Vol. 2021,
Issue. ,
p.
1.
Alimohammadi, Davood
Analouei Adegani, Ebrahim
Bulboacă, Teodor
and
Cho, Nak Eun
2021.
Logarithmic Coefficients for Classes Related to Convex Functions.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 44,
Issue. 4,
p.
2659.
Zaprawa, Paweł
and
Tra̧bka-Wiȩcław, Katarzyna
2022.
Upper Bounds of the Third Hankel Determinant for Close-to-Convex Functions.
Symmetry,
Vol. 14,
Issue. 5,
p.
885.
Srivastava, H. M.
Prajapati, A.
and
Gochhayat, P.
2022.
Integral means and Yamashita’s conjecture associated with the Janowski type (j, k)-symmetric starlike functions.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 116,
Issue. 4,
Arora, Vibhuti
2022.
Initial Successive Coefficients for Certain Classes of Univalent Functions.
Lobachevskii Journal of Mathematics,
Vol. 43,
Issue. 8,
p.
2080.
Tang, Huo
Ahmad, Adeel
Rasheed, Akhter
Ali, Asad
Hussain, Saqib
and
Noor, Saima
2023.
Sharp Coefficient and Hankel Problems Related to a Symmetric Domain.
Symmetry,
Vol. 15,
Issue. 10,
p.
1865.
Mundalia, Mridula
and
Kumar, S. Sivaprasad
2023.
On a subfamily of starlike functions related to hyperbolic cosine function.
The Journal of Analysis,
Vol. 31,
Issue. 3,
p.
2043.
Ali, Md Firoz
and
Pal, Sanjit
2023.
Pre-Schwarzian norm estimates for the class of janowski starlike functions.
Monatshefte für Mathematik,
Vol. 201,
Issue. 2,
p.
311.
Allu, Vasudevarao
Arora, Vibhuti
and
Shaji, Amal
2023.
On the Second Hankel Determinant of Logarithmic Coefficients for Certain Univalent Functions.
Mediterranean Journal of Mathematics,
Vol. 20,
Issue. 2,
Analouei Adegani, Ebrahim
Motamednezhad, Ahmad
Jafari, Mostafa
and
Bulboacă, Teodor
2023.
Logarithmic Coefficients Inequality for the Family of Functions Convex in One Direction.
Mathematics,
Vol. 11,
Issue. 9,
p.
2140.
Analouei Adegani, Ebrahim
Bulboacă, Teodor
Hameed Mohammed, Nafya
and
Zaprawa, Paweł
2023.
Solution of logarithmic coefficients conjectures for some classes of convex functions.
Mathematica Slovaca,
Vol. 73,
Issue. 1,
p.
79.
Adegani, Ebrahim Analouei
Alimohammadi, Davood
Bulboacă, Teodor
Cho, Nak Eun
and
Bidkham, Mahmood
2023.
On the logarithmic coefficients for some classes defined by subordination.
AIMS Mathematics,
Vol. 8,
Issue. 9,
p.
21732.
Guo, Dong
Tang, Huo
Zhang, Jun
Li, Zongtao
Xu, Qingbing
and
Ao, En
2023.
Improved Upper Bounds of the Third-Order Hankel Determinant for Ozaki Close-to-Convex Functions.
Symmetry,
Vol. 15,
Issue. 6,
p.
1176.
Allu, Vasudevarao
and
Sharma, Navneet Lal
2024.
On logarithmic coefficients for classes of analytic functions associated with convex functions.
Bulletin des Sciences Mathématiques,
Vol. 191,
Issue. ,
p.
103384.
Şeker, Bilal
Çekiç, Bilal
Sümer, Sevtap
and
Akçiçek, Onur
2024.
The second Hankel determinant of logarithmic coefficients and logarithmic inverse coefficients for the class of bounded turning functions of order α.
Lithuanian Mathematical Journal,
Vol. 64,
Issue. 4,
p.
530.
Guo, Dong
Tang, Huo
Zhang, Jun
Xu, Qingbing
Li, Zongtao
and
Stanimirović, Predrag S.
2024.
Hankel Determinants for the Logarithmic Coefficients of a Subclass of Close-to-Star Functions.
Journal of Mathematics,
Vol. 2024,
Issue. ,
p.
1.
Sharma, Navneet Lal
and
Bulboacă, Teodor
2024.
Logarithmic coefficient bounds for the class of Bazilevič functions.
Analysis and Mathematical Physics,
Vol. 14,
Issue. 3,
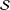 ${\mathcal{S}}$ be the family of analytic and univalent functions
${\mathcal{S}}$ be the family of analytic and univalent functions 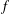 $f$ in the unit disk
$f$ in the unit disk  $\mathbb{D}$ with the normalization
$\mathbb{D}$ with the normalization 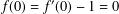 $f(0)=f^{\prime }(0)-1=0$, and let
$f(0)=f^{\prime }(0)-1=0$, and let 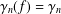 $\unicode[STIX]{x1D6FE}_{n}(f)=\unicode[STIX]{x1D6FE}_{n}$ denote the logarithmic coefficients of
$\unicode[STIX]{x1D6FE}_{n}(f)=\unicode[STIX]{x1D6FE}_{n}$ denote the logarithmic coefficients of 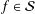 $f\in {\mathcal{S}}$. In this paper we study bounds for the logarithmic coefficients for certain subfamilies of univalent functions. Also, we consider the families
$f\in {\mathcal{S}}$. In this paper we study bounds for the logarithmic coefficients for certain subfamilies of univalent functions. Also, we consider the families  ${\mathcal{F}}(c)$ and
${\mathcal{F}}(c)$ and 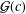 ${\mathcal{G}}(c)$ of functions
${\mathcal{G}}(c)$ of functions 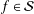 $f\in {\mathcal{S}}$ defined by
$f\in {\mathcal{S}}$ defined by 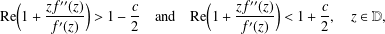 $$\begin{eqnarray}\text{Re}\biggl(1+{\displaystyle \frac{zf^{\prime \prime }(z)}{f^{\prime }(z)}}\biggr)>1-{\displaystyle \frac{c}{2}}\quad \text{and}\quad \text{Re}\biggl(1+{\displaystyle \frac{zf^{\prime \prime }(z)}{f^{\prime }(z)}}\biggr)<1+{\displaystyle \frac{c}{2}},\quad z\in \mathbb{D},\end{eqnarray}$$
$$\begin{eqnarray}\text{Re}\biggl(1+{\displaystyle \frac{zf^{\prime \prime }(z)}{f^{\prime }(z)}}\biggr)>1-{\displaystyle \frac{c}{2}}\quad \text{and}\quad \text{Re}\biggl(1+{\displaystyle \frac{zf^{\prime \prime }(z)}{f^{\prime }(z)}}\biggr)<1+{\displaystyle \frac{c}{2}},\quad z\in \mathbb{D},\end{eqnarray}$$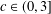 $c\in (0,3]$ and
$c\in (0,3]$ and 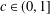 $c\in (0,1]$, respectively. We obtain the sharp upper bound for
$c\in (0,1]$, respectively. We obtain the sharp upper bound for 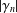 $|\unicode[STIX]{x1D6FE}_{n}|$ when
$|\unicode[STIX]{x1D6FE}_{n}|$ when 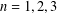 $n=1,2,3$ and
$n=1,2,3$ and 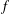 $f$ belongs to the classes
$f$ belongs to the classes 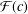 ${\mathcal{F}}(c)$ and
${\mathcal{F}}(c)$ and 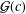 ${\mathcal{G}}(c)$, respectively. The paper concludes with the following two conjectures:
${\mathcal{G}}(c)$, respectively. The paper concludes with the following two conjectures: $f\in {\mathcal{F}}(-1/2)$, then
$f\in {\mathcal{F}}(-1/2)$, then 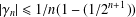 $|\unicode[STIX]{x1D6FE}_{n}|\leq 1/n(1-(1/2^{n+1}))$ for
$|\unicode[STIX]{x1D6FE}_{n}|\leq 1/n(1-(1/2^{n+1}))$ for 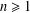 $n\geq 1$, and
$n\geq 1$, and 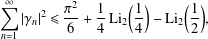 $$\begin{eqnarray}\mathop{\sum }_{n=1}^{\infty }|\unicode[STIX]{x1D6FE}_{n}|^{2}\leq {\displaystyle \frac{\unicode[STIX]{x1D70B}^{2}}{6}}+{\displaystyle \frac{1}{4}}~\text{Li}_{2}\biggl({\displaystyle \frac{1}{4}}\biggr)-\text{Li}_{2}\biggl({\displaystyle \frac{1}{2}}\biggr),\end{eqnarray}$$
$$\begin{eqnarray}\mathop{\sum }_{n=1}^{\infty }|\unicode[STIX]{x1D6FE}_{n}|^{2}\leq {\displaystyle \frac{\unicode[STIX]{x1D70B}^{2}}{6}}+{\displaystyle \frac{1}{4}}~\text{Li}_{2}\biggl({\displaystyle \frac{1}{4}}\biggr)-\text{Li}_{2}\biggl({\displaystyle \frac{1}{2}}\biggr),\end{eqnarray}$$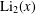 $\text{Li}_{2}(x)$ denotes the dilogarithm function.
$\text{Li}_{2}(x)$ denotes the dilogarithm function.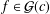 $f\in {\mathcal{G}}(c)$, then
$f\in {\mathcal{G}}(c)$, then 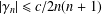 $|\unicode[STIX]{x1D6FE}_{n}|\leq c/2n(n+1)$ for
$|\unicode[STIX]{x1D6FE}_{n}|\leq c/2n(n+1)$ for 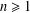 $n\geq 1$.
$n\geq 1$.